Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-27T10:50:26.918Z Has data issue: false hasContentIssue false
  • English
  • Français

HEURISTICS FOR 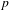$p$-CLASS TOWERS OF REAL QUADRATIC FIELDS

Part of: Algebraic number theory: global fields

Published online by Cambridge University Press:  26 December 2019

Nigel Boston ,
Michael R. Bush  and
Farshid Hajir
Nigel Boston
Affiliation:
Department of Mathematics, University of Wisconsin – Madison, 480 Lincoln Drive, Madison, WI 53706, USA ([email protected])
Michael R. Bush
Affiliation:
Department of Mathematics, Washington and Lee University, 204 W. Washington Street, Lexington, VA 24450, USA ([email protected])
Farshid Hajir
Affiliation:
Department of Mathematics & Statistics, University of Massachusetts – Amherst, 710 N. Pleasant Street, Amherst, MA 01003, USA ([email protected])
Get access Rights & Permissions [Opens in a new window]

Abstract

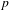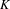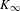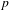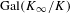Let $p$ be an odd prime. For a number field $K$, we let $K_{\infty }$ be the maximal unramified pro-$p$ extension of $K$; we call the group $\text{Gal}(K_{\infty }/K)$ the 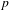$p$-class tower group of 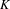$K$. In a previous work, as a non-abelian generalization of the work of Cohen and Lenstra on ideal class groups, we studied how likely it is that a given finite $p$-group occurs as the $p$-class tower group of an imaginary quadratic field. Here we do the same for an arbitrary real quadratic field 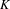$K$ as base. As before, the action of 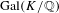$\text{Gal}(K/\mathbb{Q})$ on the 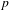$p$-class tower group of 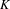$K$ plays a crucial role; however, the presence of units of infinite order in the ground field significantly complicates the possibilities for the groups that can occur. We also sharpen our results in the imaginary quadratic field case by removing a certain hypothesis, using ideas of Boston and Wood. In the appendix, we show how the probabilities introduced for finite 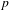$p$-groups can be extended in a consistent way to the infinite pro-$p$ groups which can arise in both the real and imaginary quadratic settings.

Keywords

Cohen–Lenstra heuristicsclass field towerreal quadratic fieldideal class groupSchur 𝜎-groupSchur + 1 𝜎-group

MSC classification

Primary: 11R29: Class numbers, class groups, discriminants 11R11: Quadratic extensions
Secondary: 11R32: Galois theory
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 20 , Issue 4 , July 2021 , pp. 1429 - 1452
Check for updates
Copyright
© The Author(s), 2019. Published by Cambridge University Press

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The research of NB is supported by Simons grant MSN179747. The work of MRB was partially supported by summer Lenfest Grants from Washington and Lee University.

References

Bosma, W., Cannon, J. J. and Playoust, C., The Magma algebra system. I. The user language, J. Symbolic Comput. 24 (1997), 235–265.CrossRefGoogle Scholar
Boston, N., Explicit deformation of Galois representations, Invent. Math. 103(1) (1991), 181–196.CrossRefGoogle Scholar
Boston, N., Bush, M. R. and Hajir, F., Heuristics for p-class towers of imaginary quadratic fields, Math. Ann. 368(1–2) (2017), 633–669.CrossRefGoogle Scholar
Boston, N. and Leedham-Green, C. R., Explicit computation of Galois p-groups unramified at p , J. Algebra 256(2) (2002), 402–413.CrossRefGoogle Scholar
Boston, N. and Nover, H., Computing Pro-p Galois Groups, Lecture Notes in Computer Science, volume 4076, ANTS VII, pp. 1–10 (Springer, Berlin, 2006).Google Scholar
Boston, N. and Wood, M. M., Nonabelian Cohen–Lenstra heuristics over function fields, Compositio Math. 153(7) (2017), 1372–1390.CrossRefGoogle Scholar
Cohen, H. and Lenstra, H. W. Jr., Heuristics on class groups of number fields, in Number Theory, Noordwijkerhout 1983, LNM, volume 1068, pp. 33–62 (Springer, Berlin, 1984).CrossRefGoogle Scholar
Gorenstein, D., Finite Groups, AMS Chelsea Publishing Series (American Mathematical Society, New York, 2007).Google Scholar
Kisilevsky, H. and Labute, J., On a sufficient condition for the p-class tower of a CM-field to be infinite, in ThĂ©orie des nombres (Quebec, PQ, 1987), pp. 556–560 (de Gruyter, Berlin, 1989).Google Scholar
Koch, H., Galois theory of $p$ -extensions. With a foreword by I. R. Shafarevic. Translated from the 1970 German original by Franz Lemmermeyer. With a postscript by the author and Lemmermeyer. Springer Monographs in Mathematics. Springer, Berlin, 2002.Google Scholar
Koch, H. and Venkov, B. B., Über den p-Klassenkörperturm eines imaginĂ€r-quadratischen Zahlkörpers, Soc. Math. France, AstĂ©risque 24–25 (1975), 57–67.Google Scholar
Neukirch, J., Wingberg, K. and Schmidt, A., Cohomology of Number Fields, 2nd edn, Grundlehren der Mathematischen Wissenschaften [Fundamental Principles of Mathematical Sciences], volume 323 (Springer, Berlin, 2008), xvi + 825 pp.CrossRefGoogle Scholar
O’Brien, E. A., The p-group generation algorithm, J. Symbolic Comput. 9 (1990), 677–698.CrossRefGoogle Scholar
The PARI Group, PARI/GP version 2.7.0, Bordeaux, 2014, http://pari.math.u-bordeaux.fr/.Google Scholar
Schoof, R., Infinite class field towers of quadratic fields, J. Reine Angew. Math. 372 (1986), 209–220.Google Scholar
Shafarevich, I. R., Extensions with prescribed ramification points, Publ. Math. Inst. Hautes Études Sci. 18 (1963), 71–95 (Russian). English Translation in I. R. Shafarevich, Collected Mathematical Papers, Springer, Berlin, 1989.Google Scholar