Hostname: page-component-586b7cd67f-vdxz6 Total loading time: 0 Render date: 2024-11-23T00:03:13.337Z Has data issue: false hasContentIssue false
  • English
  • Français

DENSITY RESULTS FOR SPECIALIZATION SETS OF GALOIS COVERS

Part of: Arithmetic algebraic geometry Birational geometry Algebraic number theory: global fields Arithmetic problems. Diophantine geometry

Published online by Cambridge University Press:  25 October 2019

Joachim König Open the ORCID record for Joachim König [Opens in a new window]  and
François Legrand
Joachim König
Affiliation:
Department of Mathematical Sciences, KAIST, 291 Daehak-ro Yuseong-gu Daejeon34141, South Korea ([email protected])
François Legrand
Affiliation:
Institut für Algebra, Fachrichtung Mathematik, TU Dresden, 01062Dresden, Germany ([email protected])
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We provide evidence for this conclusion: given a finite Galois cover 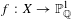$f:X\rightarrow \mathbb{P}_{\mathbb{Q}}^{1}$ of group 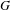$G$, almost all (in a density sense) realizations of 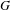$G$ over 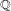$\mathbb{Q}$ do not occur as specializations of 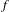$f$. We show that this holds if the number of branch points of 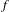$f$ is sufficiently large, under the abc-conjecture and, possibly, the lower bound predicted by the Malle conjecture for the number of Galois extensions of 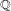$\mathbb{Q}$ of given group and bounded discriminant. This widely extends a result of Granville on the lack of 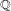$\mathbb{Q}$-rational points on quadratic twists of hyperelliptic curves over 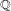$\mathbb{Q}$ with large genus, under the abc-conjecture (a diophantine reformulation of the case 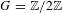$G=\mathbb{Z}/2\mathbb{Z}$ of our result). As a further evidence, we exhibit a few finite groups 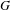$G$ for which the above conclusion holds unconditionally for almost all covers of 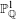$\mathbb{P}_{\mathbb{Q}}^{1}$ of group 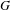$G$. We also introduce a local–global principle for specializations of Galois covers 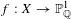$f:X\rightarrow \mathbb{P}_{\mathbb{Q}}^{1}$ and show that it often fails if 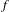$f$ has abelian Galois group and sufficiently many branch points, under the abc-conjecture. On the one hand, such a local–global conclusion underscores the ‘smallness’ of the specialization set of a Galois cover of 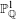$\mathbb{P}_{\mathbb{Q}}^{1}$. On the other hand, it allows to generate conditionally ‘many’ curves over $\mathbb{Q}$ failing the Hasse principle, thus generalizing a recent result of Clark and Watson devoted to the hyperelliptic case.

Keywords

Galois theoryspecializationsthe abc-conjecturethe Malle conjecturethe uniformity conjecturehyperelliptic and superelliptic curvesrational pointstwisted coversthe Hasse principle

MSC classification

Primary: 11R32: Galois theory
Secondary: 11G30: Curves of arbitrary genus or genus $ne 1$ over global fields 14E20: Coverings 14G05: Rational points
Type
Research Article
Information
Journal of the Institute of Mathematics of Jussieu , Volume 20 , Issue 5 , September 2021 , pp. 1455 - 1496
Check for updates
Creative Commons
Creative Common License - CCCreative Common License - BY
This is an Open Access article, distributed under the terms of the Creative Commons Attribution licence (http://creativecommons.org/licenses/by/4.0/), which permits unrestricted re-use, distribution, and reproduction in any medium, provided the original work is properly cited.
Copyright
© The Author(s), 2019. Published by Cambridge University Press

References

Beckmann, S., On extensions of number fields obtained by specializing branched coverings, J. Reine Angew. Math. 419 (1991), 27–53.Google Scholar
Bhargava, M., Mass formulae for extensions of local fields, and conjectures on the density of number field discriminants, Int. Math. Res. Not. IMRN 2007(17) (2007), Art. ID rnm052, 20 pp.CrossRefGoogle Scholar
Bhargava, M. and Wood, M., The density of discriminants of S 3 -sextic number fields, Proc. Amer. Math. Soc. 136(5) (2008), 1581–1587.CrossRefGoogle Scholar
Bourdon, A., Clark, P. and Stankewicz, J., Torsion points on CM elliptic curves over real number fields, Trans. Amer. Math. Soc. 369(12) (2017), 8457–8496.CrossRefGoogle Scholar
Byeon, D., Ranks of quadratic twists of an elliptic curve, Acta Arith. 114(4) (2004), 391–396.CrossRefGoogle Scholar
Byeon, D., Jeon, D. and Kim, C. H., Rank-one quadratic twists of an infinite family of elliptic curves, J. Reine Angew. Math. 633 (2009), 67–76.Google Scholar
Caporaso, L., Harris, J. and Mazur, B. C., Uniformity of rational points, J. Amer. Math. Soc. 10(1) (1997), 1–35.CrossRefGoogle Scholar
Clark, P. and Watson, L., ABC and the Hasse principle for quadratic twists of hyperelliptic curves, C. R. Math. Acad. Sci. Paris 356(9) (2018), 911–915.CrossRefGoogle Scholar
Cohen, S. D., The distribution of Galois groups and Hilbert’s irreducibility theorem, Proc. Lond. Math. Soc. (3) 43(2) (1981), 227–250.CrossRefGoogle Scholar
Dabrowski, A., On the proportion of rank 0 twists of elliptic curves, C. R. Math. Acad. Sci. Paris 346(9–10) (2008), 483–486.CrossRefGoogle Scholar
Dèbes, P., Galois covers with prescribed fibers: the Beckmann–Black problem, Ann. Sc. Norm. Super. Pisa Cl. Sci. (4) 28(2) (1999), 273–286.Google Scholar
Dèbes, P., Arithmétique des revêtements de la droite, Lecture notes, (2009). At http://math.univ-lille1.fr/∼pde/ens.html.Google Scholar
Dèbes, P., On the Malle conjecture and the self-twisted cover, Israel J. Math. 218(1) (2017), 101–131.CrossRefGoogle Scholar
Dèbes, P., Groups with no parametric Galois realizations, Ann. Sci. Éc. Norm. Supér. (4) 51(1) (2018), 143–179.CrossRefGoogle Scholar
Dèbes, P. and Fried, M. D., Rigidity and real residue class fields, Acta Arith. 56(4) (1990), 291–323.Google Scholar
Dèbes, P. and Ghazi, N., Galois covers and the Hilbert–Grunwald property, Ann. Inst. Fourier (Grenoble) 62(3) (2012), 989–1013.CrossRefGoogle Scholar
Dèbes, P., König, J., Legrand, F. and Neftin, D., Rational pullbacks of Galois covers. Manuscript, 2018. arXiv:1807.01937.Google Scholar
Demarche, C., Arteche, G. L. and Neftin, D., The Grunwald problem and approximation properties for homogeneous spaces, Ann. Inst. Fourier (Grenoble) 67(3) (2017), 1009–1033.CrossRefGoogle Scholar
Fried, M. D., Fields of definition of function fields and Hurwitz families-groups as Galois groups, Comm. Algebra 5(1) (1977), 17–82.CrossRefGoogle Scholar
Fried, M. D. and Jarden, M., Field Arithmetic, third edition, Ergebnisse der Mathematik und ihrer Grenzgebiete. 3. Folge. A Series of Modern Surveys in Mathematics [Results in Mathematics and Related Areas. 3rd Series. A Series of Modern Surveys in Mathematics], Volume 11 (Springer-Verlag, Berlin, 2008). Revised by Jarden. xxiv + 792 pp.Google Scholar
Gel’fand, I. M., Kapranov, M. M. and Zelevinsky, A. V., Discriminants, Resultants, and Multidimensional Determinants, Mathematics: Theory & Applications (Birkhäuser Boston, Inc., Boston, MA, 1994). x+523 pp.CrossRefGoogle Scholar
Gouvêa, F. Q. and Mazur, B. C., The square-free sieve and the rank of elliptic curves, J. Amer. Math. Soc. 4(1) (1991), 1–23.CrossRefGoogle Scholar
Granville, A., ABC allows us to count squarefrees, Int. Math. Res. Not. IMRN 19 (1998), 991–1009.CrossRefGoogle Scholar
Granville, A., Rational and integral points on quadratic twists of a given hyperelliptic curve, Int. Math. Res. Not. IMRN 2007(8) (2007), Art. ID 027, 24 pp.Google Scholar
Jensen, C. U., Ledet, A. and Yui, N., Generic polynomials. Constructive Aspects of the Inverse Galois Problem, Mathematical Sciences Research Institute Publications, Volume 45 (Cambridge University Press, 2002). x+258 pp.Google Scholar
König, J. and Legrand, F., Non-parametric sets of regular realizations over number fields, J. Algebra 497 (2018), 302–336.CrossRefGoogle Scholar
König, J., Legrand, F. and Neftin, D., On the local behavior of specializations of function field extensions, Int. Math. Res. Not. IMRN 2019(9) (2019), 2951–2980.CrossRefGoogle Scholar
Klüners, J., Asymptotics of number fields and the Cohen–Lenstra heuristics, J. Théor. Nombres Bordeaux 18(3) (2006), 607–615.CrossRefGoogle Scholar
Klüners, J. and Malle, G., A database for field extensions of the rationals, LMS J. Comput. Math. 4 (2001), 182–196.CrossRefGoogle Scholar
Klüners, J. and Malle, G., Counting nilpotent Galois extensions, J. Reine Angew. Math. 572 (2004), 1–26.CrossRefGoogle Scholar
König, J., Non-parametricity of rational translates of regular Galois extensions, Acta Arith. 179(3) (2017), 267–275.CrossRefGoogle Scholar
Legrand, F., Parametric Galois extensions, J. Algebra 422 (2015), 187–222.CrossRefGoogle Scholar
Legrand, F., Specialization results and ramification conditions, Israel J. Math. 214(2) (2016), 621–650.CrossRefGoogle Scholar
Legrand, F., Twists of superelliptic curves without rational points, Int. Math. Res. Not. IMRN 2018(4) (2018), 1153–1176.Google Scholar
Malle, G., On the distribution of Galois groups, J. Number Theory 92(2) (2002), 315–329.CrossRefGoogle Scholar
Malle, G. and Matzat, B. H., Inverse Galois Theory, second edition, Springer Monographs in Mathematics (Springer, Berlin, 2018). xvii+532 pp.CrossRefGoogle Scholar
Serre, J.-P., Divisibilité de certaines fonctions arithmétiques, Enseign. Math. (2) 22(3–4) (1976), 227–260.Google Scholar
Serre, J.-P., Topics in Galois Theory, Research Notes in Mathematics, Volume 1 (Jones and Bartlett Publishers, Boston, MA, 1992). Lecture notes prepared by Henri Darmon [Henri Darmon]. With a foreword by Darmon and the author. xvi+117 pp.Google Scholar
Stoll, M., Rational points on hyperelliptic curves: recent developments, in Computeralgebra-Rundbrief 54 (Fachgruppe Computeralgebra, Berlin, 2014).Google Scholar
Vatsal, V., Rank-one twists of a certain elliptic curve, Math. Ann. 311(4) (1998), 791–794.CrossRefGoogle Scholar
Völklein, H., Groups as Galois Groups. An Introduction, Cambridge Studies in Advanced Mathematics, Volume 53 (Cambridge University Press, Cambridge, 1996). xviii+248 pp.CrossRefGoogle Scholar
Yu, M., Selmer ranks of twists of hyperelliptic curves and superelliptic curves, J. Number Theory 160 (2016), 148–185.CrossRefGoogle Scholar