Article contents
Differential graded motives: weight complex, weight filtrations and spectral sequences for realizations; Voevodsky versus Hanamura
Published online by Cambridge University Press: 16 October 2008
Abstract
We describe explicitly the Voevodsky's triangulated category of motives 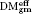 (and give a ‘differential graded enhancement’ of it). This enables us to able to verify that DMgm ℚ is (anti)isomorphic to Hanamura's
(and give a ‘differential graded enhancement’ of it). This enables us to able to verify that DMgm ℚ is (anti)isomorphic to Hanamura's  (k).
(k).
We obtain a description of all subcategories (including those of Tate motives) and of all localizations of 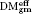 . We construct a conservative weight complex functor
. We construct a conservative weight complex functor  ; t gives an isomorphism
; t gives an isomorphism 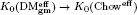 . A motif is mixed Tate whenever its weight complex is. Over finite fields the Beilinson–Parshin conjecture holds if and only if tℚ is an equivalence.
. A motif is mixed Tate whenever its weight complex is. Over finite fields the Beilinson–Parshin conjecture holds if and only if tℚ is an equivalence.
For a realization D of 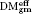 we construct a spectral sequence S (the spectral sequence of motivic descent) converging to the cohomology of an arbitrary motif X. S is ‘motivically functorial’; it gives a canonical functorial weight filtration on the cohomology of D(X). For the ‘standard’ realizations this filtration coincides with the usual one (up to a shift of indices). For the motivic cohomology this weight filtration is non-trivial and appears to be quite new.
we construct a spectral sequence S (the spectral sequence of motivic descent) converging to the cohomology of an arbitrary motif X. S is ‘motivically functorial’; it gives a canonical functorial weight filtration on the cohomology of D(X). For the ‘standard’ realizations this filtration coincides with the usual one (up to a shift of indices). For the motivic cohomology this weight filtration is non-trivial and appears to be quite new.
We define the (rational) length of a motif M; modulo certain ‘standard’ conjectures this length coincides with the maximal length of the weight filtration of the singular cohomology of M.
Keywords
MSC classification
- Type
- Research Article
- Information
- Journal of the Institute of Mathematics of Jussieu , Volume 8 , Issue 1 , January 2009 , pp. 39 - 97
- Copyright
- Copyright © Cambridge University Press 2008
References
- 30
- Cited by


