Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-25T08:55:58.718Z Has data issue: false hasContentIssue false
  • English
  • Français

A SPHERICAL VERSION OF THE KOWALSKI–SŁODKOWSKI THEOREM AND ITS APPLICATIONS

Part of: Commutative Banach algebras and commutative topological algebras Spaces and algebras of analytic functions Normed linear spaces and Banach spaces; Banach lattices

Published online by Cambridge University Press:  03 December 2020

SHIHO OI Open the ORCID record for SHIHO OI [Opens in a new window]
SHIHO OI*
Affiliation:
Niigata Prefectural Hakkai High School, Minamiuonuma949-6681, Japan Current address: Department of Mathematics, Faculty of Science, Niigata University, Niigata 950-2181, Japan e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Li et al. [‘Weak 2-local isometries on uniform algebras and Lipschitz algebras’, Publ. Mat.63 (2019), 241–264] generalized the Kowalski–Słodkowski theorem by establishing the following spherical variant: let A be a unital complex Banach algebra and let 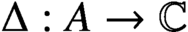$\Delta : A \to \mathbb {C}$ be a mapping satisfying the following properties:

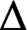  1. (a) $\Delta $ is 1-homogeneous (that is, 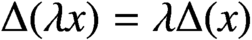$\Delta (\lambda x)=\lambda \Delta (x)$ for all 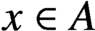$x \in A$ , $\lambda \in \mathbb C$ );

  2. (b) $\Delta (x)-\Delta (y) \in \mathbb {T}\sigma (x-y), \quad x,y \in A$ .

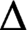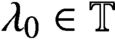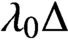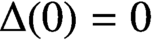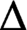Then $\Delta $ is linear and there exists $\lambda _{0} \in \mathbb {T}$ such that $\lambda _{0}\Delta $ is multiplicative. In this note we prove that if (a) is relaxed to $\Delta (0)=0$ , then $\Delta $ is complex-linear or conjugate-linear and 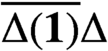$\overline {\Delta (\mathbf {1})}\Delta $ is multiplicative. We extend the Kowalski–Słodkowski theorem as a conclusion. As a corollary, we prove that every 2-local map in the set of all surjective isometries (without assuming linearity) on a certain function space is in fact a surjective isometry. This gives an affirmative answer to a problem on 2-local isometries posed by Molnár [‘On 2-local *-automorphisms and 2-local isometries of B(H)', J. Math. Anal. Appl.479(1) (2019), 569–580] and also in a private communication between Molnár and O. Hatori, 2018.

Keywords

2-local mapssurjective isometriesthe Kowalski–Słodkowski theorem

MSC classification

Primary: 46B04: Isometric theory of Banach spaces
Secondary: 46B20: Geometry and structure of normed linear spaces 46J10: Banach algebras of continuous functions, function algebras 46J15: Banach algebras of differentiable or analytic functions, $H^p$-spaces 30H05: Bounded analytic functions
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 111 , Issue 3 , December 2021 , pp. 386 - 411
Check for updates
Copyright
© 2020 Australian Mathematical Publishing Association Inc.

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Communicated by Lisa Orloff Clark

Dedicated to Professor Osamu Hatori on the occasion of his retirement from Niigata University

References

Al-Halees, H. and Fleming, R., ‘On 2-local isometries on continuous vector valued function spaces’, J. Math. Anal. Appl. 354 (2009), 70–77.10.1016/j.jmaa.2008.12.023CrossRefGoogle Scholar
Batty, C. J. K. and Molnár, L., ‘On topological reflexivity of the groups of $\ast$ -automorphisms and surjective isometries of $B(H)$ ’, Arch. Math. 67 (1996), 415–421.10.1007/BF01189101CrossRefGoogle Scholar
Botelho, F., Jamison, J. and Molnár, L., ‘Algebraic reflexivity of isometry groups and automorphism groups of some operator structures’, J. Math. Anal. Appl. 408 (2013), 177–195.10.1016/j.jmaa.2013.06.001CrossRefGoogle Scholar
Sánchez, F. Cabello, ‘Automorphisms of algebras of smooth functions and equivalent functions’, Differential Geom. Appl. 30 (2012), 216–221.10.1016/j.difgeo.2012.03.003CrossRefGoogle Scholar
Cabello, J. C. and Peralta, A. M., ‘Weak-2-local symmetric maps on ${C}^{\ast }$ -algebras’, Linear Algebra Appl . 494 (2016), 32–43.10.1016/j.laa.2015.12.024CrossRefGoogle Scholar
Gleason, A. M., ‘A characterization of maximal ideals’, J. Anal. Math. 19 (1967), 171–172.10.1007/BF02788714CrossRefGoogle Scholar
Győry, M., ‘2-local isometries of ${C}_0(X)$ ’, Acta Sci. Math. (Szeged) 67 (2001), 735–746.Google Scholar
Hatori, O. and Miura, T., ‘Real linear isometries between function algebras. II’, Cent. Eur. J. Math. 11 (2013), 1838–1842.Google Scholar
Hatori, O., Miura, T., Oka, H. and Takagi, H., ‘2-Local isometries and 2-local automorphisms on uniform algebras’, Int. Math. Forum 50 (2007), 2491–2502.10.12988/imf.2007.07219CrossRefGoogle Scholar
Hatori, O. and Oi, S., ‘2-local isometries on function spaces’, Recent Trends in Operator Theory and Applications, Contemporary Mathematics, 737 (American Mathematical Society, Providence, RI, 2019), 89–106.10.1090/conm/737/14860CrossRefGoogle Scholar
Jiménez-Vargas, A., Campoy, A. M. and Villegas-Vallecillos, M., ‘Algebraic reflexivity of the isometry group of some spaces of Lipschitz functions’, J. Math. Anal. Appl. 366 (2010), 195–201.10.1016/j.jmaa.2010.01.034CrossRefGoogle Scholar
Jiménez-Vargas, A., Li, L., Peralta, A. M., Wang, L. and Wang, Y.-S., ‘2-local standard isometries on vector-valued Lipschitz function spaces’, J. Math. Anal. Appl. 461 (2018), 1287–1298.10.1016/j.jmaa.2018.01.029CrossRefGoogle Scholar
Jimenez-Vargas, A. and Villegas-Vallecillos, M., ‘2-local isometries on spaces of Lipschitz functions’, Canad. Math. Bull. 54 (2011), 680–692.10.4153/CMB-2011-025-5CrossRefGoogle Scholar
Kahane, J. P. and Żelazko, W., ‘A characterization of maximal ideals in commutative Banach algebras’, Studia Math. 29 (1968), 339–343.10.4064/sm-29-3-339-343CrossRefGoogle Scholar
Kawamura, K., Koshimizu, H. and Miura, T., ‘Norms on ${C}^1\left(\left[0,1\right]\right)$ and their isometries’, Acta Sci. Math. (Szeged) 84 (2018), 239–261.10.14232/actasm-017-331-0CrossRefGoogle Scholar
Kowalski, S. and Słodkowski, Z., ‘A characterization of multiplicative linear functionals in Banach algebras’, Studia Math. 67 (1980), 215–223.10.4064/sm-67-3-215-223CrossRefGoogle Scholar
Li, L., Peralta, A. M., Wang, L. and Wang, Y.-S., ‘Weak-2-local isometries on uniform algebras and Lipschitz algebras’, Publ. Mat. 63 (2019), 241–264.10.5565/PUBLMAT6311908CrossRefGoogle Scholar
Mankiewicz, P., ‘On the differentiability of Lipschitz mappings in Fréchet spaces’, Studia Math. 45 (1973), 15–29.10.4064/sm-45-1-15-29CrossRefGoogle Scholar
Miura, T., ‘Surjective isometries on a Banach space of analytic functions on the open unit disc’, Preprint, 2019, arXiv:1901.02737v1.Google Scholar
Miura, T. and Takagi, H., ‘Surjective isometries on the Banach space of continuously differentiable functions’, Contemp. Math. 687 (2017), 181–192.10.1090/conm/687/13787CrossRefGoogle Scholar
Molnár, L., ‘Reflexivity of the automorphism and isometry groups of ${C}^{\ast }$ -algebras in BDF theory’, Arch. Math. 74 (2000), 120–128.10.1007/PL00000417CrossRefGoogle Scholar
Molnár, L., ‘2-local isometries of some operator algebras’, Proc. Edinb. Math. Soc. (2) 45 (2002), 349–352.10.1017/S0013091500000043CrossRefGoogle Scholar
Molnár, L., ‘Some characterizations of the automorphisms of $B(H)$ and $C(X)$ ’, Proc. Amer. Math. Soc. 130 (2002), 111–120.10.1090/S0002-9939-01-06172-XCrossRefGoogle Scholar
Molnár, L., Selected Preserver Problems on Algebraic Structures of Linear Operators and on Function Spaces (Springer, Berlin, 2007).Google Scholar
Molnár, L., Private communication with O. Hatori, 2018.Google Scholar
Molnár, L., ‘On 2-local *-automorphisms and 2-local isometries of $B(H)$ ’, J. Math. Anal. Appl. 479(1) (2019), 569–580.10.1016/j.jmaa.2019.06.038CrossRefGoogle Scholar
Molnár, L. and Györy, M., ‘Reflexivity of the automorphism and isometry groups of the suspension of $B(H)$ ’, J. Funct. Anal. 159 (1998), 568–586.10.1006/jfan.1998.3325CrossRefGoogle Scholar
Molnár, L. and Zalar, B., ‘Reflexivity of the group of surjective isometries on some Banach spaces’, Proc. Edinb. Math. Soc. 42 (1999), 17–36.10.1017/S0013091500019982CrossRefGoogle Scholar
Mori, M., ‘On 2-local nonlinear surjective isometries on normed spaces and C*-algebras’, Proc. Amer. Math. Soc. 148(6) (2020), 2477–2485.10.1090/proc/14949CrossRefGoogle Scholar
Niazi, M. and Peralta, A. M., ‘Weak-2-local $\ast -$ derivations on $B(H)$ are linear $\ast -$ derivations’, Linear Algebra Appl. 487 (2015), 276–300.10.1016/j.laa.2015.09.028CrossRefGoogle Scholar
Niazi and, M. Peralta, A. M., ‘Weak-2-local derivations on ${M}_n$ ’, FILOMAT 31(6) (2017), 1687–1708.10.2298/FIL1706687NCrossRefGoogle Scholar
Oi, S., ‘Algebraic reflexivity of isometry groups of algebras of Lipschitz maps’, Linear Algebra Appl. 566 (2019), 167–182.10.1016/j.laa.2018.12.033CrossRefGoogle Scholar
Rao, N. V. and Roy, A. K., ‘Linear isometries of some function spaces’, Pacific J. Math. 38 (1971), 177–192.10.2140/pjm.1971.38.177CrossRefGoogle Scholar
Šemrl, P., ‘Local automorphisms and derivations on $B(H)$ ’, Proc. Amer. Math. Soc. 125 (1997), 2677–2680.10.1090/S0002-9939-97-04073-2CrossRefGoogle Scholar
Żelazko, W., ‘A characterization of multiplicative linear functionals in complex Banach algebras’, Studia Math. 30 (1968), 83–85.10.4064/sm-30-1-83-85CrossRefGoogle Scholar