Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Tian, Zhenji
2007.
Zero-Semidistributive Inverse Semigroups.
Communications in Algebra,
Vol. 35,
Issue. 6,
p.
1824.
Tian, Z. J.
2007.
The lattice of completely simple subsemigroups of a completely simple semigroup.
Vestnik St. Petersburg University: Mathematics,
Vol. 40,
Issue. 1,
p.
89.
Wang, Yu
and
Luo, Yanfeng
2010.
Eventually regular semigroups with 0-semidistributive full subsemigroup lattices.
Algebra universalis,
Vol. 64,
Issue. 3-4,
p.
419.
Cheong, Kyeong Hee
and
Jones, Peter R.
2011.
Semidistributive Inverse Semigroups, II.
Communications in Algebra,
Vol. 39,
Issue. 3,
p.
972.
Wang, Yu
and
Yin, Zhixiang
2013.
TWO PARTICULAR EVENTUALLY REGULAR SEMIGROUPS WITH 0-MODULAR OR 0-DISTRIBUTIVE SUBSEMIGROUP LATTICES.
Asian-European Journal of Mathematics,
Vol. 06,
Issue. 04,
p.
1350046.
Cornejo, Juan M.
and
Sankappanavar, Hanamantagouda P.
2021.
Semidistributivity and Whitman Property in implication zroupoids.
Mathematica Slovaca,
Vol. 71,
Issue. 6,
p.
1329.


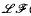 (
(