Article contents
THE REGULAR PART OF A SEMIGROUP OF LINEAR TRANSFORMATIONS WITH RESTRICTED RANGE
Published online by Cambridge University Press: 20 February 2017
Abstract
Let 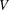 $V$ be a vector space and let
$V$ be a vector space and let 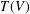 $T(V)$ denote the semigroup (under composition) of all linear transformations from
$T(V)$ denote the semigroup (under composition) of all linear transformations from 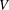 $V$ into
$V$ into 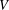 $V$. For a fixed subspace
$V$. For a fixed subspace 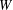 $W$ of
$W$ of 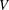 $V$, let
$V$, let 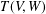 $T(V,W)$ be the semigroup consisting of all linear transformations from
$T(V,W)$ be the semigroup consisting of all linear transformations from 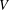 $V$ into
$V$ into 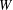 $W$. In 2008, Sullivan [‘Semigroups of linear transformations with restricted range’, Bull. Aust. Math. Soc.77(3) (2008), 441–453] proved that
$W$. In 2008, Sullivan [‘Semigroups of linear transformations with restricted range’, Bull. Aust. Math. Soc.77(3) (2008), 441–453] proved that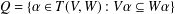 $$\begin{eqnarray}\displaystyle Q=\{\unicode[STIX]{x1D6FC}\in T(V,W):V\unicode[STIX]{x1D6FC}\subseteq W\unicode[STIX]{x1D6FC}\} & & \displaystyle \nonumber\end{eqnarray}$$
$$\begin{eqnarray}\displaystyle Q=\{\unicode[STIX]{x1D6FC}\in T(V,W):V\unicode[STIX]{x1D6FC}\subseteq W\unicode[STIX]{x1D6FC}\} & & \displaystyle \nonumber\end{eqnarray}$$ $T(V,W)$ and characterized Green’s relations on
$T(V,W)$ and characterized Green’s relations on 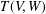 $T(V,W)$. In this paper, we determine all the maximal regular subsemigroups of
$T(V,W)$. In this paper, we determine all the maximal regular subsemigroups of 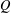 $Q$ when
$Q$ when 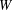 $W$ is a finite-dimensional subspace of
$W$ is a finite-dimensional subspace of 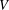 $V$ over a finite field. Moreover, we compute the rank and idempotent rank of
$V$ over a finite field. Moreover, we compute the rank and idempotent rank of 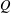 $Q$ when
$Q$ when 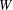 $W$ is an
$W$ is an  $n$-dimensional subspace of an
$n$-dimensional subspace of an 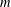 $m$-dimensional vector space
$m$-dimensional vector space 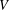 $V$ over a finite field
$V$ over a finite field 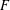 $F$.
$F$.
MSC classification
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 103 , Issue 3 , December 2017 , pp. 402 - 419
- Copyright
- © 2017 Australian Mathematical Publishing Association Inc.
Footnotes
This research was supported by Chiang Mai University.
References
- 5
- Cited by


