Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Xie, Huayou
Liu, Junming
and
Ponnusamy, Saminathan
2023.
Volterra-type operators on the minimal Möbius-invariant space.
Canadian Mathematical Bulletin,
Vol. 66,
Issue. 2,
p.
509.
Mashreghi, Javad
2023.
Lectures on Analytic Function Spaces and their Applications.
Vol. 39,
Issue. ,
p.
1.
Hai Khoi, Le
and
Mashreghi, Javad
2023.
Theory of Np Spaces.
p.
27.


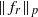 as
as 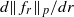 grows at most like
grows at most like 