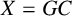1 Introduction
A group G is said to be properly factorizable if
![]() $G=AB$
for two proper subgroups A and B of G, while the expression
$G=AB$
for two proper subgroups A and B of G, while the expression
![]() $G=AB$
is called a factorization of G. Furthermore, if
$G=AB$
is called a factorization of G. Furthermore, if
![]() $A\cap B=1$
, then we say that G has an exact factorization.
$A\cap B=1$
, then we say that G has an exact factorization.
Factorizations of groups naturally arise from the well-known Frattini’s argument, including its version in permutation groups. One of the most famous results about factorized groups might be one of the theorems of Itô, saying that any group is metabelian whenever it is the product of two abelian subgroups (see [Reference Itô11]). Later, Wielandt and Kegel showed that the product of two nilpotent subgroups must be soluble (see [Reference Kegel13, Reference Wielandt19]). Douglas showed that the product of two cyclic groups must be super-solvable (see [Reference Douglas3]). The factorizations of almost simple groups with a solvable factor were determined in [Reference Li and Xia15]. There are many other papers related to factorizations, for instance, finite products of soluble groups, factorizations with one nilpotent factor and so on. Here we are not able to list all references and readers may refer to a survey paper [Reference Amberg and Kazarin2].
In this paper, we shall focus on the product group
![]() $X=GC$
for a finite group G and a cyclic group C such that
$X=GC$
for a finite group G and a cyclic group C such that
![]() $G\cap C=1$
. Suppose that C is core-free. Then, X is also called a skew product group of G. Recall that the skew morphism of a group G and a skew product group X of G were introduced by Jajcay and Širáň in [Reference Jajcay and Širáň12], which is related to studies of regular Cayley maps of G. For the reason of the length of the paper, we are not able to explain them in detail. Recently, there have been many results on skew product groups X of some particular groups G that are cyclic groups, elementary abelian p-groups, finite nonabelian characteristically simple groups, dihedral groups, generalized quaternion groups and so on. In particular, so far, there exists no classification of skew product groups of cyclic groups, and based on big efforts of several authors working on regular Cayley maps, the final classification of skew product groups of dihedral groups was given in [Reference Hu, Kovács and Kwon8]. For partial results about generalized quaternion groups, see [Reference Hu and Ruan9].
$G\cap C=1$
. Suppose that C is core-free. Then, X is also called a skew product group of G. Recall that the skew morphism of a group G and a skew product group X of G were introduced by Jajcay and Širáň in [Reference Jajcay and Širáň12], which is related to studies of regular Cayley maps of G. For the reason of the length of the paper, we are not able to explain them in detail. Recently, there have been many results on skew product groups X of some particular groups G that are cyclic groups, elementary abelian p-groups, finite nonabelian characteristically simple groups, dihedral groups, generalized quaternion groups and so on. In particular, so far, there exists no classification of skew product groups of cyclic groups, and based on big efforts of several authors working on regular Cayley maps, the final classification of skew product groups of dihedral groups was given in [Reference Hu, Kovács and Kwon8]. For partial results about generalized quaternion groups, see [Reference Hu and Ruan9].
Throughout this paper, set
![]() $C=\langle c\rangle $
and
$C=\langle c\rangle $
and
 $$ \begin{align} &Q=\langle a, b \mid a^{2n}=1,b^2=a^n,a^b=a^{-1}\rangle \cong Q_{4n},\,n\ge 2,\notag\\ &D=\langle a, b \mid a^{n}=b^2=1, a^b=a^{-1}\rangle \cong D_{2n}, \, n\ge 2. \end{align} $$
$$ \begin{align} &Q=\langle a, b \mid a^{2n}=1,b^2=a^n,a^b=a^{-1}\rangle \cong Q_{4n},\,n\ge 2,\notag\\ &D=\langle a, b \mid a^{n}=b^2=1, a^b=a^{-1}\rangle \cong D_{2n}, \, n\ge 2. \end{align} $$
Let
![]() $X(G)=GC$
be a group, where
$X(G)=GC$
be a group, where
![]() $G\in \{Q,\,D\}$
and
$G\in \{Q,\,D\}$
and
![]() $G\cap C=1$
. In this paper, we give a characterization for
$G\cap C=1$
. In this paper, we give a characterization for
![]() $X(Q)$
and a complete classification of
$X(Q)$
and a complete classification of
![]() $X(Q)$
provided that C is core-free. In the above paper dealing with skew product groups of dihedral groups, the authors adopt some computational techniques on skew morphisms. Alternatively, in this paper, we realize our goals by using classical group theoretical tools and methods (solvable groups, p-groups, permutation groups, group extension theory and so on). In our approach, we pay attention to the global structures of the group
$X(Q)$
provided that C is core-free. In the above paper dealing with skew product groups of dihedral groups, the authors adopt some computational techniques on skew morphisms. Alternatively, in this paper, we realize our goals by using classical group theoretical tools and methods (solvable groups, p-groups, permutation groups, group extension theory and so on). In our approach, we pay attention to the global structures of the group
![]() $X(G)$
. Since
$X(G)$
. Since
![]() $X(Q)$
is closely related to
$X(Q)$
is closely related to
![]() $X(D)$
, some properties of
$X(D)$
, some properties of
![]() $X(D)$
have to be considered too.
$X(D)$
have to be considered too.
Note that
![]() $X=X(G)=GC=\langle a, b\rangle \langle c\rangle $
for
$X=X(G)=GC=\langle a, b\rangle \langle c\rangle $
for
![]() $G\in \{ Q, D\}$
. Thus,
$G\in \{ Q, D\}$
. Thus,
![]() $\langle a\rangle \langle c\rangle $
is unnecessarily a subgroup of X. Clearly, X contains a subgroup M of the largest order such that
$\langle a\rangle \langle c\rangle $
is unnecessarily a subgroup of X. Clearly, X contains a subgroup M of the largest order such that
![]() $\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
. This subgroup M plays an important role in this paper. From now on, by
$\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
. This subgroup M plays an important role in this paper. From now on, by
![]() $S_X$
, we denote the core
$S_X$
, we denote the core
![]() $\cap _{x\in X}S^x$
of X in a subgroup S of X.
$\cap _{x\in X}S^x$
of X in a subgroup S of X.
There are four main theorems in this manuscript. In Theorem 1.1, the global structure of our group
![]() $X\in \{X(Q), X(D)\}$
is characterized.
$X\in \{X(Q), X(D)\}$
is characterized.
Theorem 1.1. Let
![]() $G\in \{ Q,\,D\}$
and
$G\in \{ Q,\,D\}$
and
![]() $X=X(G)=GC$
, where
$X=X(G)=GC$
, where
![]() $G\cap C=1$
. Let M be the subgroup of the largest order in X such that
$G\cap C=1$
. Let M be the subgroup of the largest order in X such that
![]() $\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
. Then the group M is characterized in Table 1.
$\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
. Then the group M is characterized in Table 1.
Table 1 The forms of M,
![]() $M_X$
and
$M_X$
and
![]() $X/M_X$
.
$X/M_X$
.

Clearly, M is a product of two cyclic subgroups, which has not been determined in general so far, as mentioned before. However, further properties of our group X are given in Theorem 1.2.
Theorem 1.2. Let
![]() $G\in \{Q, D\}$
and
$G\in \{Q, D\}$
and
![]() $X=X(G)$
, and let M be defined as above. Then we have
$X=X(G)$
, and let M be defined as above. Then we have
![]() $\langle a^2, c\rangle \le C_X(\langle c\rangle _X)$
and
$\langle a^2, c\rangle \le C_X(\langle c\rangle _X)$
and
![]() $|X: C_X(\langle c\rangle _X)|\le 4$
. Moreover, if
$|X: C_X(\langle c\rangle _X)|\le 4$
. Moreover, if
![]() $\langle c\rangle _X=1$
, then
$\langle c\rangle _X=1$
, then
![]() $M_X\cap \langle a^2\rangle \lhd M_X$
. In particular, if
$M_X\cap \langle a^2\rangle \lhd M_X$
. In particular, if
![]() $\langle c\rangle _X=1$
and
$\langle c\rangle _X=1$
and
![]() $M=\langle a\rangle \langle c\rangle $
, then
$M=\langle a\rangle \langle c\rangle $
, then
![]() $\langle a^2\rangle \lhd X$
.
$\langle a^2\rangle \lhd X$
.
Using Theorem 1.2, we classify skew product groups of generalized quaternion groups; see the following theorem.
Theorem 1.3. Let
![]() $G=Q$
and
$G=Q$
and
![]() $X=X(G)=G\langle c\rangle $
, where
$X=X(G)=G\langle c\rangle $
, where
![]() $m={\mathrm {o}}(c)\ge 2$
,
$m={\mathrm {o}}(c)\ge 2$
,
![]() $G\cap \langle c\rangle =1$
and
$G\cap \langle c\rangle =1$
and
![]() $\langle c\rangle _X=1$
. Set
$\langle c\rangle _X=1$
. Set
![]() $R:=\{a^{2n}=c^m=1,\,b^2=a^n,\,a^b=a^{-1}\}$
. Then, one of the following holds:
$R:=\{a^{2n}=c^m=1,\,b^2=a^n,\,a^b=a^{-1}\}$
. Then, one of the following holds:
-
(1)
 $X=\langle a,b,c\mid R, (a^2)^c=a^{2r}, c^a=a^{2s}c^t,c^b=a^uc^v\rangle ;$
$X=\langle a,b,c\mid R, (a^2)^c=a^{2r}, c^a=a^{2s}c^t,c^b=a^uc^v\rangle ;$
-
(2)
 $X=\langle a,b,c\mid R,(a^2)^{c^2}=a^{2r},(c^2)^a=a^{2s}c^{2t},(c^2)^b=a^{2u}c^{2}, a^c=bc^{2w}\rangle ;$
$X=\langle a,b,c\mid R,(a^2)^{c^2}=a^{2r},(c^2)^a=a^{2s}c^{2t},(c^2)^b=a^{2u}c^{2}, a^c=bc^{2w}\rangle ;$
-
(3)
 $X=\langle a,b,c\mid R, (a^2)^{c}=a^{2r},(c^3)^a=a^{2s}c^3,(c^3)^b=a^{2u}c^{3}, a^c=bc^{{im}/2},b^c=a^xb\rangle ;$
$X=\langle a,b,c\mid R, (a^2)^{c}=a^{2r},(c^3)^a=a^{2s}c^3,(c^3)^b=a^{2u}c^{3}, a^c=bc^{{im}/2},b^c=a^xb\rangle ;$
-
(4)
 $X=\langle a,b,c\mid R, (a^4)^{c}=a^{4r}, (c^3)^{a^2}=a^{4s}c^3,(c^2)^b=a^{4u}c^3, (a^2)^c=bc^{{im}/2},$
$X=\langle a,b,c\mid R, (a^4)^{c}=a^{4r}, (c^3)^{a^2}=a^{4s}c^3,(c^2)^b=a^{4u}c^3, (a^2)^c=bc^{{im}/2},$
 $b^c=a^{2x}b, c^a=a^{2+4z}c^{1+ ({jm}/3)}\rangle ;$
$b^c=a^{2x}b, c^a=a^{2+4z}c^{1+ ({jm}/3)}\rangle ;$
-
(5)
 $X=\langle a,b,c\mid R,a^{c^4}=a^r, b^{c^4}=a^{1-r}b, (a^3)^{c^{m/4}}=a^{-3}, a^{c^{m/4}}=bc^{{3m}/4}\rangle $
,
$X=\langle a,b,c\mid R,a^{c^4}=a^r, b^{c^4}=a^{1-r}b, (a^3)^{c^{m/4}}=a^{-3}, a^{c^{m/4}}=bc^{{3m}/4}\rangle $
,
where the parameters are given in Lemmas 5.1, 5.2, 5.3, 5.5 and 5.6, respectively.
The relationship between
![]() $X(Q)$
and
$X(Q)$
and
![]() $X(D)$
is characterized in the following theorem.
$X(D)$
is characterized in the following theorem.
Theorem 1.4. For the group
![]() $X(Q)$
, we have
$X(Q)$
, we have
![]() $\langle a^n\rangle \lhd X(Q)$
for all cases in items (2)–(5) and some cases in item (1). Moreover, corresponding to
$\langle a^n\rangle \lhd X(Q)$
for all cases in items (2)–(5) and some cases in item (1). Moreover, corresponding to
![]() $D\cong Q/\langle a^n\rangle $
, we have
$D\cong Q/\langle a^n\rangle $
, we have
![]() $X(D)=X(Q)/\langle a^n, c_1\rangle $
, where
$X(D)=X(Q)/\langle a^n, c_1\rangle $
, where
![]() $\langle a^n\rangle \lhd X(Q)$
and
$\langle a^n\rangle \lhd X(Q)$
and
![]() $\langle a^n, c_1\rangle =\langle a^n,\,c\rangle _{X(Q)}$
.
$\langle a^n, c_1\rangle =\langle a^n,\,c\rangle _{X(Q)}$
.
Remark 1.5. One may determine regular Cayley maps of dihedral groups (which was done in [Reference Kovács and Kwon14] via skew-morphism computations) and of generalized quaternion groups by Theorem 1.3.
After this introductory section, some preliminary results are given in Section 2; and Theorems 1.1, 1.2, 1.3 and 1.4 are proved in Sections 3, 4, 5 and 6, respectively.
2 Preliminaries
In this section, some elementary facts used in this paper are collected.
Proposition 2.1 [Reference Monakhov17, Theorem 1].
The finite group
![]() $G=AB$
is solvable, where both A and B are subgroups with cyclic subgroups of index no more than 2.
$G=AB$
is solvable, where both A and B are subgroups with cyclic subgroups of index no more than 2.
Proposition 2.2 [Reference Huppert10, Theorem 4.5].
Let H be a subgroup of G. Then
![]() $N_G(H)/C_G(H)$
is isomorphic to a subgroup of
$N_G(H)/C_G(H)$
is isomorphic to a subgroup of
![]() ${\mathrm {Aut\,}}(H)$
.
${\mathrm {Aut\,}}(H)$
.
Proposition 2.3 [Reference Lucchini16, Theorem].
If G is a transitive permutation group of degree n with a cyclic point-stabilizer, then
![]() $|G|\le n(n-1)$
.
$|G|\le n(n-1)$
.
Proposition 2.4 [Reference Itô11, Satz 1 and Satz 2].
Let
![]() $G=AB$
be a group, where both A and B are abelian subgroups of G. Then:
$G=AB$
be a group, where both A and B are abelian subgroups of G. Then:
-
(1) G is meta-abelian, that is,
 $G'$
is abelian; and
$G'$
is abelian; and -
(2) A or B contains a normal subgroup
 $N\ne 1$
of G if
$N\ne 1$
of G if
 $G\ne 1$
.
$G\ne 1$
.
Proposition 2.5 [Reference Huppert10, Theorem 11.5].
Let
![]() $G=\langle a\rangle \langle b\rangle $
be a group and p be an odd prime. If
$G=\langle a\rangle \langle b\rangle $
be a group and p be an odd prime. If
![]() $|\langle a\rangle |\leq |\langle b\rangle |$
, then
$|\langle a\rangle |\leq |\langle b\rangle |$
, then
![]() $\langle b\rangle _G\ne 1$
. If both
$\langle b\rangle _G\ne 1$
. If both
![]() $\langle a\rangle $
and
$\langle a\rangle $
and
![]() $\langle b\rangle $
are p-groups, then G is metacyclic.
$\langle b\rangle $
are p-groups, then G is metacyclic.
Proposition 2.6 [Reference Amberg, Franciosi and de Giovanni1, Corollary 1.3.3].
Let
![]() $G=G_1G_2$
be a group. Then for any prime p, there exists
$G=G_1G_2$
be a group. Then for any prime p, there exists
![]() $P_i\in Syl_p(G_i)$
such that
$P_i\in Syl_p(G_i)$
such that
![]() $P=P_1P_2\in Syl_p(G)$
for
$P=P_1P_2\in Syl_p(G)$
for
![]() $i=1$
or
$i=1$
or
![]() $2$
.
$2$
.
Proposition 2.7 [Reference Gaschütz7, Satz 1].
Let
![]() $N\leq M\leq G$
such that
$N\leq M\leq G$
such that
![]() $(|N|,|G:M|)=1$
and N is an abelian normal subgroup of G. If N has a complement in M, then N has a complement in G too.
$(|N|,|G:M|)=1$
and N is an abelian normal subgroup of G. If N has a complement in M, then N has a complement in G too.
The Schur multiplier
![]() $M(G)$
of a group G is defined as the second integral homology group
$M(G)$
of a group G is defined as the second integral homology group
![]() $H^2(G; Z)$
, where Z is a trivial G-module. It plays an important role in the central expansion of groups. The following result is well known.
$H^2(G; Z)$
, where Z is a trivial G-module. It plays an important role in the central expansion of groups. The following result is well known.
Proposition 2.8 [Reference Suzuki18, (2.21) on page 301].
The Schur multiplier
![]() $M(S_n)$
of
$M(S_n)$
of
![]() $S_n$
is a cyclic group of order 2 if
$S_n$
is a cyclic group of order 2 if
![]() $n\ge 4$
and of order 1 for
$n\ge 4$
and of order 1 for
![]() $n\leq 3$
.
$n\leq 3$
.
Recall that a group H is said to be a Burnside group if every permutation group containing a regular subgroup isomorphic to H is either 2-transitive or imprimitive.
Proposition 2.9 [Reference Wielandt20, Theorems 25.3 and 25.6].
Every cyclic group of composite order is a Burnside group. Every dihedral group is a Burnside group.
Proposition 2.10 [Reference Du, Malnič and Marušič5, Lemma 4.1].
Let
![]() $n\ge 2$
be an integer and p a prime. Then,
$n\ge 2$
be an integer and p a prime. Then,
![]() ${\mathrm {AGL}}(n, p)$
contains an element of order
${\mathrm {AGL}}(n, p)$
contains an element of order
![]() $p^n$
if and only if
$p^n$
if and only if
![]() $(n, p)=(2, 2)$
and
$(n, p)=(2, 2)$
and
![]() ${\mathrm {AGL}}(2, 2)\cong S_4$
.
${\mathrm {AGL}}(2, 2)\cong S_4$
.
Recall that our group
![]() $X(D)=DC$
, where D is a dihedral group of order
$X(D)=DC$
, where D is a dihedral group of order
![]() $2n$
and C is a cyclic group of order m such that
$2n$
and C is a cyclic group of order m such that
![]() $D\cap C=1$
, where
$D\cap C=1$
, where
![]() $n, m\ge 2$
. Then, we have the following results.
$n, m\ge 2$
. Then, we have the following results.
Proposition 2.11 [Reference Du and Zhang6, Lemma 4.1].
Suppose that
![]() $X(D)=\langle a,b\rangle \langle c\rangle ,\,\langle a\rangle \langle c\rangle \leq X(D)$
and
$X(D)=\langle a,b\rangle \langle c\rangle ,\,\langle a\rangle \langle c\rangle \leq X(D)$
and
![]() $\langle c\rangle _{X(D)}=1$
. Then,
$\langle c\rangle _{X(D)}=1$
. Then,
![]() $\langle a^2\rangle \lhd X(D)$
.
$\langle a^2\rangle \lhd X(D)$
.
Remark 2.12. An independent proof of Proposition 2.11 was also given in [Reference Du, Luo and Yu4].
Lemma 2.13. Suppose that X is a solvable group and has a faithfully
![]() $2$
-transitive permutation representation relative to a subgroup M, whose index is of composite order. Then,
$2$
-transitive permutation representation relative to a subgroup M, whose index is of composite order. Then,
![]() $X\leq {\mathrm {AGL}}(k,p)$
, where k is a positive integer and p is a prime. Moreover:
$X\leq {\mathrm {AGL}}(k,p)$
, where k is a positive integer and p is a prime. Moreover:
-
(i) if X contains an element of order
 $p^k$
, then
$p^k$
, then
 $X=S_4$
; and
$X=S_4$
; and -
(ii) if the hypotheses hold for
 $X=X(D)$
and
$X=X(D)$
and
 $M=C$
, then
$M=C$
, then
 $X(D)=A_4$
.
$X(D)=A_4$
.
Proof. Set
![]() $\Omega =[X:M]$
. Let N be a minimal normal subgroup of X. Since X is solvable,
$\Omega =[X:M]$
. Let N be a minimal normal subgroup of X. Since X is solvable,
![]() $N\cong {\mathbb {Z}}_p^k$
for some prime p and integer k. Since X is 2-transitive, it is primitive, which implies that N is transitive on
$N\cong {\mathbb {Z}}_p^k$
for some prime p and integer k. Since X is 2-transitive, it is primitive, which implies that N is transitive on
![]() $\Omega $
and so is regular on
$\Omega $
and so is regular on
![]() $\Omega $
. Therefore,
$\Omega $
. Therefore,
![]() $X=N\rtimes X_\alpha \le {\mathrm {AGL}}(k,p)$
for some
$X=N\rtimes X_\alpha \le {\mathrm {AGL}}(k,p)$
for some
![]() $\alpha \in \Omega $
. Since X is 2-transitive and
$\alpha \in \Omega $
. Since X is 2-transitive and
![]() $|\Omega |=p^k$
, we know
$|\Omega |=p^k$
, we know
![]() $|X_\alpha |\ge p^k-1$
for any
$|X_\alpha |\ge p^k-1$
for any
![]() $\alpha \in \Omega $
.
$\alpha \in \Omega $
.
-
(i) Suppose that X contains an element of order
 $p^k$
. By Proposition 2.10, we get
$p^k$
. By Proposition 2.10, we get
 $(k,p)=(2,2)$
so that
$(k,p)=(2,2)$
so that
 $X=S_4$
, reminding us that
$X=S_4$
, reminding us that
 $|\Omega |$
is not a prime.
$|\Omega |$
is not a prime. -
(ii) Let
 $X=X(D)$
and
$X=X(D)$
and
 $M=C=\langle c\rangle $
with the order of m. Then,
$M=C=\langle c\rangle $
with the order of m. Then,
 $|X(D)|=2nm=p^km$
. By Proposition 2.3, we get
$|X(D)|=2nm=p^km$
. By Proposition 2.3, we get
 $p^km\leq p^k(p^k-1)$
. Therefore,
$p^km\leq p^k(p^k-1)$
. Therefore,
 $m=p^k-1$
as
$m=p^k-1$
as
 $m=|M|=|X_{\alpha }|\geq p^k-1$
, which implies that
$m=|M|=|X_{\alpha }|\geq p^k-1$
, which implies that
 $\langle c\rangle $
is a Singer subgroup of
$\langle c\rangle $
is a Singer subgroup of
 ${\mathrm {GL}}(k,p)$
and
${\mathrm {GL}}(k,p)$
and
 $X(D)=N\rtimes \langle c\rangle $
. Then, both D and N are regular subgroups, that is,
$X(D)=N\rtimes \langle c\rangle $
. Then, both D and N are regular subgroups, that is,
 $|D|=2n=|\Omega |=p^k$
, which implies
$|D|=2n=|\Omega |=p^k$
, which implies
 $p=2$
. Now, we have
$p=2$
. Now, we have
 $|X(D)|=2^{k}(2^k-1)$
. Since both N and D are Sylow 2-subgroups of
$|X(D)|=2^{k}(2^k-1)$
. Since both N and D are Sylow 2-subgroups of
 $X(D)$
and
$X(D)$
and
 $N\lhd X(D)$
, we get
$N\lhd X(D)$
, we get
 $D=N$
, so that
$D=N$
, so that
 $D\cong {\mathbb {Z}}_2^2$
and
$D\cong {\mathbb {Z}}_2^2$
and
 $X(D)=A_4$
.
$X(D)=A_4$
.
3 Proof of Theorem 1.1
To prove Theorem 1.1, let
![]() $G\in \{D,\,Q\}$
, as defined in (1-1). Let
$G\in \{D,\,Q\}$
, as defined in (1-1). Let
![]() $X=G\langle c\rangle$
be either
$X=G\langle c\rangle$
be either
![]() $X(D)$
or
$X(D)$
or
![]() $X(Q)$
. Let M be the subgroup of the largest order in X such that
$X(Q)$
. Let M be the subgroup of the largest order in X such that
![]() $\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
, and set
$\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
, and set
![]() $M_X=\cap _{x\in X}M^x$
. By Proposition 2.1, X is solvable. Then, Theorem 1.1 is proved in Lemmas 3.1 and 3.3, which deal with
$M_X=\cap _{x\in X}M^x$
. By Proposition 2.1, X is solvable. Then, Theorem 1.1 is proved in Lemmas 3.1 and 3.3, which deal with
![]() $G=D$
and Q, respectively.
$G=D$
and Q, respectively.
Lemma 3.1. Theorem 1.1 holds, provided
![]() $G=D$
and
$G=D$
and
![]() $X=X(D)$
.
$X=X(D)$
.
Proof. Let
![]() $G=D$
and
$G=D$
and
![]() $X=X(D)$
. If
$X=X(D)$
. If
![]() $m=1$
, then
$m=1$
, then
![]() $X=D$
and
$X=D$
and
![]() $M=\langle a\rangle $
, as desired. So in what follows, we assume
$M=\langle a\rangle $
, as desired. So in what follows, we assume
![]() $m\ge 2$
. Recall that
$m\ge 2$
. Recall that
![]() $m, n\ge 2$
,
$m, n\ge 2$
,
![]() $|X|$
is even and more than 7. The lemma is proved by induction on the order of X. Up to isomorphism, all cases of
$|X|$
is even and more than 7. The lemma is proved by induction on the order of X. Up to isomorphism, all cases of
![]() $|X|\le 24$
are listed in Table 2, showing the conclusion.
$|X|\le 24$
are listed in Table 2, showing the conclusion.
Assume
![]() $|X|\gneqq 24$
. Then we carry out the proof by the following three steps.
$|X|\gneqq 24$
. Then we carry out the proof by the following three steps.
Step 1: Case of
![]() $M_X\ne 1$
. Suppose that
$M_X\ne 1$
. Suppose that
![]() $M_X\ne 1$
. Set
$M_X\ne 1$
. Set
![]() $M=\langle a^i\rangle \langle c\rangle $
for some i. Since
$M=\langle a^i\rangle \langle c\rangle $
for some i. Since
we get that
![]() $M_X=M_X\cap (\langle a^i\rangle \langle c\rangle )=\langle a^i\rangle \langle c^r\rangle $
for some r. Set
$M_X=M_X\cap (\langle a^i\rangle \langle c\rangle )=\langle a^i\rangle \langle c^r\rangle $
for some r. Set
![]() ${\overline X}:=X/{M_X}={\overline G}\langle {\overline c}\rangle $
. Then we claim that
${\overline X}:=X/{M_X}={\overline G}\langle {\overline c}\rangle $
. Then we claim that
![]() ${\overline G}\cap \langle {\overline c}\rangle =1$
. In fact, for any
${\overline G}\cap \langle {\overline c}\rangle =1$
. In fact, for any
![]() ${\overline g}={\overline c}'\in {\overline G}\cap \langle {\overline c}\rangle $
, for some
${\overline g}={\overline c}'\in {\overline G}\cap \langle {\overline c}\rangle $
, for some
![]() $g\in G$
and
$g\in G$
and
![]() $c'\in \langle {\overline c}\rangle $
, we have
$c'\in \langle {\overline c}\rangle $
, we have
![]() $gc^{\prime -1}\in M_X$
, that is,
$gc^{\prime -1}\in M_X$
, that is,
![]() $g\in \langle a^i\rangle $
and
$g\in \langle a^i\rangle $
and
![]() $c'\in \langle c^r\rangle $
, which implies
$c'\in \langle c^r\rangle $
, which implies
![]() ${\overline g}={\overline c}'=1$
. Therefore,
${\overline g}={\overline c}'=1$
. Therefore,
![]() ${\overline G}\cap \langle {\overline c}\rangle =1$
. Let
${\overline G}\cap \langle {\overline c}\rangle =1$
. Let
![]() $M_0/M_X=\langle {\overline a}^j\rangle \langle {\overline c}\rangle $
be the largest subgroup of
$M_0/M_X=\langle {\overline a}^j\rangle \langle {\overline c}\rangle $
be the largest subgroup of
![]() ${\overline X}$
containing
${\overline X}$
containing
![]() $\langle {\overline c}\rangle $
and contained in the subset
$\langle {\overline c}\rangle $
and contained in the subset
![]() $\langle {\overline a}\rangle \langle {\overline c}\rangle $
. Then,
$\langle {\overline a}\rangle \langle {\overline c}\rangle $
. Then,
![]() $\langle {\overline a}^j\rangle \langle {\overline c}\rangle =\langle {\overline c}\rangle \langle {\overline a}^j\rangle $
. Since
$\langle {\overline a}^j\rangle \langle {\overline c}\rangle =\langle {\overline c}\rangle \langle {\overline a}^j\rangle $
. Since
we get
![]() $\langle a^i, a^j\rangle \langle c\rangle \le X$
. By the maximality of M, we have
$\langle a^i, a^j\rangle \langle c\rangle \le X$
. By the maximality of M, we have
![]() $\langle a^i, a^j\rangle =\langle a^i\rangle $
so that
$\langle a^i, a^j\rangle =\langle a^i\rangle $
so that
![]() $M_0=M$
.
$M_0=M$
.
Table 2 All cases of
![]() $|X|\le 24$
up to isomorphism.
$|X|\le 24$
up to isomorphism.

Using the induction hypothesis on
![]() ${\overline X}={\overline G}\langle {\overline c}\rangle $
, noting that
${\overline X}={\overline G}\langle {\overline c}\rangle $
, noting that
![]() $M_0/M_X=M/M_X$
is core-free in
$M_0/M_X=M/M_X$
is core-free in
![]() ${\overline X}$
, we get that
${\overline X}$
, we get that
![]() ${\overline X}$
is isomorphic to
${\overline X}$
is isomorphic to
![]() ${\mathbb {Z}}_2,\,D_8,\,A_4$
or
${\mathbb {Z}}_2,\,D_8,\,A_4$
or
![]() $S_4$
, and correspondingly,
$S_4$
, and correspondingly,
![]() $o({\overline a})=k$
, where
$o({\overline a})=k$
, where
![]() $k\in \{1, 2, 3, 4\}$
, and so
$k\in \{1, 2, 3, 4\}$
, and so
![]() $a^k\in M_X$
. Since
$a^k\in M_X$
. Since
![]() $M=\langle a^i\rangle \langle c\rangle $
and
$M=\langle a^i\rangle \langle c\rangle $
and
![]() $M_X=\langle a^i\rangle \langle c^r\rangle $
, we know that
$M_X=\langle a^i\rangle \langle c^r\rangle $
, we know that
![]() $\langle a^i\rangle =\langle a^k\rangle $
, which implies that
$\langle a^i\rangle =\langle a^k\rangle $
, which implies that
![]() $i\in \{1, 2, 3, 4\}$
. Clearly, if
$i\in \{1, 2, 3, 4\}$
. Clearly, if
![]() ${\overline X}={\mathbb {Z}}_2$
, then
${\overline X}={\mathbb {Z}}_2$
, then
![]() $M_X=M$
; if
$M_X=M$
; if
![]() ${\overline X}=D_8$
and
${\overline X}=D_8$
and
![]() ${\mathrm {o}}({\overline c})=2$
, then
${\mathrm {o}}({\overline c})=2$
, then
![]() $M_X=\langle a^2\rangle \langle c^2\rangle $
; if
$M_X=\langle a^2\rangle \langle c^2\rangle $
; if
![]() ${\overline X} =A_4$
and
${\overline X} =A_4$
and
![]() ${\mathrm {o}}({\overline c})=3$
, then
${\mathrm {o}}({\overline c})=3$
, then
![]() $M_X=\langle a^2\rangle \langle c^3\rangle $
; if
$M_X=\langle a^2\rangle \langle c^3\rangle $
; if
![]() ${\overline X} =S_4$
and
${\overline X} =S_4$
and
![]() ${\mathrm {o}}({\overline c})=4$
, then
${\mathrm {o}}({\overline c})=4$
, then
![]() $M_X=\langle a^3\rangle \langle c^4\rangle $
; and if
$M_X=\langle a^3\rangle \langle c^4\rangle $
; and if
![]() ${\overline X}=S_4$
and
${\overline X}=S_4$
and
![]() ${\mathrm {o}}({\overline c})=3$
, then
${\mathrm {o}}({\overline c})=3$
, then
![]() $M_X=\langle a^4\rangle \langle c^3\rangle $
.
$M_X=\langle a^4\rangle \langle c^3\rangle $
.
Step 2: Show that if
![]() $M_X=1$
, then
$M_X=1$
, then
![]() $G\in \{D_{2kp} | k=2,3,4$
and p is a prime
$G\in \{D_{2kp} | k=2,3,4$
and p is a prime
![]() $\}$
. Suppose that
$\}$
. Suppose that
![]() $M_X=1$
. Since both
$M_X=1$
. Since both
![]() $\langle a\rangle _X$
and
$\langle a\rangle _X$
and
![]() $\langle c\rangle _X$
are contained in
$\langle c\rangle _X$
are contained in
![]() $M_X$
, we get
$M_X$
, we get
![]() $\langle a\rangle _X=\langle c\rangle _X=1$
. Arguing by contradiction, assume that
$\langle a\rangle _X=\langle c\rangle _X=1$
. Arguing by contradiction, assume that
![]() $G_X\ne 1$
. If
$G_X\ne 1$
. If
![]() $|G_X|\gneqq 4$
, then by
$|G_X|\gneqq 4$
, then by
![]() $G=\langle a, b\rangle \cong D_{2n}$
, we get
$G=\langle a, b\rangle \cong D_{2n}$
, we get
![]() $\langle a\rangle _X\ne 1$
, which is a contradiction. So
$\langle a\rangle _X\ne 1$
, which is a contradiction. So
![]() $|G_X|\le 4$
. Since
$|G_X|\le 4$
. Since
![]() $G_X\lhd G\cong D_{2n}$
, we know that
$G_X\lhd G\cong D_{2n}$
, we know that
![]() $|G:G_X|\le 2$
, which implies
$|G:G_X|\le 2$
, which implies
![]() $|G|\le 8$
, that is,
$|G|\le 8$
, that is,
![]() $G\cong D_4$
or
$G\cong D_4$
or
![]() $D_8$
. A direct check shows that X is
$D_8$
. A direct check shows that X is
![]() $D_8$
,
$D_8$
,
![]() $A_4$
or
$A_4$
or
![]() $S_4$
, which contradicts
$S_4$
, which contradicts
![]() $|X|\gneqq 24$
. Therefore,
$|X|\gneqq 24$
. Therefore,
![]() $G_X=1$
.
$G_X=1$
.
Next, we consider the faithful (right multiplication) action of X on the set of right cosets
![]() $\Omega :=[X:\langle c\rangle ]$
. By Proposition 2.9, every dihedral group is a Burnside group, which implies that X is either 2-transitive or imprimitive. If X is primitive, then noting that X has a cyclic point-stabilizer
$\Omega :=[X:\langle c\rangle ]$
. By Proposition 2.9, every dihedral group is a Burnside group, which implies that X is either 2-transitive or imprimitive. If X is primitive, then noting that X has a cyclic point-stabilizer
![]() $\langle c\rangle $
, by Lemma 2.13(2), we get
$\langle c\rangle $
, by Lemma 2.13(2), we get
![]() $G=D_4$
and
$G=D_4$
and
![]() $X=A_4$
, which contradicts
$X=A_4$
, which contradicts
![]() $|X|\gvertneqq 24$
. So in what follows, we assume that X is imprimitive. Pick a maximal subgroup H of X that contains
$|X|\gvertneqq 24$
. So in what follows, we assume that X is imprimitive. Pick a maximal subgroup H of X that contains
![]() $\langle c\rangle $
properly. Then,
$\langle c\rangle $
properly. Then,
![]() $H=H\cap X=(H\cap G)\langle c\rangle =\langle a^s, b_1\rangle \langle c\rangle <X$
for some
$H=H\cap X=(H\cap G)\langle c\rangle =\langle a^s, b_1\rangle \langle c\rangle <X$
for some
![]() $b_1\in G\setminus \langle a\rangle $
and some s. Using the same argument as that in Step 1, one has
$b_1\in G\setminus \langle a\rangle $
and some s. Using the same argument as that in Step 1, one has
![]() $a^s\in H_X$
. Reset
$a^s\in H_X$
. Reset
![]() ${\overline X}:=X/H_X$
. Consider the faithful primitive action of
${\overline X}:=X/H_X$
. Consider the faithful primitive action of
![]() ${\overline X}$
on
${\overline X}$
on
![]() $\Omega _1:=[{\overline X}:{\overline H}]$
, with a cyclic regular subgroup of
$\Omega _1:=[{\overline X}:{\overline H}]$
, with a cyclic regular subgroup of
![]() $\langle {\overline a}\rangle $
, where
$\langle {\overline a}\rangle $
, where
![]() $|\Omega _1|=s$
. By Proposition 2.9, we know that either s is a prime p such that
$|\Omega _1|=s$
. By Proposition 2.9, we know that either s is a prime p such that
![]() ${\overline X}\le {\mathrm {AGL}}(1,p)$
or s is a composite such that
${\overline X}\le {\mathrm {AGL}}(1,p)$
or s is a composite such that
![]() ${\overline X}$
is 2-transitive on
${\overline X}$
is 2-transitive on
![]() $\Omega _1$
. In what follows, we consider these two cases when
$\Omega _1$
. In what follows, we consider these two cases when
![]() $a^s=1$
or
$a^s=1$
or
![]() $a^s\ne 1$
, separately.
$a^s\ne 1$
, separately.
Case (1):
![]() $a^s=1$
. In this case,
$a^s=1$
. In this case,
![]() $H=\langle c\rangle \rtimes \langle b\rangle $
and
$H=\langle c\rangle \rtimes \langle b\rangle $
and
![]() $X=\langle c, b\rangle .\langle a\rangle $
. Then two cases when s is composite or prime are considered, separately.
$X=\langle c, b\rangle .\langle a\rangle $
. Then two cases when s is composite or prime are considered, separately.
Suppose that s is a composite such that
![]() ${\overline X} $
is 2-transitive on
${\overline X} $
is 2-transitive on
![]() $\Omega _1$
. By Lemma 2.13, we get
$\Omega _1$
. By Lemma 2.13, we get
![]() ${\overline X}\le {\mathrm {AGL}}(l,q)$
for some prime q, which contains a cyclic regular subgroup
${\overline X}\le {\mathrm {AGL}}(l,q)$
for some prime q, which contains a cyclic regular subgroup
![]() $\langle {\overline a}\rangle $
of order
$\langle {\overline a}\rangle $
of order
![]() $q^l$
. By Lemma 2.13(1),
$q^l$
. By Lemma 2.13(1),
![]() ${\overline X}\cong S_4$
and
${\overline X}\cong S_4$
and
![]() ${\mathrm {o}}({\overline a})=4$
so that
${\mathrm {o}}({\overline a})=4$
so that
![]() ${\mathrm {o}}(a)=4$
(as
${\mathrm {o}}(a)=4$
(as
![]() $H_X\le \langle b, c\rangle $
), which in turn implies
$H_X\le \langle b, c\rangle $
), which in turn implies
![]() $G=D_8$
. In this case, checking by Magma, we have that either
$G=D_8$
. In this case, checking by Magma, we have that either
![]() ${\mathrm {o}}(c)=2, 3$
and
${\mathrm {o}}(c)=2, 3$
and
![]() $|X|\le 24$
; or
$|X|\le 24$
; or
![]() ${\mathrm {o}}(c)=4$
,
${\mathrm {o}}(c)=4$
,
![]() $|X|=32$
but
$|X|=32$
but
![]() $G_X\ne 1$
, which is a contradiction.
$G_X\ne 1$
, which is a contradiction.
Suppose that s is a prime p such that
![]() ${\overline X}\le {\mathrm {AGL}}(1,p)$
. Then,
${\overline X}\le {\mathrm {AGL}}(1,p)$
. Then,
![]() ${\mathrm {o}}(a)=p$
such that
${\mathrm {o}}(a)=p$
such that
![]() $G\cong D_{2p}$
, where
$G\cong D_{2p}$
, where
![]() $p\ge 5$
, as
$p\ge 5$
, as
![]() $|X|\gvertneqq 24$
. Clearly,
$|X|\gvertneqq 24$
. Clearly,
![]() $\langle {\overline a}\rangle \lhd {\overline X}$
. Consider the action of X on the set of blocks of length 2 on
$\langle {\overline a}\rangle \lhd {\overline X}$
. Consider the action of X on the set of blocks of length 2 on
![]() $\Omega =[X:\langle c\rangle ]$
, that is, the orbital of
$\Omega =[X:\langle c\rangle ]$
, that is, the orbital of
![]() $\Omega $
under H, with the kernel
$\Omega $
under H, with the kernel
![]() $K:=H_X$
. If
$K:=H_X$
. If
![]() $K=1$
, then we get
$K=1$
, then we get
![]() ${\overline X}=X$
and
${\overline X}=X$
and
![]() $\langle a\rangle \lhd X$
, which is a contradiction. Therefore,
$\langle a\rangle \lhd X$
, which is a contradiction. Therefore,
![]() $K\neq 1$
. Then,
$K\neq 1$
. Then,
![]() $K\nleqq \langle c\rangle $
(as
$K\nleqq \langle c\rangle $
(as
![]() $\langle c\rangle _X=1$
) so that K interchanges two points
$\langle c\rangle _X=1$
) so that K interchanges two points
![]() $\langle c\rangle $
and
$\langle c\rangle $
and
![]() $\langle c\rangle b$
, which implies
$\langle c\rangle b$
, which implies
![]() $|K/K\cap \langle c\rangle |=2$
. Since
$|K/K\cap \langle c\rangle |=2$
. Since
![]() $K\cap \langle c\rangle $
is cyclic and
$K\cap \langle c\rangle $
is cyclic and
![]() $K\cap \langle c\rangle $
fixes setwise each block of length 2, we get
$K\cap \langle c\rangle $
fixes setwise each block of length 2, we get
![]() $|K\cap \langle c\rangle |\leq 2$
. Therefore,
$|K\cap \langle c\rangle |\leq 2$
. Therefore,
![]() $|K|\le 4$
. Since
$|K|\le 4$
. Since
![]() $K\rtimes \langle a\rangle \lhd X$
and
$K\rtimes \langle a\rangle \lhd X$
and
![]() $p\ge 5$
, we have
$p\ge 5$
, we have
![]() $K\rtimes \langle a\rangle =K\times \langle a\rangle $
so that
$K\rtimes \langle a\rangle =K\times \langle a\rangle $
so that
![]() $\langle a\rangle { \, \mathrm {char}\,} (K\times \langle a\rangle )\lhd X$
, which contradicts
$\langle a\rangle { \, \mathrm {char}\,} (K\times \langle a\rangle )\lhd X$
, which contradicts
![]() $G_X=1$
.
$G_X=1$
.
Case (2):
![]() $a^s\neq 1$
. First, show
$a^s\neq 1$
. First, show
![]() $s=p$
, a prime. Arguing by a contradiction, assume that s is composite. To do that, recall
$s=p$
, a prime. Arguing by a contradiction, assume that s is composite. To do that, recall
![]() ${\overline X}=X/H_X=\langle {\overline a}\rangle {\overline H}$
and
${\overline X}=X/H_X=\langle {\overline a}\rangle {\overline H}$
and
![]() $\Omega _1:=[{\overline X}:{\overline H}]$
. Then,
$\Omega _1:=[{\overline X}:{\overline H}]$
. Then,
![]() ${\overline X}$
is 2-transitive on
${\overline X}$
is 2-transitive on
![]() $\Omega _1$
, with a cyclic regular subgroup of
$\Omega _1$
, with a cyclic regular subgroup of
![]() $\langle {\overline a}\rangle $
. Since
$\langle {\overline a}\rangle $
. Since
![]() $a^s\in H_X$
, we get
$a^s\in H_X$
, we get
![]() $H_X\ne 1$
and of course
$H_X\ne 1$
and of course
![]() $H_X\nleqq \langle c\rangle $
. Suppose that
$H_X\nleqq \langle c\rangle $
. Suppose that
![]() $\langle a^j\rangle \langle c\rangle \le H$
. Then
$\langle a^j\rangle \langle c\rangle \le H$
. Then
![]() $a^j\in M$
. Using the same arguments as in the first line of Step 1 again, we get
$a^j\in M$
. Using the same arguments as in the first line of Step 1 again, we get
![]() $a^j\in M_X=1$
. Therefore, there exists an l such that
$a^j\in M_X=1$
. Therefore, there exists an l such that
![]() $bc^l\in H_X$
, which implies
$bc^l\in H_X$
, which implies
![]() ${\overline H}=\langle {\overline c}\rangle $
. Then,
${\overline H}=\langle {\overline c}\rangle $
. Then,
![]() ${\overline X}=\langle {\overline a}\rangle \langle {\overline c}\rangle $
, a product of two cyclic subgroups, cannot be isomorphic to
${\overline X}=\langle {\overline a}\rangle \langle {\overline c}\rangle $
, a product of two cyclic subgroups, cannot be isomorphic to
![]() $S_4$
, which contradicts Lemma 2.13(1). Therefore,
$S_4$
, which contradicts Lemma 2.13(1). Therefore,
![]() $s=p$
, a prime, and
$s=p$
, a prime, and
![]() $X/H_X\le {\mathrm {AGL}}(1, p)$
.
$X/H_X\le {\mathrm {AGL}}(1, p)$
.
Second, consider the quotient group
![]() ${\overline H}:=H/\langle c\rangle _H=\langle {\overline a}^p,{\overline b}\rangle \langle {\overline c}\rangle $
, taking into account
${\overline H}:=H/\langle c\rangle _H=\langle {\overline a}^p,{\overline b}\rangle \langle {\overline c}\rangle $
, taking into account
![]() $s=p$
, a prime. Clearly, we have
$s=p$
, a prime. Clearly, we have
![]() $\langle {\overline c} \rangle _{{\overline H}}=1$
and
$\langle {\overline c} \rangle _{{\overline H}}=1$
and
![]() $o({\overline a}^p)=o(a^p)$
. Let
$o({\overline a}^p)=o(a^p)$
. Let
![]() $H_0/\langle c\rangle _H=\langle {\overline a}^{pj}\rangle \langle {\overline c}\rangle $
be the subgroup of
$H_0/\langle c\rangle _H=\langle {\overline a}^{pj}\rangle \langle {\overline c}\rangle $
be the subgroup of
![]() ${\overline H}$
with the largest order such that
${\overline H}$
with the largest order such that
![]() $\langle {\overline c}\rangle \le H_0/\langle c\rangle _H\subseteqq \langle {\overline a}^p\rangle \langle {\overline c}\rangle $
. Since
$\langle {\overline c}\rangle \le H_0/\langle c\rangle _H\subseteqq \langle {\overline a}^p\rangle \langle {\overline c}\rangle $
. Since
![]() $|{\overline H}|<|X|$
, by the induction hypothesis on
$|{\overline H}|<|X|$
, by the induction hypothesis on
![]() ${\overline H}$
, we know that
${\overline H}$
, we know that
![]() $H_0/\langle c\rangle _H=\langle {\overline a}^{pk}\rangle \langle {\overline c}\rangle $
for
$H_0/\langle c\rangle _H=\langle {\overline a}^{pk}\rangle \langle {\overline c}\rangle $
for
![]() $k\in \{1, 2, 3, 4\}$
, which implies
$k\in \{1, 2, 3, 4\}$
, which implies
![]() $\langle a^{pk}\rangle \langle c\rangle \langle c\rangle _H=\langle c\rangle \langle a^{pk}\rangle \langle c\rangle _H=\langle c\rangle \langle a^{pk}\rangle $
, giving
$\langle a^{pk}\rangle \langle c\rangle \langle c\rangle _H=\langle c\rangle \langle a^{pk}\rangle \langle c\rangle _H=\langle c\rangle \langle a^{pk}\rangle $
, giving
![]() $\langle a^{pk} \rangle \langle c\rangle \le H\le X$
. Therefore, we get
$\langle a^{pk} \rangle \langle c\rangle \le H\le X$
. Therefore, we get
![]() $a^{pk}\in M_X$
. Since
$a^{pk}\in M_X$
. Since
![]() $M_X=1$
and
$M_X=1$
and
![]() $a^p=a^s\ne 1$
, we get that the order of a is
$a^p=a^s\ne 1$
, we get that the order of a is
![]() $kp$
, where
$kp$
, where
![]() $ k\in \{2, 3, 4\}.$
Therefore, only the following three groups remain:
$ k\in \{2, 3, 4\}.$
Therefore, only the following three groups remain:
![]() $G=D_{2kp}$
, where
$G=D_{2kp}$
, where
![]() $k\in \{2, 3,4\}$
and p is a prime.
$k\in \{2, 3,4\}$
and p is a prime.
Step 3: Show that G cannot be
![]() $D_{2kp}$
, where
$D_{2kp}$
, where
![]() $k\in \{2, 3, 4\}$
and p is a prime, provided
$k\in \{2, 3, 4\}$
and p is a prime, provided
![]() $M_X=1$
. Suppose that
$M_X=1$
. Suppose that
![]() $G\cong D_{2kp}$
,
$G\cong D_{2kp}$
,
![]() $k\in \{2, 3, 4\}$
, recalling that
$k\in \{2, 3, 4\}$
, recalling that
![]() $H=\langle a^p, b\rangle \langle c\rangle $
,
$H=\langle a^p, b\rangle \langle c\rangle $
,
![]() $M_X=1$
,
$M_X=1$
,
![]() $\Omega =[X:\langle c\rangle ]$
and X has blocks of length
$\Omega =[X:\langle c\rangle ]$
and X has blocks of length
![]() $2k$
acting on
$2k$
acting on
![]() $\Omega $
. Moreover,
$\Omega $
. Moreover,
![]() $\langle a^p\rangle _X=1$
and there exists no nontrivial element
$\langle a^p\rangle _X=1$
and there exists no nontrivial element
![]() $a^j\in H$
such that
$a^j\in H$
such that
![]() $\langle a^j \rangle \langle c\rangle \le H$
. Here, we only give the proof for the case
$\langle a^j \rangle \langle c\rangle \le H$
. Here, we only give the proof for the case
![]() $k=4$
, that is,
$k=4$
, that is,
![]() $G\cong D_{8p}$
. The proof for other cases is similar but easier.
$G\cong D_{8p}$
. The proof for other cases is similar but easier.
Let
![]() $G=D_{8p}$
. If
$G=D_{8p}$
. If
![]() $p=2$
or
$p=2$
or
![]() $3$
, then
$3$
, then
![]() $G\cong D_{16}$
or
$G\cong D_{16}$
or
![]() $D_{24}$
, which can be directly excluded by Magma. So assume
$D_{24}$
, which can be directly excluded by Magma. So assume
![]() $p\ge 5$
. Set
$p\ge 5$
. Set
![]() $a_1=a^p$
and
$a_1=a^p$
and
![]() $a_2=a^4$
so that
$a_2=a^4$
so that
![]() $H=\langle a_1, b\rangle \langle c\rangle $
, where
$H=\langle a_1, b\rangle \langle c\rangle $
, where
![]() ${\mathrm {o}}(a_1)=4$
. Set
${\mathrm {o}}(a_1)=4$
. Set
![]() $C_0=\langle c\rangle _H$
and
$C_0=\langle c\rangle _H$
and
![]() $K=H_X$
. Then, H contains an element
$K=H_X$
. Then, H contains an element
![]() $a_1$
of order 4 having two orbits of length 4 on each block of length 8, where
$a_1$
of order 4 having two orbits of length 4 on each block of length 8, where
![]() $a_1\le K$
. Consider the action of
$a_1\le K$
. Consider the action of
![]() ${\overline H}:=H/C_0=\langle {\overline a}_1, {\overline b}\rangle \langle {\overline c}\rangle $
on the block containing the point
${\overline H}:=H/C_0=\langle {\overline a}_1, {\overline b}\rangle \langle {\overline c}\rangle $
on the block containing the point
![]() $\langle c \rangle $
, noting that
$\langle c \rangle $
, noting that
![]() $\langle {\overline c}\rangle $
is core-free. Recall that
$\langle {\overline c}\rangle $
is core-free. Recall that
![]() $\langle a_1\rangle _X=1$
. So
$\langle a_1\rangle _X=1$
. So
![]() $\langle {\overline a}_1,{\overline b}\rangle \cong D_8$
and
$\langle {\overline a}_1,{\overline b}\rangle \cong D_8$
and
![]() ${\overline H}\cong S_4$
. Moreover, we have
${\overline H}\cong S_4$
. Moreover, we have
![]() $N_{{\overline H}}(\langle {\overline c} \rangle )=\langle {\overline c}\rangle \rtimes \langle {\overline b}\rangle \cong D_6$
, by rechoosing b in
$N_{{\overline H}}(\langle {\overline c} \rangle )=\langle {\overline c}\rangle \rtimes \langle {\overline b}\rangle \cong D_6$
, by rechoosing b in
![]() $\langle a_1, b\rangle $
. Therefore,
$\langle a_1, b\rangle $
. Therefore,
![]() $L:=\langle c\rangle \langle b\rangle \le X$
.
$L:=\langle c\rangle \langle b\rangle \le X$
.
Now we turn to considering the imprimitive action of X on
![]() $\Omega _2:=[X:L]$
, which is of degree
$\Omega _2:=[X:L]$
, which is of degree
![]() $4p$
. Let
$4p$
. Let
![]() $K\cap \langle c\rangle =\langle c_1\rangle $
. Then every orbit of
$K\cap \langle c\rangle =\langle c_1\rangle $
. Then every orbit of
![]() $\langle c_1\rangle $
on
$\langle c_1\rangle $
on
![]() $\Omega _2$
is of length no more than 4. Observing the cycle decomposition of
$\Omega _2$
is of length no more than 4. Observing the cycle decomposition of
![]() $c_1L_X\in X/L_X$
on
$c_1L_X\in X/L_X$
on
![]() $\Omega _2$
, we know that
$\Omega _2$
, we know that
![]() $k_1:=|\langle c_1\rangle L_X/L_X| | 12$
. Therefore,
$k_1:=|\langle c_1\rangle L_X/L_X| | 12$
. Therefore,
![]() $c_1^{k_1}$
fixes all points in
$c_1^{k_1}$
fixes all points in
![]() $\Omega _2$
, which implies
$\Omega _2$
, which implies
![]() $c_1^{2k_1}$
fixes all points in
$c_1^{2k_1}$
fixes all points in
![]() $\Omega $
. Therefore,
$\Omega $
. Therefore,
![]() $c_1^{2k_1}=1$
(as
$c_1^{2k_1}=1$
(as
![]() $\langle c\rangle _X=1$
), that is,
$\langle c\rangle _X=1$
), that is,
![]() $|K\cap \langle c\rangle | | 2k_1$
and, in particular,
$|K\cap \langle c\rangle | | 2k_1$
and, in particular,
![]() $|K\cap C_0| | 2k_1$
. Moreover, since
$|K\cap C_0| | 2k_1$
. Moreover, since
![]() $\langle a_2K\rangle \lhd X/K\cong {\mathbb {Z}}_p\rtimes {\mathbb {Z}}_r$
for some
$\langle a_2K\rangle \lhd X/K\cong {\mathbb {Z}}_p\rtimes {\mathbb {Z}}_r$
for some
![]() $r | (p-1)$
, we know that
$r | (p-1)$
, we know that
![]() $K\rtimes \langle a_2\rangle \lhd X$
. Then,
$K\rtimes \langle a_2\rangle \lhd X$
. Then,
![]() $|K\cap C_0| | 24$
, as
$|K\cap C_0| | 24$
, as
![]() $k_1 | 12$
. Also,
$k_1 | 12$
. Also,
![]() $K/(K\cap C_0)\cong KC_0/C_0\lhd H/C_0\cong S_4$
. Since
$K/(K\cap C_0)\cong KC_0/C_0\lhd H/C_0\cong S_4$
. Since
![]() ${\overline a}_1\in KC_0/C_0$
, where
${\overline a}_1\in KC_0/C_0$
, where
![]() ${\mathrm {o}}({\overline a}_1)=4$
, and every normal subgroup of
${\mathrm {o}}({\overline a}_1)=4$
, and every normal subgroup of
![]() $S_4$
containing an element of order 4 must contain
$S_4$
containing an element of order 4 must contain
![]() $A_4$
, we know that
$A_4$
, we know that
![]() $K/(K\cap C_0)$
contains a characteristic subgroup
$K/(K\cap C_0)$
contains a characteristic subgroup
![]() $K_1/(K\cap C_0)\cong A_4$
.
$K_1/(K\cap C_0)\cong A_4$
.
We claim that
![]() $C_{K_1}(K\cap C_0)=K_1$
. Arguing by contradiction, assume that
$C_{K_1}(K\cap C_0)=K_1$
. Arguing by contradiction, assume that
![]() $K\cap C_0\nleqslant Z(K_1)$
. Then, as a quotient of
$K\cap C_0\nleqslant Z(K_1)$
. Then, as a quotient of
![]() $A_4$
, we have
$A_4$
, we have
![]() $3| |K_1/C_{K_1}(K\cap C_0)|.$
However, since
$3| |K_1/C_{K_1}(K\cap C_0)|.$
However, since
![]() $K_1/C_{K_1}(K\cap C_0)\le {\mathrm {Aut\,}}(K\cap C_0)$
,
$K_1/C_{K_1}(K\cap C_0)\le {\mathrm {Aut\,}}(K\cap C_0)$
,
![]() $K\cap C_0\le {\mathbb {Z}}_3\times {\mathbb {Z}}_8$
and using the cycle decomposition of the generator of
$K\cap C_0\le {\mathbb {Z}}_3\times {\mathbb {Z}}_8$
and using the cycle decomposition of the generator of
![]() $K\cap C_0$
, we get that
$K\cap C_0$
, we get that
![]() $K_1/C_{K_1}(K\cap C_0)$
contains no element of order 3, which implies a contradiction. Therefore,
$K_1/C_{K_1}(K\cap C_0)$
contains no element of order 3, which implies a contradiction. Therefore,
![]() $C_{K_1}(K\cap C_0)=K_1$
, that is,
$C_{K_1}(K\cap C_0)=K_1$
, that is,
![]() $(K\cap C_0)\le Z(K_1)$
.
$(K\cap C_0)\le Z(K_1)$
.
Since
![]() $K_1/(K\cap C_0)\cong A_4$
and
$K_1/(K\cap C_0)\cong A_4$
and
![]() $Z(A_4)=1$
, we get that
$Z(A_4)=1$
, we get that
![]() $K\cap C_0=Z(K_1) { \, \mathrm {char}\,} K_1\lhd X$
. Therefore,
$K\cap C_0=Z(K_1) { \, \mathrm {char}\,} K_1\lhd X$
. Therefore,
![]() $K\cap C_0\le 1$
(as
$K\cap C_0\le 1$
(as
![]() $\langle c\rangle _X=1$
) so that
$\langle c\rangle _X=1$
) so that
![]() $K\cong A_4$
or
$K\cong A_4$
or
![]() $S_4$
, which implies
$S_4$
, which implies
which contradicts
![]() $G_X=1$
again.
$G_X=1$
again.
To handle
![]() $X(Q)$
, we need the following result.
$X(Q)$
, we need the following result.
Lemma 3.2. Let
![]() $X=X(Q)=\langle a,b\rangle \langle c\rangle $
. If
$X=X(Q)=\langle a,b\rangle \langle c\rangle $
. If
![]() $\langle c\rangle _X=1$
, then
$\langle c\rangle _X=1$
, then
![]() $\langle a\rangle _X\ne 1$
.
$\langle a\rangle _X\ne 1$
.
Proof. Since
![]() $\langle c\rangle _X=1$
, by Proposition 2.3, we have
$\langle c\rangle _X=1$
, by Proposition 2.3, we have
![]() $m<|G|$
. So
$m<|G|$
. So
![]() $S:=G\cap G^{c}\ne 1$
, otherwise
$S:=G\cap G^{c}\ne 1$
, otherwise
![]() $|X|\ge |G|^2>|X|$
. Take a subgroup T of order a prime p of S. Since
$|X|\ge |G|^2>|X|$
. Take a subgroup T of order a prime p of S. Since
![]() $o(a^jb)=4$
for any integer j, we know
$o(a^jb)=4$
for any integer j, we know
![]() $T\le \langle a\rangle $
. Since S has a unique subgroup of order p, we get
$T\le \langle a\rangle $
. Since S has a unique subgroup of order p, we get
![]() $T^c=T$
, giving
$T^c=T$
, giving
![]() $T\lhd X$
and so
$T\lhd X$
and so
![]() $\langle a\rangle _X\ne 1$
, as desired.
$\langle a\rangle _X\ne 1$
, as desired.
Lemma 3.3. Theorem 1.1 holds, provided
![]() $G=Q$
and
$G=Q$
and
![]() $X=X(Q)$
.
$X=X(Q)$
.
Proof. Let X be a minimal counter-example. First, we claim that
![]() $\langle c\rangle $
is core-free. Arguing by contradiction, assume that
$\langle c\rangle $
is core-free. Arguing by contradiction, assume that
![]() $\langle c\rangle _X\ne 1$
. Consider
$\langle c\rangle _X\ne 1$
. Consider
![]() ${\overline X}=X/\langle c\rangle _X$
. The subgroup
${\overline X}=X/\langle c\rangle _X$
. The subgroup
![]() $\overline {M_1}$
of
$\overline {M_1}$
of
![]() ${\overline X}$
is chosen to have the largest order such that
${\overline X}$
is chosen to have the largest order such that
![]() $\langle {\overline c}\rangle \le \overline {M_1}\subseteqq \langle {\overline a}\rangle \langle {\overline c}\rangle $
. Since
$\langle {\overline c}\rangle \le \overline {M_1}\subseteqq \langle {\overline a}\rangle \langle {\overline c}\rangle $
. Since
![]() $|{\overline X}|<|X|$
and
$|{\overline X}|<|X|$
and
![]() ${\overline G}$
is a generalized quaternion group, by the minimality of
${\overline G}$
is a generalized quaternion group, by the minimality of
![]() ${\overline X}$
, we get
${\overline X}$
, we get
![]() $\overline {M_1}=\langle {\overline a}^i\rangle \langle {\overline c}\rangle $
, where
$\overline {M_1}=\langle {\overline a}^i\rangle \langle {\overline c}\rangle $
, where
![]() $i\in \{1, 2,3,4\}$
. This gives
$i\in \{1, 2,3,4\}$
. This gives
![]() $M=\langle a^k\rangle \langle c\rangle $
, where
$M=\langle a^k\rangle \langle c\rangle $
, where
![]() $k\in \{1, 2, 3, 4\}$
, which is a contradiction. Therefore,
$k\in \{1, 2, 3, 4\}$
, which is a contradiction. Therefore,
![]() $\langle c\rangle $
is core-free, that is,
$\langle c\rangle $
is core-free, that is,
![]() $\langle c\rangle _X=1$
.
$\langle c\rangle _X=1$
.
By Lemma 3.2,
![]() $\langle a\rangle _X\ne 1$
. If
$\langle a\rangle _X\ne 1$
. If
![]() $\langle a\rangle _X=\langle a\rangle $
, then
$\langle a\rangle _X=\langle a\rangle $
, then
![]() $X=(\langle a\rangle \rtimes \langle c\rangle ).\langle b\rangle $
, which implies
$X=(\langle a\rangle \rtimes \langle c\rangle ).\langle b\rangle $
, which implies
![]() $M=\langle a\rangle \langle c\rangle $
, and that is a contradiction. So
$M=\langle a\rangle \langle c\rangle $
, and that is a contradiction. So
![]() $\langle a\rangle _X<\langle a\rangle $
. Set
$\langle a\rangle _X<\langle a\rangle $
. Set
![]() ${\overline X}:=X/\langle a\rangle _X={\overline G} \langle {\overline c}\rangle $
and let the subgroup
${\overline X}:=X/\langle a\rangle _X={\overline G} \langle {\overline c}\rangle $
and let the subgroup
![]() $\overline {M_2}/\langle a\rangle _X$
of
$\overline {M_2}/\langle a\rangle _X$
of
![]() ${\overline X}$
be of the largest order such that
${\overline X}$
be of the largest order such that
![]() $\langle {\overline c}\rangle \le \overline {M_2}\subseteqq \langle {\overline a}\rangle \langle {\overline c}\rangle $
. If
$\langle {\overline c}\rangle \le \overline {M_2}\subseteqq \langle {\overline a}\rangle \langle {\overline c}\rangle $
. If
![]() $a^n\not \in \langle a\rangle _X$
, then
$a^n\not \in \langle a\rangle _X$
, then
![]() ${\overline G}$
is a generalized quaternion group; if
${\overline G}$
is a generalized quaternion group; if
![]() $a^n\in \langle a\rangle _X$
, then
$a^n\in \langle a\rangle _X$
, then
![]() ${\overline G}$
is a dihedral group. For the first case, by the minimality of
${\overline G}$
is a dihedral group. For the first case, by the minimality of
![]() ${\overline X}$
, and for the second case, by Lemma 3.1, we get
${\overline X}$
, and for the second case, by Lemma 3.1, we get
![]() $\overline {M_2}=\langle {\overline a}^i\rangle \langle {\overline c}\rangle $
, where
$\overline {M_2}=\langle {\overline a}^i\rangle \langle {\overline c}\rangle $
, where
![]() $i\in \{1, 2, 3, 4\}$
. Then,
$i\in \{1, 2, 3, 4\}$
. Then,
![]() $\langle a^i\rangle \langle c\rangle \langle a\rangle _X=\langle c\rangle \langle a^i\rangle \langle a\rangle _X$
. This gives
$\langle a^i\rangle \langle c\rangle \langle a\rangle _X=\langle c\rangle \langle a^i\rangle \langle a\rangle _X$
. This gives
![]() $(\langle a^i\rangle \langle a\rangle _X)\langle c\rangle \le X$
, which implies
$(\langle a^i\rangle \langle a\rangle _X)\langle c\rangle \le X$
, which implies
![]() $M=\langle a^k\rangle \langle c\rangle $
, where
$M=\langle a^k\rangle \langle c\rangle $
, where
![]() $k\in \{1, 2, 3, 4\}$
, which is a contradiction.
$k\in \{1, 2, 3, 4\}$
, which is a contradiction.
4 Proof of Theorem 1.2
The proof of Theorem 1.2 consists of the following three lemmas.
Lemma 4.1. Suppose that
![]() $G=Q,\,X=X(G),\,M=\langle a\rangle \langle c\rangle $
and
$G=Q,\,X=X(G),\,M=\langle a\rangle \langle c\rangle $
and
![]() $\langle c\rangle _X=1$
. If G is a
$\langle c\rangle _X=1$
. If G is a
![]() $2$
-group, then
$2$
-group, then
![]() $\langle a^2\rangle \lhd X$
.
$\langle a^2\rangle \lhd X$
.
Proof. Suppose that X is a minimal counter-example. Let
![]() $a_0$
be the involution of
$a_0$
be the involution of
![]() $\langle a\rangle $
. Since
$\langle a\rangle $
. Since
![]() $\langle c\rangle _X=1$
, by Lemma 3.2, we get
$\langle c\rangle _X=1$
, by Lemma 3.2, we get
![]() $\langle a\rangle _X\ne 1$
, which implies
$\langle a\rangle _X\ne 1$
, which implies
![]() $\langle a_0\rangle \lhd X$
, as G is a 2-group. Consider
$\langle a_0\rangle \lhd X$
, as G is a 2-group. Consider
![]() ${\overline X}=X/\langle a_0\rangle ={\overline G}\langle {\overline c}\rangle $
. Note that
${\overline X}=X/\langle a_0\rangle ={\overline G}\langle {\overline c}\rangle $
. Note that
![]() ${\overline G}$
is a dihedral group. Set
${\overline G}$
is a dihedral group. Set
![]() $\langle {\overline c} \rangle _X=(\langle a_0\rangle \times \langle c_0\rangle )/\langle a_0\rangle $
. Then,
$\langle {\overline c} \rangle _X=(\langle a_0\rangle \times \langle c_0\rangle )/\langle a_0\rangle $
. Then,
![]() $\langle c_0^2\rangle \lhd X$
, which implies
$\langle c_0^2\rangle \lhd X$
, which implies
![]() $c_0^2=1$
. If
$c_0^2=1$
. If
![]() $c_0=1$
, by Proposition 2.11, we get
$c_0=1$
, by Proposition 2.11, we get
![]() $\langle {\overline a}^2\rangle \lhd {\overline X}$
. Then,
$\langle {\overline a}^2\rangle \lhd {\overline X}$
. Then,
![]() $\langle a^2\rangle \lhd X$
is a contradiction, noting X is a minimal counter-example. Therefore,
$\langle a^2\rangle \lhd X$
is a contradiction, noting X is a minimal counter-example. Therefore,
![]() $o(c_0)=2$
. By using Proposition 2.11 on
$o(c_0)=2$
. By using Proposition 2.11 on
![]() $X/\langle a_0,c_0\rangle $
, we get
$X/\langle a_0,c_0\rangle $
, we get
![]() $(\langle a^2\rangle (\langle a_0\rangle \langle c_0\rangle ))/\langle a_0,c_0\rangle \lhd X/\langle a_0,c_0\rangle $
, that is,
$(\langle a^2\rangle (\langle a_0\rangle \langle c_0\rangle ))/\langle a_0,c_0\rangle \lhd X/\langle a_0,c_0\rangle $
, that is,
![]() $H:=\langle a^2\rangle \rtimes \langle c_0\rangle \lhd X$
. Then we continue the proof by the following two steps.
$H:=\langle a^2\rangle \rtimes \langle c_0\rangle \lhd X$
. Then we continue the proof by the following two steps.
Step 1: Show that X is a 2-group. In fact, noting that
![]() $\langle a^4\rangle =\mho _1(H){ \, \mathrm {char}\,} H\lhd X$
, relabel
$\langle a^4\rangle =\mho _1(H){ \, \mathrm {char}\,} H\lhd X$
, relabel
![]() ${\overline X}=X/\langle a^4\rangle $
, where we write
${\overline X}=X/\langle a^4\rangle $
, where we write
![]() $\langle {\overline c}\rangle _{{\overline X}}=\langle {\overline c}^i\rangle $
. Then,
$\langle {\overline c}\rangle _{{\overline X}}=\langle {\overline c}^i\rangle $
. Then,
![]() $\langle a^4\rangle \rtimes \langle c^i\rangle \lhd X$
. Let Q be the
$\langle a^4\rangle \rtimes \langle c^i\rangle \lhd X$
. Let Q be the
![]() $2'$
-Hall subgroup of
$2'$
-Hall subgroup of
![]() $\langle c^i\rangle $
. Since
$\langle c^i\rangle $
. Since
![]() ${\mathrm {Aut\,}}(\langle a^4\rangle )$
is a
${\mathrm {Aut\,}}(\langle a^4\rangle )$
is a
![]() $2$
-group, we know that
$2$
-group, we know that
![]() $[Q, a^4]=1$
and so
$[Q, a^4]=1$
and so
![]() $Q\lhd X$
, which contradicts
$Q\lhd X$
, which contradicts
![]() $\langle c\rangle _X=1$
. Therefore,
$\langle c\rangle _X=1$
. Therefore,
![]() $\langle c^i\rangle $
is also a
$\langle c^i\rangle $
is also a
![]() $2$
-group. Reset
$2$
-group. Reset
![]() ${\overline X}=X/\langle a^4\rangle \langle c^i\rangle ={\overline G} \langle {\overline c}\rangle $
. Now,
${\overline X}=X/\langle a^4\rangle \langle c^i\rangle ={\overline G} \langle {\overline c}\rangle $
. Now,
![]() $|{\overline G}|=8$
and so
$|{\overline G}|=8$
and so
![]() ${\overline G}\cong D_8$
(clearly,
${\overline G}\cong D_8$
(clearly,
![]() ${\overline G}$
cannot be
${\overline G}$
cannot be
![]() $Q_8$
). Since
$Q_8$
). Since
![]() $\langle {\overline c}\rangle _{{\overline X}}=1$
, we have
$\langle {\overline c}\rangle _{{\overline X}}=1$
, we have
![]() ${\mathrm {o}}({\overline c})| 4$
. Therefore,
${\mathrm {o}}({\overline c})| 4$
. Therefore,
![]() ${\overline X}$
is a
${\overline X}$
is a
![]() $2$
-group and so is X.
$2$
-group and so is X.
Step 2: Get a contradiction. Set
![]() $K:=\langle a_0\rangle \times \langle c_0\rangle \cong {\mathbb {Z}}_2^2$
. Consider the conjugacy of G on K. Since
$K:=\langle a_0\rangle \times \langle c_0\rangle \cong {\mathbb {Z}}_2^2$
. Consider the conjugacy of G on K. Since
![]() $\langle c_0\rangle \ntrianglelefteq X$
, we get
$\langle c_0\rangle \ntrianglelefteq X$
, we get
![]() $C_X(K)\cap G< G$
. Since G may be generated by some elements of the form
$C_X(K)\cap G< G$
. Since G may be generated by some elements of the form
![]() $a^jb$
, there exists an element
$a^jb$
, there exists an element
![]() $a^ib\in G\setminus C_G(K)$
, exchanging
$a^ib\in G\setminus C_G(K)$
, exchanging
![]() $c_0$
and
$c_0$
and
![]() $c_0a_0$
(as
$c_0a_0$
(as
![]() $(a^ib)^2=a_0$
). To make it easier to write, we write b instead of
$(a^ib)^2=a_0$
). To make it easier to write, we write b instead of
![]() $a^ib$
. Since
$a^ib$
. Since
![]() $X=GC=(\langle a\rangle \langle c\rangle ).\langle b\rangle $
, first we write
$X=GC=(\langle a\rangle \langle c\rangle ).\langle b\rangle $
, first we write
![]() $c^{b}=a^{s}c^{t}$
, where
$c^{b}=a^{s}c^{t}$
, where
![]() $t\neq 0$
. Note that
$t\neq 0$
. Note that
which implies
Then we have
If
![]() $t\neq 1$
, then
$t\neq 1$
, then
![]() $c_1^b\in \langle c^{t-1}\rangle ^b=\langle c^{t-1}\rangle $
, which contradicts
$c_1^b\in \langle c^{t-1}\rangle ^b=\langle c^{t-1}\rangle $
, which contradicts
![]() $c_1^b=a_1c_1$
. So
$c_1^b=a_1c_1$
. So
![]() $t=1$
, that is,
$t=1$
, that is,
![]() $c^b=a^sc$
. Second, we write
$c^b=a^sc$
. Second, we write
![]() $c^b=c^{t_1}a^{s_1}$
. With the same arguments as the above, we can get
$c^b=c^{t_1}a^{s_1}$
. With the same arguments as the above, we can get
![]() $t_1=1$
and
$t_1=1$
and
![]() $c^b=ca^{s_1}$
. Therefore, we have
$c^b=ca^{s_1}$
. Therefore, we have
![]() $a^{s}c=c^{b}=ca^{s_1},$
that is,
$a^{s}c=c^{b}=ca^{s_1},$
that is,
![]() $(a^{s})^{c}=a^{s_1}$
. Clearly,
$(a^{s})^{c}=a^{s_1}$
. Clearly,
![]() $\langle a^s\rangle =\langle a^{s_1}\rangle $
, which implies that c normalizes
$\langle a^s\rangle =\langle a^{s_1}\rangle $
, which implies that c normalizes
![]() $\langle a^{s}, b\rangle $
. Note that
$\langle a^{s}, b\rangle $
. Note that
which implies
![]() $b^a=ba^{-2}\in G_X$
. Thus,
$b^a=ba^{-2}\in G_X$
. Thus,
![]() $a^2\in G_X$
, which implies
$a^2\in G_X$
, which implies
![]() $\langle a^2\rangle \lhd X$
. This contradicts the minimality of X.
$\langle a^2\rangle \lhd X$
. This contradicts the minimality of X.
Lemma 4.2. Suppose that
![]() $G=Q,\,X=X(Q),\,M=\langle a\rangle \langle c\rangle $
and
$G=Q,\,X=X(Q),\,M=\langle a\rangle \langle c\rangle $
and
![]() $\langle c\rangle _X=1$
. Then,
$\langle c\rangle _X=1$
. Then,
![]() $\langle a^2\rangle \lhd~X$
.
$\langle a^2\rangle \lhd~X$
.
Proof. Take a minimal counter-example X and set
![]() ${\mathrm {o}}(c)=m$
,
${\mathrm {o}}(c)=m$
,
![]() ${\mathrm {o}}(a)=2n$
and
${\mathrm {o}}(a)=2n$
and
![]() $a_1:=a^n$
. By Lemma 4.1, we know that G is not a 2-group. We carry out the proof by the following three steps.
$a_1:=a^n$
. By Lemma 4.1, we know that G is not a 2-group. We carry out the proof by the following three steps.
Step 1: Show that the possible groups for G are
![]() $Q_{4p^k}$
, where p is an odd prime. By Lemma 3.2, let p be the maximal prime divisor of
$Q_{4p^k}$
, where p is an odd prime. By Lemma 3.2, let p be the maximal prime divisor of
![]() $|\langle a\rangle _X|$
and set
$|\langle a\rangle _X|$
and set
![]() $a_0=a^{{2n}/p}$
. Clearly,
$a_0=a^{{2n}/p}$
. Clearly,
![]() $a_0\in \langle a^2\rangle $
if p is odd. Consider
$a_0\in \langle a^2\rangle $
if p is odd. Consider
![]() ${\overline X}=X/\langle a_0\rangle ={\overline G}\langle {\overline c}\rangle $
and set
${\overline X}=X/\langle a_0\rangle ={\overline G}\langle {\overline c}\rangle $
and set
![]() $\langle {\overline c}\rangle _{{\overline X}}=\langle \overline {c_0}\rangle $
. Then depending on whether p is 2,
$\langle {\overline c}\rangle _{{\overline X}}=\langle \overline {c_0}\rangle $
. Then depending on whether p is 2,
![]() ${\overline G}$
is either a dihedral group or a generalized quaternion group. We claim that
${\overline G}$
is either a dihedral group or a generalized quaternion group. We claim that
![]() $1\ne \langle {\overline c}\rangle _{{\overline X}}=\langle \overline {c_0}\rangle \lneqq \langle {\overline c}\rangle $
. Arguing by contradiction, assume that
$1\ne \langle {\overline c}\rangle _{{\overline X}}=\langle \overline {c_0}\rangle \lneqq \langle {\overline c}\rangle $
. Arguing by contradiction, assume that
![]() $\langle {\overline c}\rangle _{{\overline X}}$
is either 1 or
$\langle {\overline c}\rangle _{{\overline X}}$
is either 1 or
![]() $\langle {\overline c}\rangle $
. Suppose that
$\langle {\overline c}\rangle $
. Suppose that
![]() $\langle {\overline c} \rangle _{{\overline X}}=1$
. Then by the minimality of X or Proposition 2.11, we get
$\langle {\overline c} \rangle _{{\overline X}}=1$
. Then by the minimality of X or Proposition 2.11, we get
![]() $\langle {\overline a}^2\rangle \lhd {\overline X}$
, which implies
$\langle {\overline a}^2\rangle \lhd {\overline X}$
, which implies
![]() $\langle a^2\rangle \langle a_0\rangle \lhd X$
. Since
$\langle a^2\rangle \langle a_0\rangle \lhd X$
. Since
![]() $\langle a^2\rangle \langle a_0\rangle $
is either
$\langle a^2\rangle \langle a_0\rangle $
is either
![]() $\langle a^2\rangle $
or
$\langle a^2\rangle $
or
![]() $\langle a\rangle $
, we get
$\langle a\rangle $
, we get
![]() $\langle a^2\rangle \lhd X$
, which is a contradiction. Suppose that
$\langle a^2\rangle \lhd X$
, which is a contradiction. Suppose that
![]() $\langle {\overline c}\rangle \lhd {\overline X}$
. Then,
$\langle {\overline c}\rangle \lhd {\overline X}$
. Then,
![]() ${\overline X}/C_{{\overline X}}(\langle {\overline c}\rangle )\le {\mathrm {Aut\,}}(\langle {\overline c}\rangle )$
which is abelian and so
${\overline X}/C_{{\overline X}}(\langle {\overline c}\rangle )\le {\mathrm {Aut\,}}(\langle {\overline c}\rangle )$
which is abelian and so
![]() ${\overline X}'\le C_{{\overline X}}(\langle {\overline c}\rangle )$
. Then,
${\overline X}'\le C_{{\overline X}}(\langle {\overline c}\rangle )$
. Then,
![]() ${\overline a}^2\in {\overline G}'\leq {\overline X}'\leq C_{{\overline X}}(\langle {\overline c}\rangle )$
, that is,
${\overline a}^2\in {\overline G}'\leq {\overline X}'\leq C_{{\overline X}}(\langle {\overline c}\rangle )$
, that is,
![]() $[a^2, c]\in \langle a_0\rangle $
, which implies
$[a^2, c]\in \langle a_0\rangle $
, which implies
![]() $\langle a^2, a_0\rangle \lhd X$
, and again we get
$\langle a^2, a_0\rangle \lhd X$
, and again we get
![]() $\langle a^2\rangle \lhd X$
, which is a contradiction. Therefore, we have
$\langle a^2\rangle \lhd X$
, which is a contradiction. Therefore, we have
![]() $1\ne \langle {\overline c}\rangle _{{\overline X}}=\langle \overline {c_0}\rangle \lneqq \langle {\overline c}\rangle $
.
$1\ne \langle {\overline c}\rangle _{{\overline X}}=\langle \overline {c_0}\rangle \lneqq \langle {\overline c}\rangle $
.
Consider
![]() $X_1=G\langle c_0\rangle <X$
. By the minimality of X, we get
$X_1=G\langle c_0\rangle <X$
. By the minimality of X, we get
![]() $\langle a^2\rangle \lhd X_1$
, which implies that
$\langle a^2\rangle \lhd X_1$
, which implies that
![]() $\langle c_0\rangle $
normalizes
$\langle c_0\rangle $
normalizes
![]() $\langle a^2\rangle $
. Reset
$\langle a^2\rangle $
. Reset
If
![]() ${\mathrm {o}}(a_0)<{\mathrm {o}}(c_0)$
, then
${\mathrm {o}}(a_0)<{\mathrm {o}}(c_0)$
, then
![]() $1\neq \langle c_0^j\rangle =Z(K)\lhd X$
is, for some j, a contradiction. Therefore,
$1\neq \langle c_0^j\rangle =Z(K)\lhd X$
is, for some j, a contradiction. Therefore,
![]() $1< {\mathrm {o}}(c_0)\le {\mathrm {o}}(a_0)$
. Note that K is either a Frobenius group or
$1< {\mathrm {o}}(c_0)\le {\mathrm {o}}(a_0)$
. Note that K is either a Frobenius group or
![]() ${\mathbb {Z}}_p^2$
. Thus, we have the following two cases.
${\mathbb {Z}}_p^2$
. Thus, we have the following two cases.
Case (1):
![]() $K=\langle a_0\rangle \rtimes \langle c_0\rangle \cong {\mathbb {Z}}_p\rtimes {\mathbb {Z}}_r$
, a Frobenius group, where
$K=\langle a_0\rangle \rtimes \langle c_0\rangle \cong {\mathbb {Z}}_p\rtimes {\mathbb {Z}}_r$
, a Frobenius group, where
![]() $r\ge 2$
and
$r\ge 2$
and
![]() $r\mid (p-1)$
. In this case, p is odd, which implies
$r\mid (p-1)$
. In this case, p is odd, which implies
![]() $a_0\in \langle a^2\rangle $
. Set
$a_0\in \langle a^2\rangle $
. Set
![]() ${\overline X}=X/K $
. By the minimality of X, we have
${\overline X}=X/K $
. By the minimality of X, we have
![]() $H/K=\langle {\overline a}^2\rangle \lhd {\overline X}$
, that is,
$H/K=\langle {\overline a}^2\rangle \lhd {\overline X}$
, that is,
![]() $H:=\langle a^2\rangle \rtimes \langle c_0\rangle \lhd X$
. Since
$H:=\langle a^2\rangle \rtimes \langle c_0\rangle \lhd X$
. Since
![]() $K\lhd X$
, we know that
$K\lhd X$
, we know that
![]() $\langle a^2\rangle /\langle a_0\rangle $
and
$\langle a^2\rangle /\langle a_0\rangle $
and
![]() $\langle c_0\rangle \langle a_0\rangle /\langle a_0\rangle $
are normal in
$\langle c_0\rangle \langle a_0\rangle /\langle a_0\rangle $
are normal in
![]() $H/\langle a_0\rangle $
. Then,
$H/\langle a_0\rangle $
. Then,
![]() $[a^2, c_0]\le \langle a_0\rangle $
. So
$[a^2, c_0]\le \langle a_0\rangle $
. So
Let
![]() $P\in {\mathrm {Syl}}_p(H)$
. Actually,
$P\in {\mathrm {Syl}}_p(H)$
. Actually,
![]() $P\leq \langle a^2\rangle $
. Then,
$P\leq \langle a^2\rangle $
. Then,
![]() $P{ \, \mathrm {char}\,} H\lhd X$
so that
$P{ \, \mathrm {char}\,} H\lhd X$
so that
![]() $P\leq \langle a\rangle _X$
. Clearly,
$P\leq \langle a\rangle _X$
. Clearly,
![]() $Z(H)=\langle a^{2p}\rangle $
. Then,
$Z(H)=\langle a^{2p}\rangle $
. Then,
![]() $\langle a^{2p}\rangle \leq \langle a\rangle _X$
. Noting that
$\langle a^{2p}\rangle \leq \langle a\rangle _X$
. Noting that
![]() $\langle a^{2p},P\rangle =\langle a^{2p}, a^{n/p^k}\rangle =\langle a^2\rangle $
, where
$\langle a^{2p},P\rangle =\langle a^{2p}, a^{n/p^k}\rangle =\langle a^2\rangle $
, where
![]() $p^k|| n$
, we get
$p^k|| n$
, we get
![]() $a^2\in \langle a \rangle _X$
, which is a contradiction.
$a^2\in \langle a \rangle _X$
, which is a contradiction.
Case (2):
![]() $K=\langle a_0\rangle \times \langle c_0\rangle \cong {\mathbb {Z}}_p^2$
. In this case, we claim that
$K=\langle a_0\rangle \times \langle c_0\rangle \cong {\mathbb {Z}}_p^2$
. In this case, we claim that
![]() $a_0\in \langle a^2\rangle $
. Arguing by contradiction, assume that
$a_0\in \langle a^2\rangle $
. Arguing by contradiction, assume that
![]() $a_0\notin \langle a^2\rangle $
. Then,
$a_0\notin \langle a^2\rangle $
. Then,
![]() $p=2$
and n is odd. In
$p=2$
and n is odd. In
![]() ${\overline X}$
, we know that
${\overline X}$
, we know that
![]() ${\overline H}\lhd {\overline X}$
, which implies
${\overline H}\lhd {\overline X}$
, which implies
![]() $\langle a_0\rangle H\lhd X$
. Noting
$\langle a_0\rangle H\lhd X$
. Noting
![]() $\langle a^2\rangle $
is a
$\langle a^2\rangle $
is a
![]() $2'$
-Hall subgroup of
$2'$
-Hall subgroup of
![]() $\langle a_0\rangle H$
, we have
$\langle a_0\rangle H$
, we have
![]() $\langle a^2\rangle { \, \mathrm {char}\,} \langle a_0\rangle H\lhd X,$
which implies
$\langle a^2\rangle { \, \mathrm {char}\,} \langle a_0\rangle H\lhd X,$
which implies
![]() $a^2\in \langle a\rangle _X$
, and that is a contradiction. Therefore,
$a^2\in \langle a\rangle _X$
, and that is a contradiction. Therefore,
![]() $a_0\in \langle a^2\rangle $
, which implies that p is an odd prime. With the same reason as that in Case (1), we have
$a_0\in \langle a^2\rangle $
, which implies that p is an odd prime. With the same reason as that in Case (1), we have
![]() $H\lhd X$
. Let
$H\lhd X$
. Let
![]() $H_1$
be the
$H_1$
be the
![]() $p'-$
Hall subgroup of H. We get that
$p'-$
Hall subgroup of H. We get that
![]() $H_1$
is also the
$H_1$
is also the
![]() $p'$
-Hall subgroup of
$p'$
-Hall subgroup of
![]() $\langle a^2\rangle $
as
$\langle a^2\rangle $
as
![]() ${\mathrm {o}}(c_0)=p$
. Then,
${\mathrm {o}}(c_0)=p$
. Then,
![]() $H_1{ \, \mathrm {char}\,} H\lhd X$
, which implies
$H_1{ \, \mathrm {char}\,} H\lhd X$
, which implies
![]() $H_1\leq \langle a\rangle _X$
. We claim that
$H_1\leq \langle a\rangle _X$
. We claim that
![]() $H_1=1$
. Arguing by contradiction, assume that
$H_1=1$
. Arguing by contradiction, assume that
![]() $H_1\neq 1$
. Then there exists an element
$H_1\neq 1$
. Then there exists an element
![]() $a_1$
of prime order q in
$a_1$
of prime order q in
![]() $H_1$
, where
$H_1$
, where
![]() $q < p$
, as p is maximal. Consider
$q < p$
, as p is maximal. Consider
![]() ${\overline X}:=X/\langle a_1\rangle ={\overline G}\langle c\rangle $
. Similarly, we have
${\overline X}:=X/\langle a_1\rangle ={\overline G}\langle c\rangle $
. Similarly, we have
![]() $1\ne \langle {\overline c}\rangle _{{\overline X}}:=\langle \overline {c_1}\rangle \lneqq {\overline C}$
and
$1\ne \langle {\overline c}\rangle _{{\overline X}}:=\langle \overline {c_1}\rangle \lneqq {\overline C}$
and
![]() $H_0:=\langle a^2\rangle \rtimes \langle c_1\rangle \lhd X$
. Let
$H_0:=\langle a^2\rangle \rtimes \langle c_1\rangle \lhd X$
. Let
![]() $P\in {\mathrm {Syl}}_p(H_0)$
. Then,
$P\in {\mathrm {Syl}}_p(H_0)$
. Then,
![]() $P{ \, \mathrm {char}\,} H$
and so
$P{ \, \mathrm {char}\,} H$
and so
![]() $P\lhd X$
, which implies
$P\lhd X$
, which implies
![]() $P\leq \langle a\rangle _X$
. Noting
$P\leq \langle a\rangle _X$
. Noting
![]() $\langle H_1,P\rangle =\langle a^2\rangle $
, we therefore get
$\langle H_1,P\rangle =\langle a^2\rangle $
, we therefore get
![]() $a^2\in \langle a\rangle _X$
, which is a contradiction. So
$a^2\in \langle a\rangle _X$
, which is a contradiction. So
![]() $H_1=1$
, which means that G is
$H_1=1$
, which means that G is
![]() $Q_{4p^k}$
where p is an odd prime as G is not a 2-group.
$Q_{4p^k}$
where p is an odd prime as G is not a 2-group.
Step 2: Show that the possible values of m are
![]() $pq^e$
for a prime q (may be equal to p) and an integer e. Arguing by contradiction, assume that
$pq^e$
for a prime q (may be equal to p) and an integer e. Arguing by contradiction, assume that
![]() $m=pq^em_1$
, where
$m=pq^em_1$
, where
![]() $m_1\ne 1$
and
$m_1\ne 1$
and
![]() $q\nmid m_1$
. Reset
$q\nmid m_1$
. Reset
![]() $a_1=a^n$
, the involution of
$a_1=a^n$
, the involution of
![]() $\langle a\rangle $
. Suppose that
$\langle a\rangle $
. Suppose that
![]() $a_1\in \langle a\rangle _X$
. Let
$a_1\in \langle a\rangle _X$
. Let
![]() $\langle a_1\rangle \rtimes \langle c_1\rangle $
be the core of
$\langle a_1\rangle \rtimes \langle c_1\rangle $
be the core of
![]() $\langle a_1,c\rangle $
in X, noting
$\langle a_1,c\rangle $
in X, noting
![]() $\langle a_1\rangle \lhd X$
. Since
$\langle a_1\rangle \lhd X$
. Since
![]() $\langle c_1^2\rangle \lhd X$
and
$\langle c_1^2\rangle \lhd X$
and
![]() $\langle c\rangle _X=1$
, we get
$\langle c\rangle _X=1$
, we get
![]() $c_1^2=1$
. Consider
$c_1^2=1$
. Consider
![]() ${\overline X}=X/\langle a_1\rangle \langle c_1\rangle $
. Since
${\overline X}=X/\langle a_1\rangle \langle c_1\rangle $
. Since
![]() ${\overline G}\cong D_{2p^k}$
is a dihedral group, by Proposition 2.11, we get
${\overline G}\cong D_{2p^k}$
is a dihedral group, by Proposition 2.11, we get
![]() $\langle {\overline a}^2\rangle =\langle {\overline a}\rangle \lhd {\overline X}$
, which implies
$\langle {\overline a}^2\rangle =\langle {\overline a}\rangle \lhd {\overline X}$
, which implies
![]() $\langle a\rangle \rtimes \langle c_1\rangle \lhd X$
. Then,
$\langle a\rangle \rtimes \langle c_1\rangle \lhd X$
. Then,
![]() $\langle a^2\rangle \lhd X$
is a contradiction. So in what follows (including Step 3), we assume that
$\langle a^2\rangle \lhd X$
is a contradiction. So in what follows (including Step 3), we assume that
![]() $a_1\notin \langle a\rangle _X$
, which implies that
$a_1\notin \langle a\rangle _X$
, which implies that
![]() $\langle a\rangle _X$
is a p-group.
$\langle a\rangle _X$
is a p-group.
Recall
![]() $H=\langle a^2\rangle \rtimes \langle c_0\rangle $
. Since
$H=\langle a^2\rangle \rtimes \langle c_0\rangle $
. Since
![]() $H\lhd X$
and
$H\lhd X$
and
![]() $b^2=a_1$
, we get
$b^2=a_1$
, we get
![]() ${\overline X}=X/H=\langle {\overline b}\rangle \langle {\overline c}\rangle $
and
${\overline X}=X/H=\langle {\overline b}\rangle \langle {\overline c}\rangle $
and
![]() $\langle {\overline b}\rangle \cong {\mathbb {Z}}_4$
. By considering the permutation representation of
$\langle {\overline b}\rangle \cong {\mathbb {Z}}_4$
. By considering the permutation representation of
![]() ${\overline X}$
on the cosets
${\overline X}$
on the cosets
![]() $[{\overline X}:\langle {\overline c}\rangle ]$
of size 4, we know that
$[{\overline X}:\langle {\overline c}\rangle ]$
of size 4, we know that
![]() $\langle {\overline c}^2\rangle \lhd {\overline X}$
. So
$\langle {\overline c}^2\rangle \lhd {\overline X}$
. So
![]() $\langle b, c^2, H\rangle \le X$
, that is,
$\langle b, c^2, H\rangle \le X$
, that is,
![]() $X_1:=\langle b, c^2, H\rangle =\langle a,b\rangle \langle c^2\rangle =G\langle c^2\rangle \le X$
.
$X_1:=\langle b, c^2, H\rangle =\langle a,b\rangle \langle c^2\rangle =G\langle c^2\rangle \le X$
.
First, suppose that m (=
![]() $o(c)$
) is even. Then,
$o(c)$
) is even. Then,
![]() $[X:X_1]=2$
. Let
$[X:X_1]=2$
. Let
![]() $\langle c_2\rangle $
be the Sylow 2-subgroup of
$\langle c_2\rangle $
be the Sylow 2-subgroup of
![]() $\langle c\rangle $
. By induction on
$\langle c\rangle $
. By induction on
![]() $X_1$
,
$X_1$
,
![]() $\langle a^2\rangle \lhd X_1$
and, in particular,
$\langle a^2\rangle \lhd X_1$
and, in particular,
![]() $\langle c^2\rangle $
normalizes
$\langle c^2\rangle $
normalizes
![]() $\langle a^2\rangle $
. By Proposition 2.6, we know that
$\langle a^2\rangle $
. By Proposition 2.6, we know that
![]() $\langle a^ib\rangle \langle c_2\rangle $
is a Sylow 2-subgroup of X for some i. Then we get
$\langle a^ib\rangle \langle c_2\rangle $
is a Sylow 2-subgroup of X for some i. Then we get
![]() $X_2:=H(\langle a^ib\rangle \langle c_2\rangle )=\langle a,b\rangle \langle c_0, c_2\rangle <X.$
By the minimality of X again,
$X_2:=H(\langle a^ib\rangle \langle c_2\rangle )=\langle a,b\rangle \langle c_0, c_2\rangle <X.$
By the minimality of X again,
![]() $\langle a^2\rangle \lhd X_2$
, which implies
$\langle a^2\rangle \lhd X_2$
, which implies
![]() $\langle c_2\rangle $
normalizes
$\langle c_2\rangle $
normalizes
![]() $\langle a^2\rangle $
. Since both
$\langle a^2\rangle $
. Since both
![]() $\langle c_2\rangle $
and
$\langle c_2\rangle $
and
![]() $\langle c^2\rangle $
normalize
$\langle c^2\rangle $
normalize
![]() $\langle a^2\rangle $
and
$\langle a^2\rangle $
and
![]() $\langle c_2, c^2\rangle =\langle c\rangle $
, we get
$\langle c_2, c^2\rangle =\langle c\rangle $
, we get
![]() $\langle a^2\rangle \lhd X$
, which is a contradiction.
$\langle a^2\rangle \lhd X$
, which is a contradiction.
Second, suppose that m is odd. Then both q and
![]() $m_1$
are odd, so that
$m_1$
are odd, so that
![]() $X=X_1=((\langle a^2\rangle \rtimes \langle c_0\rangle ).\langle c\rangle )\rtimes \langle b\rangle $
. By induction on
$X=X_1=((\langle a^2\rangle \rtimes \langle c_0\rangle ).\langle c\rangle )\rtimes \langle b\rangle $
. By induction on
![]() $X_3:=\langle H, c^{{m}/{m_1}}\rangle =\langle a,b\rangle \langle c_0, c^{{m}/{m_1}}\rangle <X$
and
$X_3:=\langle H, c^{{m}/{m_1}}\rangle =\langle a,b\rangle \langle c_0, c^{{m}/{m_1}}\rangle <X$
and
![]() $X_4:=\langle H, c^{{m}/{pq^e}}\rangle =\langle a,b\rangle \langle c^{{m}/{pq^e}}\rangle <X$
, we respectively get both
$X_4:=\langle H, c^{{m}/{pq^e}}\rangle =\langle a,b\rangle \langle c^{{m}/{pq^e}}\rangle <X$
, we respectively get both
![]() $\langle c^{{m}/{m_1}}\rangle $
and
$\langle c^{{m}/{m_1}}\rangle $
and
![]() $\langle c^{{m}/{pq^e}}\rangle $
normalize
$\langle c^{{m}/{pq^e}}\rangle $
normalize
![]() $\langle a^2\rangle $
. Noting
$\langle a^2\rangle $
. Noting
![]() $\langle c^{{m}/{m_1}},c^{{m}/{pq^e}}\rangle =\langle c\rangle $
, we get
$\langle c^{{m}/{m_1}},c^{{m}/{pq^e}}\rangle =\langle c\rangle $
, we get
![]() $\langle a^2\rangle \lhd X$
, which is a contradiction again.
$\langle a^2\rangle \lhd X$
, which is a contradiction again.
Step 3: Exclude the case
![]() $m=pq^e$
for a prime q and an integer e. Recall that
$m=pq^e$
for a prime q and an integer e. Recall that
![]() $a_1=a^n$
is the unique involution in G;
$a_1=a^n$
is the unique involution in G;
![]() $\langle a_0\rangle $
is a normal subgroup of order p in X;
$\langle a_0\rangle $
is a normal subgroup of order p in X;
![]() $K=\langle a_0\rangle \times \langle c_0\rangle =(\langle a_0\rangle \langle c\rangle )_X\cong {\mathbb {Z}}_p^2$
;
$K=\langle a_0\rangle \times \langle c_0\rangle =(\langle a_0\rangle \langle c\rangle )_X\cong {\mathbb {Z}}_p^2$
;
![]() $H=\langle a^2\rangle \rtimes \langle c_0\rangle \lhd X$
(by the induction hypothesis) and
$H=\langle a^2\rangle \rtimes \langle c_0\rangle \lhd X$
(by the induction hypothesis) and
![]() $X=((H.\langle c\rangle ).\langle a_1\rangle ).\langle b\rangle $
. Suppose that
$X=((H.\langle c\rangle ).\langle a_1\rangle ).\langle b\rangle $
. Suppose that
![]() $e=0$
. Then, consider
$e=0$
. Then, consider
![]() $S:=G\cap G^c$
. Since
$S:=G\cap G^c$
. Since
![]() $a_1\notin \langle a\rangle _X\leq S$
, we get that S is also a p-group,
$a_1\notin \langle a\rangle _X\leq S$
, we get that S is also a p-group,
![]() $S\leq \langle a\rangle _X$
and
$S\leq \langle a\rangle _X$
and
![]() $|S|\leq p^{k-1}$
. However, noting
$|S|\leq p^{k-1}$
. However, noting
![]() $16p^{k+1}\leq {|G||G^c|}/{|S|} < |X|=4p^{k+1}$
, we get a contradiction. Suppose that
$16p^{k+1}\leq {|G||G^c|}/{|S|} < |X|=4p^{k+1}$
, we get a contradiction. Suppose that
![]() $q=2$
and
$q=2$
and
![]() $e=1,2$
. Then using the same argument as above, we get a contradiction again. So in what follows, we assume that either q is odd and
$e=1,2$
. Then using the same argument as above, we get a contradiction again. So in what follows, we assume that either q is odd and
![]() $e\ge 1$
; or
$e\ge 1$
; or
![]() $q=2$
and
$q=2$
and
![]() $e\geq 3$
.
$e\geq 3$
.
Since H is a p-group and
![]() $\langle a^{2p}\rangle =\mho _1(H){ \, \mathrm {char}\,} H\lhd X$
, we get
$\langle a^{2p}\rangle =\mho _1(H){ \, \mathrm {char}\,} H\lhd X$
, we get
![]() $\langle a^{2p}\rangle \lhd X$
. Set
$\langle a^{2p}\rangle \lhd X$
. Set
![]() $X_5:=(H.\langle c^q\rangle )\rtimes \langle b\rangle =\langle a,b\rangle \langle c^q\rangle < X$
. By the induction hypothesis on
$X_5:=(H.\langle c^q\rangle )\rtimes \langle b\rangle =\langle a,b\rangle \langle c^q\rangle < X$
. By the induction hypothesis on
![]() $X_5$
, we get
$X_5$
, we get
![]() $\langle a^2\rangle \lhd X_5$
, that is,
$\langle a^2\rangle \lhd X_5$
, that is,
![]() $X_5=(\langle a^2\rangle \rtimes \langle c^q\rangle )\rtimes \langle b\rangle $
. Clearly,
$X_5=(\langle a^2\rangle \rtimes \langle c^q\rangle )\rtimes \langle b\rangle $
. Clearly,
![]() $\langle a^2\rangle =G'\le X_5'\le \langle a^2, c^q\rangle $
. So set
$\langle a^2\rangle =G'\le X_5'\le \langle a^2, c^q\rangle $
. So set
![]() $X_5'=\langle a^2, c_3\rangle $
for some
$X_5'=\langle a^2, c_3\rangle $
for some
![]() $c_3\in \langle c^q\rangle $
. By Proposition 2.2, both
$c_3\in \langle c^q\rangle $
. By Proposition 2.2, both
![]() $X/C_{X}(\langle a^{2p}\rangle )$
and
$X/C_{X}(\langle a^{2p}\rangle )$
and
![]() $X_5/C_{X_5}(\langle a^2\rangle )$
are abelian, which implies that
$X_5/C_{X_5}(\langle a^2\rangle )$
are abelian, which implies that
![]() $X'\leq C_X(\langle a^{2p}\rangle )$
and
$X'\leq C_X(\langle a^{2p}\rangle )$
and
![]() $X_5'\leq C_{X_5}(\langle a^2\rangle )$
. Then,
$X_5'\leq C_{X_5}(\langle a^2\rangle )$
. Then,
![]() $X_5'$
is abelian as
$X_5'$
is abelian as
![]() $\langle a^2\rangle \leq X_5'=\langle a^2, c_3\rangle $
. The
$\langle a^2\rangle \leq X_5'=\langle a^2, c_3\rangle $
. The
![]() $p'$
-Hall subgroup of
$p'$
-Hall subgroup of
![]() $X_5'$
is normal, which contradicts
$X_5'$
is normal, which contradicts
![]() $\langle c^q\rangle _{X_5}=1$
, meaning that
$\langle c^q\rangle _{X_5}=1$
, meaning that
![]() $X_5'$
is an abelian p-group. Set
$X_5'$
is an abelian p-group. Set
![]() $L:=H\rtimes \langle a_1\rangle =\langle a\rangle \langle c_0\rangle < X_5$
.
$L:=H\rtimes \langle a_1\rangle =\langle a\rangle \langle c_0\rangle < X_5$
.
We claim that
![]() $L\ntrianglelefteq X$
. Arguing by contradiction, assume that
$L\ntrianglelefteq X$
. Arguing by contradiction, assume that
![]() $L\lhd X$
. If H is abelian, then we get that either
$L\lhd X$
. If H is abelian, then we get that either
![]() $\langle a^2\rangle =Z(L){ \, \mathrm {char}\,} L\lhd X$
, which is a contradiction; or L is abelian, forcing
$\langle a^2\rangle =Z(L){ \, \mathrm {char}\,} L\lhd X$
, which is a contradiction; or L is abelian, forcing
![]() $\langle a_1\rangle { \, \mathrm {char}\,} L\lhd X$
, which is a contradiction again. Therefore, H is nonabelian. Note that
$\langle a_1\rangle { \, \mathrm {char}\,} L\lhd X$
, which is a contradiction again. Therefore, H is nonabelian. Note that
![]() $X_5'=\langle a^2, c_3\rangle $
for
$X_5'=\langle a^2, c_3\rangle $
for
![]() $c_3\in \langle c^q\rangle $
. If
$c_3\in \langle c^q\rangle $
. If
![]() $c_3\ne 1$
, then
$c_3\ne 1$
, then
![]() $c_0\in \langle c_3\rangle \leq X_5'$
as
$c_0\in \langle c_3\rangle \leq X_5'$
as
![]() ${\mathrm {o}}(c_0)=p$
, which implies that
${\mathrm {o}}(c_0)=p$
, which implies that
![]() $H=\langle a^2,c_0\rangle $
is abelian, which is a contradiction. Therefore,
$H=\langle a^2,c_0\rangle $
is abelian, which is a contradiction. Therefore,
![]() $X_5'=\langle a^2\rangle $
, which implies
$X_5'=\langle a^2\rangle $
, which implies
![]() $L=\langle a\rangle \rtimes \langle c_0\rangle $
. Note that
$L=\langle a\rangle \rtimes \langle c_0\rangle $
. Note that
![]() $\langle a_1\rangle { \, \mathrm {char}\,} L\lhd X$
. Thus, we get
$\langle a_1\rangle { \, \mathrm {char}\,} L\lhd X$
. Thus, we get
![]() $\langle a_1\rangle \lhd X$
, which contradicts
$\langle a_1\rangle \lhd X$
, which contradicts
![]() $a_1\notin \langle a\rangle _X$
. Therefore,
$a_1\notin \langle a\rangle _X$
. Therefore,
![]() $L\ntrianglelefteq X$
, which implies that
$L\ntrianglelefteq X$
, which implies that
![]() $\langle {\overline c}\rangle $
does not normalize
$\langle {\overline c}\rangle $
does not normalize
![]() $\langle \overline {a_1}\rangle $
in
$\langle \overline {a_1}\rangle $
in
![]() ${\overline X}=X/H$
.
${\overline X}=X/H$
.
In
![]() ${\overline X}=X/H=(\langle {\overline c}\rangle \rtimes \langle \overline {a_1}\rangle ).\langle {\overline b}\rangle $
, we get that either
${\overline X}=X/H=(\langle {\overline c}\rangle \rtimes \langle \overline {a_1}\rangle ).\langle {\overline b}\rangle $
, we get that either
![]() ${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
if q is odd; or
${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
if q is odd; or
![]() ${\overline c}^{\overline {a_1}}$
is either
${\overline c}^{\overline {a_1}}$
is either
![]() ${\overline c}^{-1}$
or
${\overline c}^{-1}$
or
![]() ${\overline c}^{\pm 1+2^{e-1}}$
if
${\overline c}^{\pm 1+2^{e-1}}$
if
![]() $q=2$
. Then we divide the proof into the following two cases.
$q=2$
. Then we divide the proof into the following two cases.
Case (1): q is an odd prime. In this case, q is odd. Since
![]() ${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
in
${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
in
![]() ${\overline X}=X/H$
, we get
${\overline X}=X/H$
, we get
![]() $\langle a^2, c^{qp}\rangle \leq X_5'\leq \langle a^2\rangle \langle c^q\rangle $
. Note that
$\langle a^2, c^{qp}\rangle \leq X_5'\leq \langle a^2\rangle \langle c^q\rangle $
. Note that
![]() $X_5'$
is the abelian p-group. Thus, either
$X_5'$
is the abelian p-group. Thus, either
![]() $q\ne p$
and
$q\ne p$
and
![]() $e=1$
; or
$e=1$
; or
![]() $q=p$
. Suppose that
$q=p$
. Suppose that
![]() $q\ne p$
and
$q\ne p$
and
![]() $e=1$
, that is,
$e=1$
, that is,
![]() ${\mathrm {o}}(c)=pq$
. Consider
${\mathrm {o}}(c)=pq$
. Consider
![]() $M=\langle a\rangle \langle c\rangle \lhd X$
. Then, by Proportion 2.4,
$M=\langle a\rangle \langle c\rangle \lhd X$
. Then, by Proportion 2.4,
![]() $M'$
is abelian. Note that
$M'$
is abelian. Note that
![]() $\langle c\rangle _X=1$
and
$\langle c\rangle _X=1$
and
![]() $G_X$
is the p-group. Thus,
$G_X$
is the p-group. Thus,
![]() $M'$
is an abelian p-group with the same argument as the case of
$M'$
is an abelian p-group with the same argument as the case of
![]() $X_5'$
. Noting that
$X_5'$
. Noting that
![]() $\langle a_1\rangle \langle c^p\rangle $
is the
$\langle a_1\rangle \langle c^p\rangle $
is the
![]() $p'$
-Hall subgroup of M, we get
$p'$
-Hall subgroup of M, we get
![]() $[a_1,c^p]\in \langle a_1\rangle \langle c^p\rangle \cap M'=1$
, which implies
$[a_1,c^p]\in \langle a_1\rangle \langle c^p\rangle \cap M'=1$
, which implies
![]() ${\overline c}^{\overline {a_1}}={\overline c}$
in
${\overline c}^{\overline {a_1}}={\overline c}$
in
![]() ${\overline X}=X/H$
, which is a contradiction. So in what follows, we assume that
${\overline X}=X/H$
, which is a contradiction. So in what follows, we assume that
![]() $q=p$
, that is,
$q=p$
, that is,
![]() ${\mathrm {o}}(c)=p^{e+1}$
. Note that
${\mathrm {o}}(c)=p^{e+1}$
. Note that
![]() ${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
in
${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
in
![]() ${\overline X}=X/H$
and
${\overline X}=X/H$
and
![]() $\langle a^2\rangle \leq X_5'$
. Thus,
$\langle a^2\rangle \leq X_5'$
. Thus,
![]() $X_5'$
is either
$X_5'$
is either
![]() $\langle a^2\rangle \langle c^p\rangle $
or
$\langle a^2\rangle \langle c^p\rangle $
or
![]() $\langle a^2\rangle $
, noting
$\langle a^2\rangle $
, noting
![]() $X_5'=\langle a^2\rangle $
only happens when
$X_5'=\langle a^2\rangle $
only happens when
![]() $e=1$
.
$e=1$
.
Suppose that
![]() $X_5'=\langle a^2\rangle \langle c^p\rangle $
. Since
$X_5'=\langle a^2\rangle \langle c^p\rangle $
. Since
![]() $H=\langle a^2\rangle \rtimes \langle c_0\rangle \leq X_5'\leq C_{X_5}(\langle a^2\rangle )$
, we get that
$H=\langle a^2\rangle \rtimes \langle c_0\rangle \leq X_5'\leq C_{X_5}(\langle a^2\rangle )$
, we get that
![]() $H=\langle a^2\rangle \times \langle c_0\rangle $
is abelian. Note that both
$H=\langle a^2\rangle \times \langle c_0\rangle $
is abelian. Note that both
![]() $\langle a^2\rangle $
and
$\langle a^2\rangle $
and
![]() $\langle c\rangle $
are p-groups and
$\langle c\rangle $
are p-groups and
![]() $X=(H.\langle c\rangle )\rtimes \langle b\rangle $
. Set
$X=(H.\langle c\rangle )\rtimes \langle b\rangle $
. Set
![]() $(a^2)^{c}=a^{2s}c_0^{t}$
and
$(a^2)^{c}=a^{2s}c_0^{t}$
and
![]() $c^b=a^{2u}c^v$
, where
$c^b=a^{2u}c^v$
, where
![]() $s\equiv 1\pmod {\ p}$
and
$s\equiv 1\pmod {\ p}$
and
![]() $p\nmid v$
. Then for an integer w, we get
$p\nmid v$
. Then for an integer w, we get
![]() $(a^2)^{c^w}=a^{2x_1}c_0^{wt}$
and
$(a^2)^{c^w}=a^{2x_1}c_0^{wt}$
and
![]() $c_0^b=a^{x_2}c_0^{v}$
for some integers
$c_0^b=a^{x_2}c_0^{v}$
for some integers
![]() $x_1$
and
$x_1$
and
![]() $x_2$
. Since
$x_2$
. Since
![]() $((a^2)^{c})^b=(a^{2s}c_0^{t})^b$
, there exist some integers x and y such that
$((a^2)^{c})^b=(a^{2s}c_0^{t})^b$
, there exist some integers x and y such that
which gives
![]() $t\equiv 0\,({\mathrm {mod }}\ p)$
. Then,
$t\equiv 0\,({\mathrm {mod }}\ p)$
. Then,
![]() $\langle a^2\rangle \lhd X$
, which is a contradiction.
$\langle a^2\rangle \lhd X$
, which is a contradiction.
Suppose that
![]() $X_5'=\langle a^2\rangle $
. Then,
$X_5'=\langle a^2\rangle $
. Then,
![]() ${\mathrm {o}}(c)=p^2$
,
${\mathrm {o}}(c)=p^2$
,
![]() $c_0=c^p$
and
$c_0=c^p$
and
![]() $X_5=\langle a,b\rangle \langle c_0\rangle $
. Since
$X_5=\langle a,b\rangle \langle c_0\rangle $
. Since
![]() $X_5'\leq G\leq X_5$
, we get that
$X_5'\leq G\leq X_5$
, we get that
![]() $\langle a_1\rangle { \, \mathrm {char}\,} G\lhd X$
, which implies that
$\langle a_1\rangle { \, \mathrm {char}\,} G\lhd X$
, which implies that
![]() $\langle a_1\rangle \leq Z(X_5)$
as
$\langle a_1\rangle \leq Z(X_5)$
as
![]() $a_1$
is an involution. Thus,
$a_1$
is an involution. Thus,
![]() $[c_0,a_1]=1$
. Set
$[c_0,a_1]=1$
. Set
![]() $c^{a_1}=a^xc^{-1+yp}$
as
$c^{a_1}=a^xc^{-1+yp}$
as
![]() ${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
in
${\overline c}^{\overline {a_1}}={\overline c}^{-1}$
in
![]() ${\overline X}=X/H$
. Then,
${\overline X}=X/H$
. Then,
which implies
![]() $(a^x)^{c^{1-yp}}=a^x$
. Then,
$(a^x)^{c^{1-yp}}=a^x$
. Then,
![]() $[a^x,c]=1$
. Note that
$[a^x,c]=1$
. Note that
![]() $c_0=c^p$
and
$c_0=c^p$
and
![]() $c_0=c_0^{a_1}=(c^{a_1})^p=a^{x}c^{-p}$
for some x. Thus, we get
$c_0=c_0^{a_1}=(c^{a_1})^p=a^{x}c^{-p}$
for some x. Thus, we get
![]() $c_0^2=1$
, which contradicts
$c_0^2=1$
, which contradicts
![]() ${\mathrm {o}}(c_0)=p$
.
${\mathrm {o}}(c_0)=p$
.
Case (2):
![]() $q=2$
. In this case, we know that
$q=2$
. In this case, we know that
![]() ${\mathrm {o}}(c)\ge 8p$
,
${\mathrm {o}}(c)\ge 8p$
,
![]() $X_5=(\langle a^2\rangle \langle c^2\rangle )\rtimes \langle b\rangle \lhd X$
and
$X_5=(\langle a^2\rangle \langle c^2\rangle )\rtimes \langle b\rangle \lhd X$
and
![]() ${\overline c}^{\overline {a_1}}$
is either
${\overline c}^{\overline {a_1}}$
is either
![]() ${\overline c}^{-1}$
or
${\overline c}^{-1}$
or
![]() ${\overline c}^{\pm 1+2^{e-1}}$
in
${\overline c}^{\pm 1+2^{e-1}}$
in
![]() $X/H$
.
$X/H$
.
We show
![]() ${\overline c}^{\overline {a_1}}={\overline c}^{1+2^{e-1}}$
in
${\overline c}^{\overline {a_1}}={\overline c}^{1+2^{e-1}}$
in
![]() $X/H$
. Since
$X/H$
. Since
![]() $\langle a^2\rangle \leq X_5'{ \, \mathrm {char}\,} X_5\lhd X$
and
$\langle a^2\rangle \leq X_5'{ \, \mathrm {char}\,} X_5\lhd X$
and
![]() $X_5'$
is the abelian p-group, we get
$X_5'$
is the abelian p-group, we get
![]() $X_5'=H$
, which implies that H is abelian. By Proposition 2.6, we get that both
$X_5'=H$
, which implies that H is abelian. By Proposition 2.6, we get that both
![]() $\langle a^{i_1}b\rangle \langle c^p\rangle $
and
$\langle a^{i_1}b\rangle \langle c^p\rangle $
and
![]() $\langle a^{i_2}b\rangle \langle c^{2p}\rangle $
are Sylow 2-subgroups of X and
$\langle a^{i_2}b\rangle \langle c^{2p}\rangle $
are Sylow 2-subgroups of X and
![]() $X_5$
for some
$X_5$
for some
![]() $i_1$
and
$i_1$
and
![]() $i_2$
, respectively. Then,
$i_2$
, respectively. Then,
![]() $[c^{2p},a^{i_2}b]\in X_5'\cap \langle c^{2p}\rangle \langle a^{i_2}b\rangle =1$
, which implies that
$[c^{2p},a^{i_2}b]\in X_5'\cap \langle c^{2p}\rangle \langle a^{i_2}b\rangle =1$
, which implies that
![]() $\langle c^{2p}\rangle \langle a^{i_2}b\rangle $
is abelian. So,
$\langle c^{2p}\rangle \langle a^{i_2}b\rangle $
is abelian. So,
![]() $[c^{2p},a_1]=1$
. Since
$[c^{2p},a_1]=1$
. Since
![]() ${\overline c}^{\overline {a_1}}$
is either
${\overline c}^{\overline {a_1}}$
is either
![]() ${\overline c}^{-1}$
or
${\overline c}^{-1}$
or
![]() ${\overline c}^{\pm 1+2^{e-1}}$
in
${\overline c}^{\pm 1+2^{e-1}}$
in
![]() $X/H$
, we get
$X/H$
, we get
![]() ${\overline c}^{\overline {a_1}}={\overline c}^{1+2^{e-1}}$
in
${\overline c}^{\overline {a_1}}={\overline c}^{1+2^{e-1}}$
in
![]() $X/H$
. Since
$X/H$
. Since
![]() $\langle a^{i_1}b\rangle \langle c^p\rangle =(\langle c^p\rangle \rtimes \langle a_1\rangle ).\langle a^{i_1}b\rangle $
, we know
$\langle a^{i_1}b\rangle \langle c^p\rangle =(\langle c^p\rangle \rtimes \langle a_1\rangle ).\langle a^{i_1}b\rangle $
, we know
![]() $(c^p)^{a_1}=c^{p+2^{e-1}p}$
, which implies
$(c^p)^{a_1}=c^{p+2^{e-1}p}$
, which implies
![]() $c^{2^{e-1}p}\in M'$
.
$c^{2^{e-1}p}\in M'$
.
Noting that
![]() $\langle c^p\rangle \rtimes \langle a_1\rangle =\langle a_1,c^p|a_1^2=c^{2^ep}=1,\,(c^p)^{a_1}=c^{(1+2^{e-1})p}\rangle $
, there are only three involutions in
$\langle c^p\rangle \rtimes \langle a_1\rangle =\langle a_1,c^p|a_1^2=c^{2^ep}=1,\,(c^p)^{a_1}=c^{(1+2^{e-1})p}\rangle $
, there are only three involutions in
![]() $\langle c^p\rangle \rtimes \langle a_1\rangle $
:
$\langle c^p\rangle \rtimes \langle a_1\rangle $
:
![]() $a_1,c^{2^{e-1}p}$
and
$a_1,c^{2^{e-1}p}$
and
![]() $a_1c^{2^{e-1}p}$
. By Proposition 2.5, we know that
$a_1c^{2^{e-1}p}$
. By Proposition 2.5, we know that
![]() $\langle c^{2p}\rangle \lhd \langle a^{i_1}b\rangle \langle c^q\rangle $
. Recall that
$\langle c^{2p}\rangle \lhd \langle a^{i_1}b\rangle \langle c^q\rangle $
. Recall that
![]() $M=\langle a\rangle \langle c\rangle $
. By Proposition 2.4,
$M=\langle a\rangle \langle c\rangle $
. By Proposition 2.4,
![]() $M'$
is abelian. Let
$M'$
is abelian. Let
![]() $M_2$
be the Sylow 2-subgroup of
$M_2$
be the Sylow 2-subgroup of
![]() $M'$
. Note that
$M'$
. Note that
![]() $M_2{ \, \mathrm {char}\,} M'{ \, \mathrm {char}\,} M\lhd X$
,
$M_2{ \, \mathrm {char}\,} M'{ \, \mathrm {char}\,} M\lhd X$
,
![]() $c^{2^{e-1}p}\in M'$
,
$c^{2^{e-1}p}\in M'$
,
![]() $\langle c\rangle _X=1$
and
$\langle c\rangle _X=1$
and
![]() $a_1$
is an involution. Thus, we get
$a_1$
is an involution. Thus, we get
![]() $M_2\cong {\mathbb {Z}}_2^2$
, that is,
$M_2\cong {\mathbb {Z}}_2^2$
, that is,
![]() $M_2=\langle c^{2^{e-1}p}\rangle \times \langle a_1\rangle $
. Consider
$M_2=\langle c^{2^{e-1}p}\rangle \times \langle a_1\rangle $
. Consider
![]() $HM_2\leq X$
. Since
$HM_2\leq X$
. Since
![]() $M_2\lhd X$
,
$M_2\lhd X$
,
![]() $H\lhd X$
,
$H\lhd X$
,
![]() $H\cap M_2=1$
and p is odd prime, we get
$H\cap M_2=1$
and p is odd prime, we get
![]() $HM_2=H\times M_2\lhd X$
, which implies that a normalizes
$HM_2=H\times M_2\lhd X$
, which implies that a normalizes
![]() $\langle c^{2^{e-1}p}\rangle $
. Recall that
$\langle c^{2^{e-1}p}\rangle $
. Recall that
![]() $\langle c^{2p}\rangle \langle a^{i_2}b\rangle $
is abelian. Then, we get
$\langle c^{2p}\rangle \langle a^{i_2}b\rangle $
is abelian. Then, we get
![]() $[c^{2p},a^{i_2}b]=1$
. Since
$[c^{2p},a^{i_2}b]=1$
. Since
![]() $\langle c^{2^{e-1}p}\rangle \leq \langle c^{2p}\rangle $
, we get that b also normalizes
$\langle c^{2^{e-1}p}\rangle \leq \langle c^{2p}\rangle $
, we get that b also normalizes
![]() $\langle c^{2^{e-1}p}\rangle $
. Since
$\langle c^{2^{e-1}p}\rangle $
. Since
![]() $X=\langle a,b,c\rangle $
, we get
$X=\langle a,b,c\rangle $
, we get
![]() $\langle c^{2^{e-1}p}\rangle \lhd X$
, which is a contradiction.
$\langle c^{2^{e-1}p}\rangle \lhd X$
, which is a contradiction.
Lemma 4.3. Theorem 1.2 holds.
Proof. (1) Suppose that
![]() $\langle c\rangle _X=1$
. For the five cases of Theorem 1.1, we know that
$\langle c\rangle _X=1$
. For the five cases of Theorem 1.1, we know that
![]() $M_X$
is
$M_X$
is
![]() $M,\,\langle a^2\rangle \langle c^2\rangle ,\,\langle a^2\rangle \langle c^3\rangle ,\,\langle a^3\rangle \langle c^4\rangle $
or
$M,\,\langle a^2\rangle \langle c^2\rangle ,\,\langle a^2\rangle \langle c^3\rangle ,\,\langle a^3\rangle \langle c^4\rangle $
or
![]() $\langle a^4\rangle \langle c^3\rangle $
. Set
$\langle a^4\rangle \langle c^3\rangle $
. Set
![]() $M_X=\langle a^i\rangle \langle c^j\rangle $
, which is one of the above five cases, and
$M_X=\langle a^i\rangle \langle c^j\rangle $
, which is one of the above five cases, and
![]() $X_1=GM_X=\langle a,b\rangle \langle c^j\rangle $
, where
$X_1=GM_X=\langle a,b\rangle \langle c^j\rangle $
, where
![]() $G\in \{ Q,\,D\}$
. Then,
$G\in \{ Q,\,D\}$
. Then,
![]() $\langle c^j\rangle _{X_1}=1$
. By Proposition 2.11 and Lemma 4.2, we get
$\langle c^j\rangle _{X_1}=1$
. By Proposition 2.11 and Lemma 4.2, we get
![]() $\langle a^2\rangle \lhd X_1$
, that is,
$\langle a^2\rangle \lhd X_1$
, that is,
![]() $c^j$
normalizes
$c^j$
normalizes
![]() $\langle a^2\rangle $
, and so
$\langle a^2\rangle $
, and so
![]() $M_X\cap \langle a^2\rangle \lhd M_X$
.
$M_X\cap \langle a^2\rangle \lhd M_X$
.
(2) Suppose
![]() $\langle c_1\rangle :=\langle c\rangle _X\ne 1$
. Then, by Proposition 2.2, we get
$\langle c_1\rangle :=\langle c\rangle _X\ne 1$
. Then, by Proposition 2.2, we get
![]() $\langle c\rangle \leq C_X(\langle c_1\rangle )\lhd X$
and
$\langle c\rangle \leq C_X(\langle c_1\rangle )\lhd X$
and
![]() ${\overline X}=X/C_X(\langle c_1\rangle )=\langle {\overline a},{\overline b}\rangle $
is abelian. This implies
${\overline X}=X/C_X(\langle c_1\rangle )=\langle {\overline a},{\overline b}\rangle $
is abelian. This implies
![]() $\langle {\overline a},{\overline b}\rangle \lessapprox {\mathbb {Z}}_2\times {\mathbb {Z}}_2$
or
$\langle {\overline a},{\overline b}\rangle \lessapprox {\mathbb {Z}}_2\times {\mathbb {Z}}_2$
or
![]() ${\mathbb {Z}}_4$
. Therefore,
${\mathbb {Z}}_4$
. Therefore,
![]() $\langle a^2, c\rangle \leq C_X(\langle c_1\rangle )$
. Therefore,
$\langle a^2, c\rangle \leq C_X(\langle c_1\rangle )$
. Therefore,
![]() $|X:C_X(\langle c_1\rangle )|\le 4$
.
$|X:C_X(\langle c_1\rangle )|\le 4$
.
To classify skew product groups of generalized quaternion groups, the following lemma is useful.
Lemma 4.4. Let
![]() $G\in \{Q, D\}$
and
$G\in \{Q, D\}$
and
![]() $X=X(G)$
. If
$X=X(G)$
. If
![]() $\langle c\rangle _X=1$
and
$\langle c\rangle _X=1$
and
![]() $\langle a\rangle \lhd X$
, then
$\langle a\rangle \lhd X$
, then
![]() $G\lhd X$
.
$G\lhd X$
.
Proof.
![]() $X=(\langle a\rangle \rtimes \langle c\rangle ).\langle b\rangle ,$
and so we may write
$X=(\langle a\rangle \rtimes \langle c\rangle ).\langle b\rangle ,$
and so we may write
![]() $a^c=a^i$
and
$a^c=a^i$
and
![]() $c^b=a^kc^j$
. If
$c^b=a^kc^j$
. If
![]() $j=1$
, then
$j=1$
, then
![]() $G\lhd X$
. So assume
$G\lhd X$
. So assume
![]() $j\ne 1$
. Since
$j\ne 1$
. Since
![]() $b^2=a^n$
(
$b^2=a^n$
(
![]() $o(a^n)=1$
or 2 if
$o(a^n)=1$
or 2 if
![]() $G=D$
or
$G=D$
or
![]() $G=Q$
, respectively) and
$G=Q$
, respectively) and
![]() $\langle a^n\rangle \lhd X$
, we get
$\langle a^n\rangle \lhd X$
, we get
![]() $a^n\in Z(X)$
, which implies
$a^n\in Z(X)$
, which implies
![]() $c=c^{b^2}$
. Then,
$c=c^{b^2}$
. Then,
that is,
![]() $c^{1-j}=(a^kc^j)^{j-1}=(c^{j-1})^b,$
so that b normalizes
$c^{1-j}=(a^kc^j)^{j-1}=(c^{j-1})^b,$
so that b normalizes
![]() $\langle c^{1-j}\rangle $
. Since
$\langle c^{1-j}\rangle $
. Since
![]() ${\overline X}=X/C_X(\langle a\rangle )\le {\mathrm {Aut\,}}(\langle a\rangle )$
, which is abelian, we get
${\overline X}=X/C_X(\langle a\rangle )\le {\mathrm {Aut\,}}(\langle a\rangle )$
, which is abelian, we get
![]() ${\overline c} ={\overline c}^{{\overline b}}={\overline c}^j$
, that is,
${\overline c} ={\overline c}^{{\overline b}}={\overline c}^j$
, that is,
![]() $c^{1-j}\le C_X(\langle a\rangle )$
so that
$c^{1-j}\le C_X(\langle a\rangle )$
so that
![]() $[c^{1-j}, a]=1$
. Thus, we get
$[c^{1-j}, a]=1$
. Thus, we get
![]() $\langle c^{1-j}\rangle \lhd X$
. It follows from
$\langle c^{1-j}\rangle \lhd X$
. It follows from
![]() $\langle c\rangle _X=1$
that
$\langle c\rangle _X=1$
that
![]() $j=1$
, which is a contradiction.
$j=1$
, which is a contradiction.
5 Proof of Theorem 1.3
To prove Theorem 1.3, set
![]() $R:=\{a^{2n}=c^m=1,\,b^2=a^n,\,a^b=a^{-1}\}.$
Recall that M is the subgroup of the largest order in
$R:=\{a^{2n}=c^m=1,\,b^2=a^n,\,a^b=a^{-1}\}.$
Recall that M is the subgroup of the largest order in
![]() $X(Q)$
such that
$X(Q)$
such that
![]() $\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
. By Theorems 1.1 and 1.2, we get that
$\langle c\rangle \le M\subseteqq \langle a\rangle \langle c\rangle $
. By Theorems 1.1 and 1.2, we get that
![]() $X(Q)$
, where
$X(Q)$
, where
![]() $\langle c\rangle _{X(Q)}=1$
has the forms listed in Table 3. Then, we deal with these five cases in the following five subsections, separately.
$\langle c\rangle _{X(Q)}=1$
has the forms listed in Table 3. Then, we deal with these five cases in the following five subsections, separately.
Table 3 The forms of
![]() $\langle c\rangle _{X(Q)}=1$
.
$\langle c\rangle _{X(Q)}=1$
.

Let
![]() $A=G.\langle t\rangle $
, where
$A=G.\langle t\rangle $
, where
![]() $G\lhd A$
, be a group and
$G\lhd A$
, be a group and
![]() $t^l=g\in G$
. Then, t induces an automorphism
$t^l=g\in G$
. Then, t induces an automorphism
![]() $\tau $
of G by conjugacy. Recall that by the cyclic extension theory of groups, this extension is valid if and only if
$\tau $
of G by conjugacy. Recall that by the cyclic extension theory of groups, this extension is valid if and only if
5.1
 $M=\langle a\rangle \langle c\rangle $
$M=\langle a\rangle \langle c\rangle $
Lemma 5.1. Suppose that
![]() $X=X(Q)$
,
$X=X(Q)$
,
![]() $M=\langle a\rangle \langle c\rangle $
and
$M=\langle a\rangle \langle c\rangle $
and
![]() $\langle c\rangle _X=1$
. Then,
$\langle c\rangle _X=1$
. Then,
where
 $$ \begin{align*} r^m\equiv r^{t-1}&\equiv r^{v-1}\equiv1\,({\mathrm{mod}}\ n), t^2\equiv 1\,({\mathrm{mod}}\ m),\\ 2s\sum_{l=1}^tr^{l}+2sr&\equiv 2sr+2s\sum_{l=1}^{v} r^l-u\sum_{l=1}^tr^l+ur\equiv 2(1-r)\,({\mathrm{mod}}\ 2n),\\ 2s\sum_{l=1}^{w}r^l&\equiv u\sum_{l=1}^{w}\bigg(1-s\bigg(\sum_{l=1}^tr^l+r\bigg)\bigg)^l\equiv 0\,({\mathrm{mod}}\ 2n) \end{align*} $$
$$ \begin{align*} r^m\equiv r^{t-1}&\equiv r^{v-1}\equiv1\,({\mathrm{mod}}\ n), t^2\equiv 1\,({\mathrm{mod}}\ m),\\ 2s\sum_{l=1}^tr^{l}+2sr&\equiv 2sr+2s\sum_{l=1}^{v} r^l-u\sum_{l=1}^tr^l+ur\equiv 2(1-r)\,({\mathrm{mod}}\ 2n),\\ 2s\sum_{l=1}^{w}r^l&\equiv u\sum_{l=1}^{w}\bigg(1-s\bigg(\sum_{l=1}^tr^l+r\bigg)\bigg)^l\equiv 0\,({\mathrm{mod}}\ 2n) \end{align*} $$
only when
![]() $w\equiv 0\,({\mathrm {mod}}\ m)$
, and moreover, if
$w\equiv 0\,({\mathrm {mod}}\ m)$
, and moreover, if
![]() $2\mid n$
, then
$2\mid n$
, then
![]() $u(\sum _{l=0}^{v-1}r^l-1)\equiv 0\,({\mathrm {mod}}\ 2n)$
and
$u(\sum _{l=0}^{v-1}r^l-1)\equiv 0\,({\mathrm {mod}}\ 2n)$
and
![]() $v^2\equiv 1\,({\mathrm {mod}}\ m)$
; if
$v^2\equiv 1\,({\mathrm {mod}}\ m)$
; if
![]() $2\nmid n$
, then
$2\nmid n$
, then
![]() $u\sum _{l=1}^vr^l-ur\equiv 2sr+(n-1)(1-r)\,({\mathrm {mod}}\ 2n)$
and
$u\sum _{l=1}^vr^l-ur\equiv 2sr+(n-1)(1-r)\,({\mathrm {mod}}\ 2n)$
and
![]() $v^2\equiv t\,({\mathrm {mod}}\ m)$
; and if
$v^2\equiv t\,({\mathrm {mod}}\ m)$
; and if
![]() $t\ne 1$
, then u is even.
$t\ne 1$
, then u is even.
Proof. Noting
![]() $\langle a^2\rangle \lhd X$
and
$\langle a^2\rangle \lhd X$
and
![]() $M=\langle a\rangle \langle c\rangle \le X$
, the group X may be obtained by three cyclic extensions of groups in order:
$M=\langle a\rangle \langle c\rangle \le X$
, the group X may be obtained by three cyclic extensions of groups in order:
So X has presentation as in (5-1). Then, we should determine the parameters
![]() $r,s,t,u$
and v by analysing three extensions.
$r,s,t,u$
and v by analysing three extensions.
-
(1)
 $\langle a^2\rangle \rtimes \langle c\rangle $
, where
$\langle a^2\rangle \rtimes \langle c\rangle $
, where
 $(a^2)^c=a^{2r}$
. Set
$(a^2)^c=a^{2r}$
. Set
 $\pi _1\in {\mathrm {Aut\,}}(\langle a^2\rangle )$
such that
$\pi _1\in {\mathrm {Aut\,}}(\langle a^2\rangle )$
such that
 $\pi _1(a^2)=a^{2r}$
. Since
$\pi _1(a^2)=a^{2r}$
. Since
 $\pi _1(\langle a^2\rangle )=\langle a^{2r}\rangle $
, we get
$\pi _1(\langle a^2\rangle )=\langle a^{2r}\rangle $
, we get
 $(r,n)=1$
. As mentioned before, this extension is valid if and only if
$(r,n)=1$
. As mentioned before, this extension is valid if and only if
 ${\mathrm {o}}(\pi _1(a^{2}))={\mathrm {o}}(a^2)$
and
${\mathrm {o}}(\pi _1(a^{2}))={\mathrm {o}}(a^2)$
and
 $\pi _1^{m}=1$
, that is, (5-2)
$\pi _1^{m}=1$
, that is, (5-2) $$ \begin{align} r^m-1\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} r^m-1\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
-
(2)
 $(\langle a^2\rangle \rtimes \langle c\rangle ).\langle a\rangle $
, where
$(\langle a^2\rangle \rtimes \langle c\rangle ).\langle a\rangle $
, where
 $c^a=a^{2s}c^t$
. Set
$c^a=a^{2s}c^t$
. Set
 $\pi _2\in {\mathrm {Aut\,}}(\langle a^2\rangle \rtimes \langle c\rangle )$
:
$\pi _2\in {\mathrm {Aut\,}}(\langle a^2\rangle \rtimes \langle c\rangle )$
:
 $a^2\to a^2$
and
$a^2\to a^2$
and
 $c\to a^{2s}c^t$
. Since
$c\to a^{2s}c^t$
. Since
 $\pi _2(\langle a^2,c\rangle )=\langle a^2,a^{2s}c^t\rangle $
, we get
$\pi _2(\langle a^2,c\rangle )=\langle a^2,a^{2s}c^t\rangle $
, we get
 $(t,m)=1$
. This extension is valid if and only if the following three equalities hold:
$(t,m)=1$
. This extension is valid if and only if the following three equalities hold:-
(i)
 $\pi _2$
preserves
$\pi _2$
preserves
 $(a^2)^c=a^{2r}$
: (5-3)
$(a^2)^c=a^{2r}$
: (5-3) $$ \begin{align} r^{t-1}-1\equiv 0\,({\mathrm{mod}}\ n); \end{align} $$
$$ \begin{align} r^{t-1}-1\equiv 0\,({\mathrm{mod}}\ n); \end{align} $$
-
(ii)
 ${\mathrm {o}}(\pi _2(c))=m$
: for any integer i, Note that
${\mathrm {o}}(\pi _2(c))=m$
: for any integer i, Note that $$ \begin{align*}(a^{2s}c^t)^i=c^{it}(a^{2s})^{c^{it}}\cdots(a^{2s})^{c^t} =c^{it}a^{2s\sum_{l=1}^ir^{tl}}=c^{ti}a^{2s\sum_{l=1}^i r^{l}}.\end{align*} $$
$$ \begin{align*}(a^{2s}c^t)^i=c^{it}(a^{2s})^{c^{it}}\cdots(a^{2s})^{c^t} =c^{it}a^{2s\sum_{l=1}^ir^{tl}}=c^{ti}a^{2s\sum_{l=1}^i r^{l}}.\end{align*} $$
 $c^{it}\ne 1$
for any
$c^{it}\ne 1$
for any
 $0<i<m$
. Thus, we only show
$0<i<m$
. Thus, we only show
 $(a^{2s}c^t)^m=1$
, that is,(5-4)
$(a^{2s}c^t)^m=1$
, that is,(5-4) $$ \begin{align} s\sum_{l=1}^{m} r^{l}\equiv 0\,({\mathrm{mod}}\ n); \end{align} $$
$$ \begin{align} s\sum_{l=1}^{m} r^{l}\equiv 0\,({\mathrm{mod}}\ n); \end{align} $$
-
(iii)
 $\pi _2^2={\mathrm {Inn}}(a^2)$
: that is,
$\pi _2^2={\mathrm {Inn}}(a^2)$
: that is, $$ \begin{align*}ca^{2-2r}={\mathrm{Inn}}(a^2)(c)=\pi_2^2(c)=(a^{2s}c^t)^{a}=a^{2s}(a^{2s}c^t)^t =c^{t^2}a^{2sr+2s\sum_{l=1}^{t} r^{l}},\end{align*} $$
(5-5)
$$ \begin{align*}ca^{2-2r}={\mathrm{Inn}}(a^2)(c)=\pi_2^2(c)=(a^{2s}c^t)^{a}=a^{2s}(a^{2s}c^t)^t =c^{t^2}a^{2sr+2s\sum_{l=1}^{t} r^{l}},\end{align*} $$
(5-5) $$ \begin{align} t^2-1\equiv 0\,({\mathrm{mod}}\ m) \quad \mathrm{and}\quad s\sum_{l=1}^{t}r^{l}+rs+r-1\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} t^2-1\equiv 0\,({\mathrm{mod}}\ m) \quad \mathrm{and}\quad s\sum_{l=1}^{t}r^{l}+rs+r-1\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
-
-
(3)
 $((\langle a^2\rangle \rtimes \langle c\rangle ).\langle a\rangle ).\langle b\rangle $
, where
$((\langle a^2\rangle \rtimes \langle c\rangle ).\langle a\rangle ).\langle b\rangle $
, where
 $c^b=a^uc^v$
. Set
$c^b=a^uc^v$
. Set
 $\pi _3\in {\mathrm {Aut\,}}((\langle a^2\rangle \rtimes \langle c\rangle ).\langle a\rangle ):\, a\to a^{-1}$
and
$\pi _3\in {\mathrm {Aut\,}}((\langle a^2\rangle \rtimes \langle c\rangle ).\langle a\rangle ):\, a\to a^{-1}$
and
 $c\to a^uc^v$
, where
$c\to a^uc^v$
, where
 $(v,m)=1$
. We divide the proof into two cases according to the parity of u, separately.
$(v,m)=1$
. We divide the proof into two cases according to the parity of u, separately.
Case 1: u is even.
-
(i)
 $\pi _3$
preserves
$\pi _3$
preserves
 $(a^2)^c=a^{2r}$
: (5-6)
$(a^2)^c=a^{2r}$
: (5-6) $$ \begin{align} r^{v-1}-1\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} r^{v-1}-1\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
-
(ii)
 ${\mathrm {o}}(\pi _3(c))=m$
: that is,
${\mathrm {o}}(\pi _3(c))=m$
: that is, $$ \begin{align*}1=(a^uc^v)^m=c^{vm}a^{u\sum_{l=1}^{m}r^l},\end{align*} $$
(5-7)
$$ \begin{align*}1=(a^uc^v)^m=c^{vm}a^{u\sum_{l=1}^{m}r^l},\end{align*} $$
(5-7) $$ \begin{align} u\sum_{l=1}^{m}r^l\equiv0\,({\mathrm{mod}}\ 2n). \end{align} $$
$$ \begin{align} u\sum_{l=1}^{m}r^l\equiv0\,({\mathrm{mod}}\ 2n). \end{align} $$
-
(iii)
 $\pi _3$
preserves
$\pi _3$
preserves
 $c^a=a^{2s}c^t$
: we get
$c^a=a^{2s}c^t$
: we get
 $(c^a)^b=(a^{2s}c^t)^b$
, which implies
$(c^a)^b=(a^{2s}c^t)^b$
, which implies
 $a^uc^v=((a^{2s}c^t)^b)^a$
. that is,
$a^uc^v=((a^{2s}c^t)^b)^a$
. that is, $$ \begin{align*}a^uc^v=(a^{-2s}(a^uc^v)^t)^a=a^{-2s}(a^u(a^{2s}c^t)^v)^t =a^{-2s}c^{t^2v}a^{(ru+2s\sum_{l=1}^{v}r^l)\sum_{l=0}^{t-1}r^l},\end{align*} $$
(5-8)By (5-5), (5-8) is equivalent to
$$ \begin{align*}a^uc^v=(a^{-2s}(a^uc^v)^t)^a=a^{-2s}(a^u(a^{2s}c^t)^v)^t =a^{-2s}c^{t^2v}a^{(ru+2s\sum_{l=1}^{v}r^l)\sum_{l=0}^{t-1}r^l},\end{align*} $$
(5-8)By (5-5), (5-8) is equivalent to $$ \begin{align} r(u+2s)\equiv \bigg(ru+2s\sum_{l=1}^{v}r^l\bigg)\sum_{l=0}^{t-1}r^l \,({\mathrm{mod}}\ 2n). \end{align} $$
(5-9)
$$ \begin{align} r(u+2s)\equiv \bigg(ru+2s\sum_{l=1}^{v}r^l\bigg)\sum_{l=0}^{t-1}r^l \,({\mathrm{mod}}\ 2n). \end{align} $$
(5-9) $$ \begin{align} u\sum_{l=1}^{t}r^l-ur-2s\sum_{l=1}^{v}r^l-2sr+2(1-r)\equiv 0 \,({\mathrm{mod}}\ 2n). \end{align} $$
$$ \begin{align} u\sum_{l=1}^{t}r^l-ur-2s\sum_{l=1}^{v}r^l-2sr+2(1-r)\equiv 0 \,({\mathrm{mod}}\ 2n). \end{align} $$
-
(iv)
 $\pi _3^2={\mathrm {Inn}}(a^n)$
: Suppose that n is even. Then,
$\pi _3^2={\mathrm {Inn}}(a^n)$
: Suppose that n is even. Then,
 $a^n\in \langle a^2\rangle $
, which implies
$a^n\in \langle a^2\rangle $
, which implies
 $(a^n)^c=a^n$
. Then,
$(a^n)^c=a^n$
. Then,
 $\pi _3^2={\mathrm {Inn}}(a^n)=1$
. that is,
$\pi _3^2={\mathrm {Inn}}(a^n)=1$
. that is, $$ \begin{align*}c={\mathrm{Inn}}(a^n)(c)=\pi_3^2(c)=c^{b^2}=a^{-u}(a^uc^v)^v=c^{v^2}a^{u\sum_{l=1}^{v}r^l-ur},\end{align*} $$
(5-10)Suppose that n is odd. Then,
$$ \begin{align*}c={\mathrm{Inn}}(a^n)(c)=\pi_3^2(c)=c^{b^2}=a^{-u}(a^uc^v)^v=c^{v^2}a^{u\sum_{l=1}^{v}r^l-ur},\end{align*} $$
(5-10)Suppose that n is odd. Then, $$ \begin{align} u\sum_{l=1}^vr^l-ur\equiv0\,({\mathrm{mod}}\ 2n)\quad\mathrm{{and}}\quad v^2-1\equiv 0\,({\mathrm{mod}}\ m). \end{align} $$
$$ \begin{align} u\sum_{l=1}^vr^l-ur\equiv0\,({\mathrm{mod}}\ 2n)\quad\mathrm{{and}}\quad v^2-1\equiv 0\,({\mathrm{mod}}\ m). \end{align} $$
 $c^{a^n}=c^ta^{(n-1)(1-r)+2sr},$
so that is,
$c^{a^n}=c^ta^{(n-1)(1-r)+2sr},$
so that is, $$ \begin{align*}c^ta^{(n-1)(1-r)+2sr}={\mathrm{Inn}}(a^n)(c)=\pi_3^2(c) =c^{b^2}=a^{-u}(a^uc^v)^v=c^{v^2}a^{u\sum_{l=1}^{v}r^l-ur},\end{align*} $$
(5-11)
$$ \begin{align*}c^ta^{(n-1)(1-r)+2sr}={\mathrm{Inn}}(a^n)(c)=\pi_3^2(c) =c^{b^2}=a^{-u}(a^uc^v)^v=c^{v^2}a^{u\sum_{l=1}^{v}r^l-ur},\end{align*} $$
(5-11) $$ \begin{align} u\sum_{l=1}^vr^l-ur\equiv(n-1)(1-r)+2sr\,({\mathrm{mod}}\ 2n)\quad{and}\quad v^2-t\equiv 0\,({\mathrm{mod}}\ m). \end{align} $$
$$ \begin{align} u\sum_{l=1}^vr^l-ur\equiv(n-1)(1-r)+2sr\,({\mathrm{mod}}\ 2n)\quad{and}\quad v^2-t\equiv 0\,({\mathrm{mod}}\ m). \end{align} $$
Case 2: u is odd. If
![]() $t=1$
, then c normalizes
$t=1$
, then c normalizes
![]() $\langle a\rangle $
, which implies
$\langle a\rangle $
, which implies
![]() $\langle a\rangle \lhd X$
. By Lemma 4.4, we get
$\langle a\rangle \lhd X$
. By Lemma 4.4, we get
![]() $G\lhd X$
. Then,
$G\lhd X$
. Then,
![]() $v=1$
. With the same argument as Case 1,
$v=1$
. With the same argument as Case 1,
 $$ \begin{align} u\sum_{l=1}^{m}(1-2sr)^l\equiv0\,({\mathrm{mod}}\ 2n). \end{align} $$
$$ \begin{align} u\sum_{l=1}^{m}(1-2sr)^l\equiv0\,({\mathrm{mod}}\ 2n). \end{align} $$
So assuming
![]() $t\ne 1$
, we shall get a contradiction.
$t\ne 1$
, we shall get a contradiction.
Let
![]() $S=\langle a^2, c\rangle $
. Since u is odd again, we know that
$S=\langle a^2, c\rangle $
. Since u is odd again, we know that
![]() $\langle a^2\rangle \le S_X< S$
. Since
$\langle a^2\rangle \le S_X< S$
. Since
![]() $|X:S|=~4$
, we have
$|X:S|=~4$
, we have
![]() ${\overline X}=X/S_X=\langle {\overline c}, {\overline a}\rangle .\langle {\overline b}\rangle \lessapprox S_4$
. The only possibility is
${\overline X}=X/S_X=\langle {\overline c}, {\overline a}\rangle .\langle {\overline b}\rangle \lessapprox S_4$
. The only possibility is
![]() ${\mathrm {o}}({\overline c})=2$
and
${\mathrm {o}}({\overline c})=2$
and
![]() ${\overline X} \cong D_8$
so that m is even and v is odd. Then, t is odd, as
${\overline X} \cong D_8$
so that m is even and v is odd. Then, t is odd, as
![]() $t^2\equiv 1\, \pmod {\ m}$
. Moreover, we have
$t^2\equiv 1\, \pmod {\ m}$
. Moreover, we have
![]() $\langle a^2, c^2\rangle =S_X\lhd X$
.
$\langle a^2, c^2\rangle =S_X\lhd X$
.
Consider
![]() ${\overline X}=X/\langle a^2\rangle =\langle {\overline a},{\overline c}\rangle .\langle {\overline b}\rangle $
, where
${\overline X}=X/\langle a^2\rangle =\langle {\overline a},{\overline c}\rangle .\langle {\overline b}\rangle $
, where
![]() ${\overline a}^{{\overline b}}={\overline a},\,{\overline b}^2={\overline a}^n,\,{\overline c}^{{\overline a}}={\overline c}^t$
and
${\overline a}^{{\overline b}}={\overline a},\,{\overline b}^2={\overline a}^n,\,{\overline c}^{{\overline a}}={\overline c}^t$
and
![]() ${\overline c}^{{\overline b}}={\overline a}{\overline c}^v$
. Let
${\overline c}^{{\overline b}}={\overline a}{\overline c}^v$
. Let
![]() $\pi _3$
be defined as above. Since the induced action of
$\pi _3$
be defined as above. Since the induced action of
![]() $\pi _3$
preserves
$\pi _3$
preserves
![]() ${\overline c}^{{\overline a}}={\overline c}^t$
, we have
${\overline c}^{{\overline a}}={\overline c}^t$
, we have
![]() $({\overline a}{\overline c}^v)^{{\overline a}}=({\overline a}{\overline c}^v)^t$
, that is
$({\overline a}{\overline c}^v)^{{\overline a}}=({\overline a}{\overline c}^v)^t$
, that is
![]() ${\overline a}{\overline c}^{tv}={\overline a}{\overline c}^v(({\overline a}{\overline c}^v)^2)^{({t-1})/2} = {\overline a}{\overline c}^v({\overline c}^{tv+v})^{({t-1})/2} ={\overline a}{\overline c}^{v+ ({v(t+1)(t-1)})/2},$
which implies
${\overline a}{\overline c}^{tv}={\overline a}{\overline c}^v(({\overline a}{\overline c}^v)^2)^{({t-1})/2} = {\overline a}{\overline c}^v({\overline c}^{tv+v})^{({t-1})/2} ={\overline a}{\overline c}^{v+ ({v(t+1)(t-1)})/2},$
which implies
![]() $tv\equiv v+ {v(t+1)(t-1)}/2 \,({\mathrm {mod}}\ m).$
Noting that
$tv\equiv v+ {v(t+1)(t-1)}/2 \,({\mathrm {mod}}\ m).$
Noting that
![]() $t^2\equiv 1({\mod m})$
,
$t^2\equiv 1({\mod m})$
,
![]() $t\ne 1$
and
$t\ne 1$
and
![]() $(v, m)=1$
,
$(v, m)=1$
,
Let
![]() $X_1=GS_X=\langle a, b\rangle \langle c^2\rangle $
. By (5-13), we have
$X_1=GS_X=\langle a, b\rangle \langle c^2\rangle $
. By (5-13), we have
![]() $(c^2)^a=(a^{2s}c^t)^2=a^{2s(1+r^{-1})}c^2$
, which implies that
$(c^2)^a=(a^{2s}c^t)^2=a^{2s(1+r^{-1})}c^2$
, which implies that
![]() $c^2$
normalizes
$c^2$
normalizes
![]() $\langle a\rangle $
. By Lemma 4.4, we get
$\langle a\rangle $
. By Lemma 4.4, we get
![]() $G\lhd X_1$
.
$G\lhd X_1$
.
If n is odd, then
![]() $X_1=\langle a,b\rangle \rtimes \langle c^2\rangle \lhd X$
, which implies
$X_1=\langle a,b\rangle \rtimes \langle c^2\rangle \lhd X$
, which implies
![]() $\langle a^n\rangle \lhd X_1$
. Note that
$\langle a^n\rangle \lhd X_1$
. Note that
![]() $a^n$
is an involution and
$a^n$
is an involution and
![]() $\langle c^2\rangle _{X_1}=1$
, then
$\langle c^2\rangle _{X_1}=1$
, then
![]() $a^n$
is the unique involution in
$a^n$
is the unique involution in
![]() $Z(X_1)$
. Then
$Z(X_1)$
. Then
![]() $\langle a^n\rangle { \, \mathrm {char}\,} X_1\lhd X$
, which implies
$\langle a^n\rangle { \, \mathrm {char}\,} X_1\lhd X$
, which implies
![]() $a^n\in G_X$
, that is,
$a^n\in G_X$
, that is,
![]() $\langle a\rangle \lhd X$
. By Lemma 4.4 again, we get
$\langle a\rangle \lhd X$
. By Lemma 4.4 again, we get
![]() $G\lhd X$
, which implies
$G\lhd X$
, which implies
![]() $t=v=1$
, which is a contradiction. So in what follows, we assume that n is even.
$t=v=1$
, which is a contradiction. So in what follows, we assume that n is even.
By
![]() $G\lhd X_1$
, we get
$G\lhd X_1$
, we get
![]() $b^{c^2}\in G$
. Since
$b^{c^2}\in G$
. Since
![]() $b^{c^2}=c^{v(t+1)-2}a^xb$
for some x, we get
$b^{c^2}=c^{v(t+1)-2}a^xb$
for some x, we get
![]() $v(t+1)-2\equiv 0 \,({\mathrm {mod}}\ m)$
. By combining this with (5-13),
$v(t+1)-2\equiv 0 \,({\mathrm {mod}}\ m)$
. By combining this with (5-13),
Since
![]() ${\overline c}={\overline c}^{{\overline b}^2}={\overline a}({\overline a}{\overline c}^v)^v={\overline c}^v({\overline a}{\overline c}^v{\overline a}{\overline c}^v)^{({v-1})/2} ={\overline c}^{v+(tv+v) ({v-1})/2},$
${\overline c}={\overline c}^{{\overline b}^2}={\overline a}({\overline a}{\overline c}^v)^v={\overline c}^v({\overline a}{\overline c}^v{\overline a}{\overline c}^v)^{({v-1})/2} ={\overline c}^{v+(tv+v) ({v-1})/2},$
Then (5-14) and (5-15) may give
![]() $m/2 \equiv 0\,({\mathrm {mod}}\ m)$
, which is a contradiction.
$m/2 \equiv 0\,({\mathrm {mod}}\ m)$
, which is a contradiction.
-
(4) Ensure
 $\langle c\rangle _X=1$
: If
$\langle c\rangle _X=1$
: If
 $t=1$
, then
$t=1$
, then
 $v=1$
and
$v=1$
and
 $1-2sr\equiv r\,({\mathrm {mod}}\ n)$
by (5-5). For any integer w, Since
$1-2sr\equiv r\,({\mathrm {mod}}\ n)$
by (5-5). For any integer w, Since $$ \begin{align*}(c^w)^a=(a^{2s}c)^w=c^wa^{2s\sum_{l=1}^{w}r^l}\quad\mathrm{{and}}\quad (c^w)^b=(a^uc)^w=c^{w}a^{u\sum_{l=1}^{w}(1-2sr)^l}.\end{align*} $$
$$ \begin{align*}(c^w)^a=(a^{2s}c)^w=c^wa^{2s\sum_{l=1}^{w}r^l}\quad\mathrm{{and}}\quad (c^w)^b=(a^uc)^w=c^{w}a^{u\sum_{l=1}^{w}(1-2sr)^l}.\end{align*} $$
 $\langle c\rangle _X=1$
, we know that
$\langle c\rangle _X=1$
, we know that
 $2s\sum _{l=1}^{w}r^l\equiv 0\equiv u\sum _{l=1}^{w}(1-2sr)^l\,({\mathrm {mod}}\ 2n)$
only when
$2s\sum _{l=1}^{w}r^l\equiv 0\equiv u\sum _{l=1}^{w}(1-2sr)^l\,({\mathrm {mod}}\ 2n)$
only when
 $w\equiv 0\,({\mathrm {mod}}\ m)$
.
$w\equiv 0\,({\mathrm {mod}}\ m)$
.
If
![]() $t\neq 1$
, then u is even, for any integer w,
$t\neq 1$
, then u is even, for any integer w,
Since
![]() $\langle c\rangle _X=1$
, we know that
$\langle c\rangle _X=1$
, we know that
![]() $2s\sum _{l=1}^{w}r^l\equiv 0\equiv u\sum _{l=1}^{w}r^l\,({\mathrm {mod}}\ 2n)$
if and only if
$2s\sum _{l=1}^{w}r^l\equiv 0\equiv u\sum _{l=1}^{w}r^l\,({\mathrm {mod}}\ 2n)$
if and only if
![]() $w\equiv 0\,({\mathrm {mod}}\ m)$
.
$w\equiv 0\,({\mathrm {mod}}\ m)$
.
Summarizing (5-2)–(5-12), we get the parameters
![]() $(m,n,r,s,t,u,v)$
as shown in the lemma. Moreover, since all the above conditions are sufficient and necessary, our group
$(m,n,r,s,t,u,v)$
as shown in the lemma. Moreover, since all the above conditions are sufficient and necessary, our group
![]() $X=X(Q)$
really does exist for any given parameters satisfying the equations.
$X=X(Q)$
really does exist for any given parameters satisfying the equations.
5.2
 $M=\langle a^2\rangle \langle c\rangle $
and
$M=\langle a^2\rangle \langle c\rangle $
and
 $X/M_X\cong D_8$
$X/M_X\cong D_8$
Lemma 5.2. Suppose that
![]() $X=X(Q)$
,
$X=X(Q)$
,
![]() $M=\langle a^2\rangle \langle c\rangle $
,
$M=\langle a^2\rangle \langle c\rangle $
,
![]() $X/M_X\cong D_8$
and
$X/M_X\cong D_8$
and
![]() $\langle c\rangle _X=1$
. Then,
$\langle c\rangle _X=1$
. Then,
where either
![]() $w=0$
and
$w=0$
and
![]() $r=s=t=u=1$
; or
$r=s=t=u=1$
; or
 $$ \begin{align*} w\neq0,\,s=u^2\sum_{l=0}^{w-1}r^l+ ({un}/2),\,t=2wu+1,\\ r^{2w}-1\equiv \bigg(u\sum_{l=1}^{w}r^l + n/2\bigg)^2-r\equiv0\,({\mathrm{mod}}\ n),\\ s\sum_{l=1}^tr^{l}+sr\equiv 2sr-u\sum_{l=1}^tr^l+ur\equiv 1-r\,({\mathrm{mod}}\ n),\\ 2w(1+uw)\equiv nw\equiv 2w(r-1)\equiv0\,({\mathrm{mod}}\ m/2) \end{align*} $$
$$ \begin{align*} w\neq0,\,s=u^2\sum_{l=0}^{w-1}r^l+ ({un}/2),\,t=2wu+1,\\ r^{2w}-1\equiv \bigg(u\sum_{l=1}^{w}r^l + n/2\bigg)^2-r\equiv0\,({\mathrm{mod}}\ n),\\ s\sum_{l=1}^tr^{l}+sr\equiv 2sr-u\sum_{l=1}^tr^l+ur\equiv 1-r\,({\mathrm{mod}}\ n),\\ 2w(1+uw)\equiv nw\equiv 2w(r-1)\equiv0\,({\mathrm{mod}}\ m/2) \end{align*} $$
and
![]() $2^{{1+(-1)^u}/2}\sum _{l=1}^{i}r^l \equiv 0\,({\mathrm {mod}}\ n)$
only when
$2^{{1+(-1)^u}/2}\sum _{l=1}^{i}r^l \equiv 0\,({\mathrm {mod}}\ n)$
only when
![]() $i\equiv 0\,({\mathrm {mod}}\ m/2).$
$i\equiv 0\,({\mathrm {mod}}\ m/2).$
Proof. Under the hypothesis,
![]() $M_X=\langle a^2\rangle \rtimes \langle c^2\rangle $
. Set
$M_X=\langle a^2\rangle \rtimes \langle c^2\rangle $
. Set
![]() $2n={\mathrm {o}}(a)$
and
$2n={\mathrm {o}}(a)$
and
![]() $m={\mathrm {o}}(c)$
. If n is odd, then
$m={\mathrm {o}}(c)$
. If n is odd, then
![]() $\langle {\overline a},{\overline b}\rangle \cong {\mathbb {Z}}_4$
, which is a contradiction. So both n and m are even. Since
$\langle {\overline a},{\overline b}\rangle \cong {\mathbb {Z}}_4$
, which is a contradiction. So both n and m are even. Since
![]() $X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong D_8$
, we can choose
$X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong D_8$
, we can choose
![]() ${\overline b}$
in
${\overline b}$
in
![]() $X/M_X$
such that
$X/M_X$
such that
![]() ${\overline a}^{{\overline c}}={\overline b}$
and
${\overline a}^{{\overline c}}={\overline b}$
and
![]() ${\overline b}^{{\overline c}}={\overline a}.$
Set
${\overline b}^{{\overline c}}={\overline a}.$
Set
![]() $c_1:=c^2$
and
$c_1:=c^2$
and
![]() $X_1=GM_X=\langle a,b\rangle \langle c_1\rangle $
. Noting that
$X_1=GM_X=\langle a,b\rangle \langle c_1\rangle $
. Noting that
![]() $\langle a\rangle \langle c_1\rangle \leq X_1$
and
$\langle a\rangle \langle c_1\rangle \leq X_1$
and
![]() $\langle c_1\rangle _{X_1}=1$
, by Lemma 5.1, we get
$\langle c_1\rangle _{X_1}=1$
, by Lemma 5.1, we get
![]() $X_1=\langle a,b,c_1|R, (a^2)^{c_1}=a^{2r},c_1^a=a^{2s}c_1^{t}, c_1^b=a^{2u}c_1^v\rangle $
, where
$X_1=\langle a,b,c_1|R, (a^2)^{c_1}=a^{2r},c_1^a=a^{2s}c_1^{t}, c_1^b=a^{2u}c_1^v\rangle $
, where
 $$ \begin{align} &r^{ m/2}-1\equiv r^{t-1}-1\equiv r^{v-1}-1\equiv u\bigg(\sum_{l=0}^{v-1}r^l-1\bigg)\equiv 0\,({\mathrm{mod}}\ n),\notag\\ &s\sum_{l=1}^tr^{l}+sr\equiv sr+s\sum_{l=1}^{v}r^l-u\sum_{l=1}^tr^l+ur \equiv 1-r\,({\mathrm{mod}}\ n),\notag\\ &t^2\equiv v^2\equiv1\,\bigg({\mathrm{mod}}\ \frac m2\bigg),\notag\\ &s\sum_{l=1}^{i}r^l\equiv u\sum_{l=1}^{i}r^l\equiv 0\,({\mathrm{mod}}\ n) \,\mathrm{if~ and~ only~ if}\, i\equiv 0 \,\bigg({\mathrm{mod}}\ \frac m2\bigg). \end{align} $$
$$ \begin{align} &r^{ m/2}-1\equiv r^{t-1}-1\equiv r^{v-1}-1\equiv u\bigg(\sum_{l=0}^{v-1}r^l-1\bigg)\equiv 0\,({\mathrm{mod}}\ n),\notag\\ &s\sum_{l=1}^tr^{l}+sr\equiv sr+s\sum_{l=1}^{v}r^l-u\sum_{l=1}^tr^l+ur \equiv 1-r\,({\mathrm{mod}}\ n),\notag\\ &t^2\equiv v^2\equiv1\,\bigg({\mathrm{mod}}\ \frac m2\bigg),\notag\\ &s\sum_{l=1}^{i}r^l\equiv u\sum_{l=1}^{i}r^l\equiv 0\,({\mathrm{mod}}\ n) \,\mathrm{if~ and~ only~ if}\, i\equiv 0 \,\bigg({\mathrm{mod}}\ \frac m2\bigg). \end{align} $$
Moreover, since n is even and
![]() $\langle c_1\rangle _{X_1}=1$
, we get that
$\langle c_1\rangle _{X_1}=1$
, we get that
![]() $b^2=a^n$
is the unique involution of
$b^2=a^n$
is the unique involution of
![]() $Z(X_1)$
. Then,
$Z(X_1)$
. Then,
![]() $a^n\in Z(X)$
, that is,
$a^n\in Z(X)$
, that is,
![]() $[b^2,c]=[a^n,c]=1$
. Now
$[b^2,c]=[a^n,c]=1$
. Now
![]() $X=X_1.\langle c\rangle $
. Set
$X=X_1.\langle c\rangle $
. Set
![]() $a^c=bc_1^w$
. Then, X may be defined by R and
$a^c=bc_1^w$
. Then, X may be defined by R and
Then,
![]() $a^{c_1}=a^{1-2sr}c_1^{1-t}$
and
$a^{c_1}=a^{1-2sr}c_1^{1-t}$
and
![]() $b=a^cc_1^{-w}$
.
$b=a^cc_1^{-w}$
.
If
![]() $w\equiv 0\,({\mathrm {mod}}\ m/2)$
, then
$w\equiv 0\,({\mathrm {mod}}\ m/2)$
, then
![]() ${\mathrm {o}}(a)={\mathrm {o}}(a^c)={\mathrm {o}}(b)=4$
, which implies
${\mathrm {o}}(a)={\mathrm {o}}(a^c)={\mathrm {o}}(b)=4$
, which implies
that is, the former part of Lemma 5.2. So in what follows, we assume that
![]() $w\not \equiv 0\,({\mathrm {mod}}\ m/2)$
.
$w\not \equiv 0\,({\mathrm {mod}}\ m/2)$
.
Recalling
![]() $c_1=c^2$
,
$c_1=c^2$
,
Since
![]() $b^2=a^n\in Z(X)$
, we also get
$b^2=a^n\in Z(X)$
, we also get
![]() $b^c=(b^{-1}a^n)^c=(b^c)^{-1}a^n=c_1^{w+t-1}a^{2sr-1+n}.$
Set
$b^c=(b^{-1}a^n)^c=(b^c)^{-1}a^n=c_1^{w+t-1}a^{2sr-1+n}.$
Set
![]() $\pi \in {\mathrm {Aut\,}}(X_1): a\to bc_1^{w}$
,
$\pi \in {\mathrm {Aut\,}}(X_1): a\to bc_1^{w}$
,
![]() $b\to a^{1-2sr}c_1^{1-t-w}$
and
$b\to a^{1-2sr}c_1^{1-t-w}$
and
![]() $c_1\to c_1$
. We need to carry out the following seven steps.
$c_1\to c_1$
. We need to carry out the following seven steps.
-
(i)
 ${\mathrm {o}}(\pi (b))=4:$
Since
${\mathrm {o}}(\pi (b))=4:$
Since
 $b^2\in Z(X)$
, we only need to show
$b^2\in Z(X)$
, we only need to show
 $(b^c)^2=a^n$
: that is,
$(b^c)^2=a^n$
: that is, $$ \begin{align*} a^n&=(b^c)^2=(c_1^{w+t-1}a^{2sr-1+n})^2=c_1^{w+t-1}a^{2sr-1}c_1^{w+t-1}a^{2sr-1}\\ &=c_1^{w+t-1}(c_1^{w+t-1})^aa^{-1}(a^{2sr})^{c_1^{w+t-1}}a^{2sr-1} = c_1^{w+t-1}(a^{2s}c_1^t)^{w+t-1}a^{2sr^{w+1}+2sr-2}\\ &=c_1^{(t+1)(w+t-1)}a^{2sr^{w+1}+2s\sum_{l=1}^{t+w-1}r^l+2sr-2}\\ &=c_1^{w(t+1)}a^{2sr^{w+1}+2s\sum_{l=1}^{w+t-1}r^{l}+2sr-2}, \end{align*} $$
(5-18)which implies
$$ \begin{align*} a^n&=(b^c)^2=(c_1^{w+t-1}a^{2sr-1+n})^2=c_1^{w+t-1}a^{2sr-1}c_1^{w+t-1}a^{2sr-1}\\ &=c_1^{w+t-1}(c_1^{w+t-1})^aa^{-1}(a^{2sr})^{c_1^{w+t-1}}a^{2sr-1} = c_1^{w+t-1}(a^{2s}c_1^t)^{w+t-1}a^{2sr^{w+1}+2sr-2}\\ &=c_1^{(t+1)(w+t-1)}a^{2sr^{w+1}+2s\sum_{l=1}^{t+w-1}r^l+2sr-2}\\ &=c_1^{w(t+1)}a^{2sr^{w+1}+2s\sum_{l=1}^{w+t-1}r^{l}+2sr-2}, \end{align*} $$
(5-18)which implies $$ \begin{align} w(t+1)\equiv 0 \,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\text{and}\quad sr^{w+1}+s\sum_{l=1}^{w+t-1}r^{l}+sr-1\equiv \frac n2\,({\mathrm{mod}}\ n),\hfill \end{align} $$
$$ \begin{align} w(t+1)\equiv 0 \,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\text{and}\quad sr^{w+1}+s\sum_{l=1}^{w+t-1}r^{l}+sr-1\equiv \frac n2\,({\mathrm{mod}}\ n),\hfill \end{align} $$
 $r^{2w}\equiv r^{w(t+1)}\equiv 1\,({\mathrm {mod}}\ n).$
Hence,
$r^{2w}\equiv r^{w(t+1)}\equiv 1\,({\mathrm {mod}}\ n).$
Hence,
 $[c_1^{w(v+1)},a^2]=[c_1^w,a^2]=1$
.
$[c_1^{w(v+1)},a^2]=[c_1^w,a^2]=1$
.
-
(ii)
 ${\mathrm {o}}(\pi (a))=2n$
: that is,
${\mathrm {o}}(\pi (a))=2n$
: that is, $$ \begin{align*} 1&=(bc_1^w)^{2n}=(a^n(c_1^w)^bc_1^w)^n=(bc_1^wbc_1^w)^n=((a^{2u}c_1^v)^wc_1^wa^n)^n \\ &=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^n\\ &=c_1^{nw(v+1)}a^{2nur^w\sum_{l=1}^{w}r^l+n^2}=c_1^{nw(v+1)}, \end{align*} $$
(5-19)
$$ \begin{align*} 1&=(bc_1^w)^{2n}=(a^n(c_1^w)^bc_1^w)^n=(bc_1^wbc_1^w)^n=((a^{2u}c_1^v)^wc_1^wa^n)^n \\ &=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^n\\ &=c_1^{nw(v+1)}a^{2nur^w\sum_{l=1}^{w}r^l+n^2}=c_1^{nw(v+1)}, \end{align*} $$
(5-19) $$ \begin{align} nw(v+1)\equiv0\,\bigg({\mathrm{mod}}\ \frac m2\bigg). \end{align} $$
$$ \begin{align} nw(v+1)\equiv0\,\bigg({\mathrm{mod}}\ \frac m2\bigg). \end{align} $$
-
(iii)
 $\pi $
preserves
$\pi $
preserves
 $(a^2)^{c_1}=a^{2r}$
: and
$(a^2)^{c_1}=a^{2r}$
: and $$ \begin{align*}((a^2)^{c_1})^c\!=\!((a^2)^c)^{c_1}\!=\!(bc_1^wbc_1^w)^{c_1}=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{c_1} =c^{2w(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n}\end{align*} $$
that is,
$$ \begin{align*}((a^2)^{c_1})^c\!=\!((a^2)^c)^{c_1}\!=\!(bc_1^wbc_1^w)^{c_1}=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{c_1} =c^{2w(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n}\end{align*} $$
that is, $$ \begin{align*}(a^{2r})^c=((a^2)^c)^r=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^r =c^{2wr(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n},\end{align*} $$
(5-20)
$$ \begin{align*}(a^{2r})^c=((a^2)^c)^r=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^r =c^{2wr(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n},\end{align*} $$
(5-20) $$ \begin{align} w(v+1)(r-1)\equiv 0\,\bigg({\mathrm{mod}}\ \frac m2\bigg). \end{align} $$
$$ \begin{align} w(v+1)(r-1)\equiv 0\,\bigg({\mathrm{mod}}\ \frac m2\bigg). \end{align} $$
-
(iv)
 $\pi $
preserves
$\pi $
preserves
 $c_1^a=a^{2s}c_1^{t}$
: and
$c_1^a=a^{2s}c_1^{t}$
: and $$ \begin{align*}(c_1^a)^c=c^{-1}a^{-1}cc_1c^{-1}ac={c_1}^{(a^c)}=c_1^{bc_1^w}=(c_1^va^{2ur})^{c_1^w}=c_1^va^{2ur^{w+1}}\end{align*} $$
that is,
$$ \begin{align*}(c_1^a)^c=c^{-1}a^{-1}cc_1c^{-1}ac={c_1}^{(a^c)}=c_1^{bc_1^w}=(c_1^va^{2ur})^{c_1^w}=c_1^va^{2ur^{w+1}}\end{align*} $$
that is, $$ \begin{align*}(a^{2s}c_1^t)^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^sc_1^t =c_1^{ws(v+1)+t}a^{2sur^{w+1}\sum_{l=1}^{w}r^l+ns},\end{align*} $$
(5-21)
$$ \begin{align*}(a^{2s}c_1^t)^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^sc_1^t =c_1^{ws(v+1)+t}a^{2sur^{w+1}\sum_{l=1}^{w}r^l+ns},\end{align*} $$
(5-21) $$ \begin{align} v\equiv ws(v+1)+t\,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\mathrm{{and}}\quad u\equiv su\sum_{l=1}^{w}r^l+\frac{ns}2\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} v\equiv ws(v+1)+t\,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\mathrm{{and}}\quad u\equiv su\sum_{l=1}^{w}r^l+\frac{ns}2\,({\mathrm{mod}}\ n). \end{align} $$
-
(v)
 $\pi $
preserves
$\pi $
preserves
 $c_1^b=a^{2u}c_1^{v}$
: and
$c_1^b=a^{2u}c_1^{v}$
: and $$ \begin{align*}(c_1^b)^c={c_1}^{(b^c)}=c_1^{c_1^{w+t-1}a^{2sr-1+n}}=c_1^ta^{2sr^{2-w}}\end{align*} $$
that is,
$$ \begin{align*}(c_1^b)^c={c_1}^{(b^c)}=c_1^{c_1^{w+t-1}a^{2sr-1+n}}=c_1^ta^{2sr^{2-w}}\end{align*} $$
that is, $$ \begin{align*}(a^{2u}c_1^v)^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^uc_1^v =c_1^{wu(v+1)+v}a^{2u^2r^{w+1}\sum_{l=1}^{w}r^l+un},\end{align*} $$
(5-22)
$$ \begin{align*}(a^{2u}c_1^v)^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^uc_1^v =c_1^{wu(v+1)+v}a^{2u^2r^{w+1}\sum_{l=1}^{w}r^l+un},\end{align*} $$
(5-22) $$ \begin{align} t\equiv wu(v+1)+v\,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\text{and}\quad s\equiv u^2\sum_{l=0}^{w-1}r^l+\frac{un}2\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} t\equiv wu(v+1)+v\,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\text{and}\quad s\equiv u^2\sum_{l=0}^{w-1}r^l+\frac{un}2\,({\mathrm{mod}}\ n). \end{align} $$
-
(vi)
 $\pi ^2={\mathrm {Inn}}(c_1)$
: Recall
$\pi ^2={\mathrm {Inn}}(c_1)$
: Recall
 ${\mathrm {Inn}}(c_1)(a)=a^{1-2sr}c_1^{1-t},\,{\mathrm {Inn}}(c_1)(a^2)=a^{2r}$
and
${\mathrm {Inn}}(c_1)(a)=a^{1-2sr}c_1^{1-t},\,{\mathrm {Inn}}(c_1)(a^2)=a^{2r}$
and
 ${\mathrm {Inn}}(c_1)(b)=c_1^{v-1}a^{2ur}b$
. as desired;
${\mathrm {Inn}}(c_1)(b)=c_1^{v-1}a^{2ur}b$
. as desired; $$ \begin{align*}a^{1-2sr}c^{2-2t}={\mathrm{Inn}}(c_1)(a)=\pi^2(a)=a^{1-2sr}c^{2-2t-2w+2w},\end{align*} $$
that is,
$$ \begin{align*}a^{1-2sr}c^{2-2t}={\mathrm{Inn}}(c_1)(a)=\pi^2(a)=a^{1-2sr}c^{2-2t-2w+2w},\end{align*} $$
that is, $$ \begin{align*} a^{2r}&= {\mathrm{Inn}}(c_1)(a^2)=\pi^2(a^2)=((a^2)^c)^c\\ &= (c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^c =c_1^{w(v+1)}(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{ur^w\sum_{l=1}^{w}r^l+\frac n2}\\ &= c_1^{w(v+1)(1+uw+ n/2)}a^{2(u\sum_{l=1}^wr^l+ n/2)^2}, \end{align*} $$
(5-23)and noting (5-20) and (5-21), we get
$$ \begin{align*} a^{2r}&= {\mathrm{Inn}}(c_1)(a^2)=\pi^2(a^2)=((a^2)^c)^c\\ &= (c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^c =c_1^{w(v+1)}(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{ur^w\sum_{l=1}^{w}r^l+\frac n2}\\ &= c_1^{w(v+1)(1+uw+ n/2)}a^{2(u\sum_{l=1}^wr^l+ n/2)^2}, \end{align*} $$
(5-23)and noting (5-20) and (5-21), we get $$ \begin{align} w(v+1)\bigg(1+uw+\frac n2\bigg)\equiv 0\,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\mathrm{{and}}\quad r\equiv \bigg(u\sum_{l=1}^{w}r^l+ n/2\bigg)^2 \,({\mathrm{mod}}\ n); \end{align} $$
$$ \begin{align} w(v+1)\bigg(1+uw+\frac n2\bigg)\equiv 0\,\bigg({\mathrm{mod}}\ \frac m2\bigg)\quad\mathrm{{and}}\quad r\equiv \bigg(u\sum_{l=1}^{w}r^l+ n/2\bigg)^2 \,({\mathrm{mod}}\ n); \end{align} $$
 $w(v+1)(r-1)\equiv ws(v+1)+t-v\equiv 0 \,({\mathrm {mod}}\ m/2)$
and
$w(v+1)(r-1)\equiv ws(v+1)+t-v\equiv 0 \,({\mathrm {mod}}\ m/2)$
and
 $u\equiv su\sum _{l=1}^{w}r^l+ ({ns}/2)\,({\mathrm {mod}}\ n).$
Then, as desired.
$u\equiv su\sum _{l=1}^{w}r^l+ ({ns}/2)\,({\mathrm {mod}}\ n).$
Then, as desired. $$ \begin{align*} c^{2(v-1)}a^{2ur}b&={\mathrm{Inn}}(c_1)(b)=\pi^2(b)=(c_1^{w+t-1}a^{2sr-1+n})^c\\ &=c_1^{w+t-1}(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{sr}(bc_1^w)^{-1}a^n\\ &=c_1^{t-1+wsr(v+1)}a^{2usr\sum_{l=1}^{w}r^l+srn}b, \end{align*} $$
$$ \begin{align*} c^{2(v-1)}a^{2ur}b&={\mathrm{Inn}}(c_1)(b)=\pi^2(b)=(c_1^{w+t-1}a^{2sr-1+n})^c\\ &=c_1^{w+t-1}(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{sr}(bc_1^w)^{-1}a^n\\ &=c_1^{t-1+wsr(v+1)}a^{2usr\sum_{l=1}^{w}r^l+srn}b, \end{align*} $$
-
(vii) Ensure
 $\langle c\rangle _X=1$
: Since
$\langle c\rangle _X=1$
: Since
 $\langle c\rangle _X\leq M$
, we get
$\langle c\rangle _X\leq M$
, we get
 $\langle c\rangle _X\leq M_X=\langle a^2\rangle \langle c^2\rangle $
. Then,
$\langle c\rangle _X\leq M_X=\langle a^2\rangle \langle c^2\rangle $
. Then,
 $\langle c\rangle _X=\cap _{x\in X}\langle c\rangle ^x=\cap _{x\in G}\langle c\rangle ^x =\cap _{x\in G}\langle c^2\rangle ^x=\langle c^2\rangle _{X_1}=1$
. Recall
$\langle c\rangle _X=\cap _{x\in X}\langle c\rangle ^x=\cap _{x\in G}\langle c\rangle ^x =\cap _{x\in G}\langle c^2\rangle ^x=\langle c^2\rangle _{X_1}=1$
. Recall
 $s\sum _{l=1}^{i}r^l\equiv u\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
$s\sum _{l=1}^{i}r^l\equiv u\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
 $i\equiv 0\,({\mathrm {mod}}\ (m/2)).$
$i\equiv 0\,({\mathrm {mod}}\ (m/2)).$
Now we are ready to determine the parameters by summarizing (5-16)–(5-23). If
![]() $v=1$
, then inserting
$v=1$
, then inserting
![]() $v=1$
in (5-16)–(5-23), we get that
$v=1$
in (5-16)–(5-23), we get that
![]() $s=u^2\sum _{l=0}^{w-1}r^l+ (n/2)$
and
$s=u^2\sum _{l=0}^{w-1}r^l+ (n/2)$
and
![]() $t=2wu+1$
by (5-22);
$t=2wu+1$
by (5-22);
![]() $nw\equiv 0\,({\mathrm {mod}}\ m/2)$
by (5-18), (5-19) and (5-23); and
$nw\equiv 0\,({\mathrm {mod}}\ m/2)$
by (5-18), (5-19) and (5-23); and
![]() $2w(r-1)\equiv 2w(1+uw)\equiv 0\,({\mathrm {mod}}\ m/2)$
by (5-20) and (5-23). All these are summarized in the lemma. So in what follows, we show
$2w(r-1)\equiv 2w(1+uw)\equiv 0\,({\mathrm {mod}}\ m/2)$
by (5-20) and (5-23). All these are summarized in the lemma. So in what follows, we show
![]() $v=1$
, that is,
$v=1$
, that is,
![]() $v\equiv 1\,({\mathrm {mod}}\ m/2)$
.
$v\equiv 1\,({\mathrm {mod}}\ m/2)$
.
Suppose that u is odd. Then by (5-23), we get
![]() $(u,n)=(\sum _{l=1}^{w}r^l, n/2)=1$
as
$(u,n)=(\sum _{l=1}^{w}r^l, n/2)=1$
as
![]() $(r,n)=1$
. Moreover, if
$(r,n)=1$
. Moreover, if
![]() $n/2$
is odd, then
$n/2$
is odd, then
![]() $\sum _{l=1}^{w}r^l$
is even as r is odd. Then by (5-22), we get
$\sum _{l=1}^{w}r^l$
is even as r is odd. Then by (5-22), we get
![]() $s\equiv u^2\sum _{l=0}^{w-1}r^l+ ({n}/2)\,({\mathrm {mod}}\ n)$
, which implies
$s\equiv u^2\sum _{l=0}^{w-1}r^l+ ({n}/2)\,({\mathrm {mod}}\ n)$
, which implies
![]() $(s,n)=1$
. Then, we have
$(s,n)=1$
. Then, we have
![]() $\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
$\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
![]() $i\equiv 0\,({\mathrm {mod}}\ m/2)$
by item (vii) and
$i\equiv 0\,({\mathrm {mod}}\ m/2)$
by item (vii) and
![]() $\sum _{l=0}^{v-1}r^l\equiv 1\,({\mathrm {mod}}\ n)$
from (5-16). Then,
$\sum _{l=0}^{v-1}r^l\equiv 1\,({\mathrm {mod}}\ n)$
from (5-16). Then,
![]() $v\equiv 1\,({\mathrm {mod}}\ m/2)$
.
$v\equiv 1\,({\mathrm {mod}}\ m/2)$
.
Suppose that u is even. Then, by (5-23), we get
![]() $(u, n/2)=(\sum _{l=1}^{w}r^l, n/2)=1$
. Then by (5-22), we get
$(u, n/2)=(\sum _{l=1}^{w}r^l, n/2)=1$
. Then by (5-22), we get
![]() $s\equiv u^2\sum _{l=0}^{w-1}r^l\,({\mathrm {mod}}\ n)$
, which implies s is even. Then, by (5-21), we get
$s\equiv u^2\sum _{l=0}^{w-1}r^l\,({\mathrm {mod}}\ n)$
, which implies s is even. Then, by (5-21), we get
![]() $u\equiv su\sum _{l=1}^{w}r^l\,({\mathrm {mod}}\ n).$
Then, we have
$u\equiv su\sum _{l=1}^{w}r^l\,({\mathrm {mod}}\ n).$
Then, we have
![]() $\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
$\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
![]() $i\equiv 0\,({\mathrm {mod}}\ m/2)$
by item (vii) and
$i\equiv 0\,({\mathrm {mod}}\ m/2)$
by item (vii) and
![]() $\sum _{l=0}^{v-1}r^l\equiv 1\,({\mathrm {mod}}\ n/2)$
from (5-16). Then,
$\sum _{l=0}^{v-1}r^l\equiv 1\,({\mathrm {mod}}\ n/2)$
from (5-16). Then,
![]() $v\equiv 1\,({\mathrm {mod}}\ m/2)$
.
$v\equiv 1\,({\mathrm {mod}}\ m/2)$
.
5.3
 $M=\langle a^2\rangle \langle c\rangle $
and
$M=\langle a^2\rangle \langle c\rangle $
and
 $X/M_X\cong A_4$
$X/M_X\cong A_4$
Lemma 5.3. Suppose that
![]() $X=X(Q)$
,
$X=X(Q)$
,
![]() $M=\langle a^2\rangle \langle c\rangle $
,
$M=\langle a^2\rangle \langle c\rangle $
,
![]() $X/M_X\cong A_4$
and
$X/M_X\cong A_4$
and
![]() $\langle c\rangle _X=1$
. Then,
$\langle c\rangle _X=1$
. Then,
where
![]() $n\equiv 2\,({\mathrm {mod}}\ 4)$
and either:
$n\equiv 2\,({\mathrm {mod}}\ 4)$
and either:
-
(1)
 $i=s=u=0$
,
$i=s=u=0$
,
 $r=x=1$
; or
$r=x=1$
; or -
(2)
 $i=1$
,
$i=1$
,
 $6| m$
,
$6| m$
,
 $r^{m/2}\equiv -1\,({\mathrm {mod}}\ n)$
with
$r^{m/2}\equiv -1\,({\mathrm {mod}}\ n)$
with
 ${\mathrm {o}}(r)=m$
,
${\mathrm {o}}(r)=m$
,
 $s\equiv ({r^{-3}-1})/2 + n/2 \,({\mathrm {mod}}\ n)$
,
$s\equiv ({r^{-3}-1})/2 + n/2 \,({\mathrm {mod}}\ n)$
,
 $u\equiv ({r^3-1})/{2r^2} + n/2\,({\mathrm {mod}}\ n)$
,
$u\equiv ({r^3-1})/{2r^2} + n/2\,({\mathrm {mod}}\ n)$
,
 $x\equiv -r+r^2+ n/2 \,({\mathrm {mod}}\ n)$
.
$x\equiv -r+r^2+ n/2 \,({\mathrm {mod}}\ n)$
.
Proof. Under the hypothesis,
![]() $M_X=\langle a^2\rangle \rtimes \langle c^3\rangle $
. Set
$M_X=\langle a^2\rangle \rtimes \langle c^3\rangle $
. Set
![]() ${\mathrm {o}}(a)=2n$
and
${\mathrm {o}}(a)=2n$
and
![]() ${\mathrm {o}}(c)=m$
. If n is odd, then we get
${\mathrm {o}}(c)=m$
. If n is odd, then we get
![]() $\langle {\overline a},{\overline b}\rangle \cong {\mathbb {Z}}_4$
, which is a contradiction. So n is even and
$\langle {\overline a},{\overline b}\rangle \cong {\mathbb {Z}}_4$
, which is a contradiction. So n is even and
![]() $3| m$
. Since
$3| m$
. Since
![]() $X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong A_4$
, we can choose
$X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong A_4$
, we can choose
![]() ${\overline b}$
such that
${\overline b}$
such that
![]() ${\overline a}^{{\overline c}}={\overline b}$
and
${\overline a}^{{\overline c}}={\overline b}$
and
![]() ${\overline b}^{{\overline c}}={\overline a}{\overline b}$
in
${\overline b}^{{\overline c}}={\overline a}{\overline b}$
in
![]() $X/M_X$
. Set
$X/M_X$
. Set
![]() $c_1:=c^3$
and
$c_1:=c^3$
and
![]() $X_1=GM_X=\langle a,b\rangle \langle c_1\rangle $
. By Lemma 5.1, we get
$X_1=GM_X=\langle a,b\rangle \langle c_1\rangle $
. By Lemma 5.1, we get
where
 $$ \begin{align} &r^{ m/3}-1\equiv r^{t-1}-1\equiv r^{v-1}-1\equiv u\bigg(\sum_{l=0}^{v-1}r^l-1\bigg)\equiv0\,({\mathrm{mod}}\ n),\notag\\ &s\sum_{l=1}^tr^{l}+sr\equiv sr+s\sum_{l=1}^{v}r^l-u\sum_{l=1}^tr^l+ur \equiv 1-r\,({\mathrm{mod}}\ n),\notag\\ &t^2-1\equiv v^2-1\equiv0\,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ &s\sum_{l=1}^{i}r^l\equiv u\sum_{l=1}^{i}r^l\equiv0\,({\mathrm{mod}}\ n)~\mathrm{if~and~only~if}~i\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
$$ \begin{align} &r^{ m/3}-1\equiv r^{t-1}-1\equiv r^{v-1}-1\equiv u\bigg(\sum_{l=0}^{v-1}r^l-1\bigg)\equiv0\,({\mathrm{mod}}\ n),\notag\\ &s\sum_{l=1}^tr^{l}+sr\equiv sr+s\sum_{l=1}^{v}r^l-u\sum_{l=1}^tr^l+ur \equiv 1-r\,({\mathrm{mod}}\ n),\notag\\ &t^2-1\equiv v^2-1\equiv0\,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ &s\sum_{l=1}^{i}r^l\equiv u\sum_{l=1}^{i}r^l\equiv0\,({\mathrm{mod}}\ n)~\mathrm{if~and~only~if}~i\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
Moreover, since n is even and
![]() $\langle c_1\rangle _{X_1}=1$
, we get that
$\langle c_1\rangle _{X_1}=1$
, we get that
![]() $b^2=a^n$
is the unique involution of
$b^2=a^n$
is the unique involution of
![]() $Z(X_1)$
. Then,
$Z(X_1)$
. Then,
![]() $a^n\in Z(X)$
. Now
$a^n\in Z(X)$
. Now
![]() $X=X_1.\langle c\rangle $
. Set
$X=X_1.\langle c\rangle $
. Set
![]() $a^c=bc_1^{w}$
. Then, X may be defined by R and
$a^c=bc_1^{w}$
. Then, X may be defined by R and
If
![]() $w\equiv 0\,({\mathrm {mod}}\ m/3)$
, then
$w\equiv 0\,({\mathrm {mod}}\ m/3)$
, then
![]() ${\mathrm {o}}(a)={\mathrm {o}}(a^c)={\mathrm {o}}(b)=4$
, which implies
${\mathrm {o}}(a)={\mathrm {o}}(a^c)={\mathrm {o}}(b)=4$
, which implies
that is, the former part of Lemma 5.3. So in what follows, we assume
![]() $w\not \equiv 0\,({\mathrm {mod}}\ m/3)$
.
$w\not \equiv 0\,({\mathrm {mod}}\ m/3)$
.
What we should do is to determine the parameters
![]() $r,s,t,u,v,w,x$
and y by analysing the last extension
$r,s,t,u,v,w,x$
and y by analysing the last extension
![]() $X_1.\langle c\rangle $
, where
$X_1.\langle c\rangle $
, where
![]() $a^c=bc_1^{w}$
and
$a^c=bc_1^{w}$
and
![]() $b^c=a^{1+2x}bc_1^{y}$
. Set
$b^c=a^{1+2x}bc_1^{y}$
. Set
![]() $\pi \in {\mathrm {Aut\,}}(X_1): a\to bc_1^{w}, \, b\to a^{1+2x}bc_1^{y}, \quad c_1\to c_1.$
We need to carry out the following eight steps.
$\pi \in {\mathrm {Aut\,}}(X_1): a\to bc_1^{w}, \, b\to a^{1+2x}bc_1^{y}, \quad c_1\to c_1.$
We need to carry out the following eight steps.
-
(i)
 ${\mathrm {o}}(\pi (b))=4$
: Since
${\mathrm {o}}(\pi (b))=4$
: Since
 $b^2\in Z(X)$
, we only need to show
$b^2\in Z(X)$
, we only need to show
 $(b^c)^2=a^n$
: that is,
$(b^c)^2=a^n$
: that is, $$ \begin{align*} a^n&=(b^c)^2=(a^{1+2x}bc_1^y)^2=a^{2x+n}(c_1^y)^{ab}a^{-2x}c_1^y\\ &=a^{2x+n}(c_1^{vt}a^{2u\sum_{l=1}^tr^l-2sr})^ya^{-2x}c_1^y=a^{2x+n}c_1^{vty}a^{2u\sum_{l=1}^{ty}r^l-2s\sum_{l=1}^{y}r^l-2x}c_1^y\\ &=c_1^{vty+y}a^{r^y(2u\sum_{l=1}^{ty}r^l+2x(r^y-1)-2s\sum_{l=1}^{y}r^l)+n}, \end{align*} $$
(5-26)which implies
$$ \begin{align*} a^n&=(b^c)^2=(a^{1+2x}bc_1^y)^2=a^{2x+n}(c_1^y)^{ab}a^{-2x}c_1^y\\ &=a^{2x+n}(c_1^{vt}a^{2u\sum_{l=1}^tr^l-2sr})^ya^{-2x}c_1^y=a^{2x+n}c_1^{vty}a^{2u\sum_{l=1}^{ty}r^l-2s\sum_{l=1}^{y}r^l-2x}c_1^y\\ &=c_1^{vty+y}a^{r^y(2u\sum_{l=1}^{ty}r^l+2x(r^y-1)-2s\sum_{l=1}^{y}r^l)+n}, \end{align*} $$
(5-26)which implies $$ \begin{align} y(tv+1)\equiv0\,\bigg({\mathrm{mod}}\ \frac m3\bigg)\quad\mathrm{{and}}\quad u\sum_{l=1}^{ty}r^l+xr^y-x-s\sum_{l=1}^yr^l\equiv0\,({\mathrm{mod}}\ n), \end{align} $$
$$ \begin{align} y(tv+1)\equiv0\,\bigg({\mathrm{mod}}\ \frac m3\bigg)\quad\mathrm{{and}}\quad u\sum_{l=1}^{ty}r^l+xr^y-x-s\sum_{l=1}^yr^l\equiv0\,({\mathrm{mod}}\ n), \end{align} $$
 $r^{2y}\equiv r^{y(tv+1)}\equiv 1\,({\mathrm {mod}}\ n).$
$r^{2y}\equiv r^{y(tv+1)}\equiv 1\,({\mathrm {mod}}\ n).$
-
(ii)
 ${\mathrm {o}}(\pi (ab))=4$
: Since
${\mathrm {o}}(\pi (ab))=4$
: Since
 $(ab)^2=a^n\in Z(X)$
, we only show
$(ab)^2=a^n\in Z(X)$
, we only show
 $((ab)^c)^2=a^n$
: that is,
$((ab)^c)^2=a^n$
: that is, $$ \begin{align*} a^n&=(bc_1^wa^{1+2x}bc_1^y)^2=(a^{n-1}(c_1^w)^{ab}a^{-2x}c_1^y)^2 =(a^{n-1}c_1^{wvt+y}a^{r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)})^2\\ &=(c_1^{wvt+y})^aa^{-2+r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)} c_1^{wvt+y}a^{r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)}\\ &=c_1^{vw+yt+tvw+y}a^{2((r^{w+y}+1)(ur^y\sum_{l=1}^wr^l-r^yx+s\sum_{l=1}^yr^l)+ s\sum_{l=1}^{vw+yt}r^l-1)}, \end{align*} $$
(5-27)which implies
$$ \begin{align*} a^n&=(bc_1^wa^{1+2x}bc_1^y)^2=(a^{n-1}(c_1^w)^{ab}a^{-2x}c_1^y)^2 =(a^{n-1}c_1^{wvt+y}a^{r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)})^2\\ &=(c_1^{wvt+y})^aa^{-2+r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)} c_1^{wvt+y}a^{r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)}\\ &=c_1^{vw+yt+tvw+y}a^{2((r^{w+y}+1)(ur^y\sum_{l=1}^wr^l-r^yx+s\sum_{l=1}^yr^l)+ s\sum_{l=1}^{vw+yt}r^l-1)}, \end{align*} $$
(5-27)which implies $$ \begin{align} &vw+yt+tvw+y\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ &(r^{w+y}+1)\bigg(ur^y\sum_{l=1}^wr^l-r^yx+s\sum_{l=1}^yr^l\bigg)+ s\sum_{l=1}^{vw+yt}r^l-1\equiv\frac n2\,({\mathrm{mod}}\ n), \end{align} $$
$$ \begin{align} &vw+yt+tvw+y\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ &(r^{w+y}+1)\bigg(ur^y\sum_{l=1}^wr^l-r^yx+s\sum_{l=1}^yr^l\bigg)+ s\sum_{l=1}^{vw+yt}r^l-1\equiv\frac n2\,({\mathrm{mod}}\ n), \end{align} $$
 $r^{2w}\equiv r^{vw+yt+tvw+y}\equiv 1\,({\mathrm {mod}}\ n)$
. Hence,
$r^{2w}\equiv r^{vw+yt+tvw+y}\equiv 1\,({\mathrm {mod}}\ n)$
. Hence,
 $[c_1^{w(v+1)},a^2]\,{=}\, [c_1^{2w},a^2]\,{=}\,1$
.
$[c_1^{w(v+1)},a^2]\,{=}\, [c_1^{2w},a^2]\,{=}\,1$
.
-
(iii)
 ${\mathrm {o}}(\pi (a))=2n$
: that is,
${\mathrm {o}}(\pi (a))=2n$
: that is, $$ \begin{align*} 1&=((a^2)^c)^n=(bc_1^wbc_1^w)^n= (c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^n\\ &=c_1^{nw(v+1)}a^{2nur^w\sum_{l=1}^{w}r^l+n^2}=c_1^{nw(v+1)}, \end{align*} $$
(5-28)
$$ \begin{align*} 1&=((a^2)^c)^n=(bc_1^wbc_1^w)^n= (c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^n\\ &=c_1^{nw(v+1)}a^{2nur^w\sum_{l=1}^{w}r^l+n^2}=c_1^{nw(v+1)}, \end{align*} $$
(5-28) $$ \begin{align} nw(v+1)\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
$$ \begin{align} nw(v+1)\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
-
(iv)
 $\pi $
preserves
$\pi $
preserves
 $(a^2)^{c_1}=a^{2r}$
: and
$(a^2)^{c_1}=a^{2r}$
: and $$ \begin{align*}((a^2)^{c_1})^c=((a^2)^c)^{c_1}=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{c_1} =c_1^{w(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n}\end{align*} $$
that is,
$$ \begin{align*}((a^2)^{c_1})^c=((a^2)^c)^{c_1}=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{c_1} =c_1^{w(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n}\end{align*} $$
that is, $$ \begin{align*}(a^{2r})^c=((a^2)^c)^r=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^r =c_1^{wr(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n},\end{align*} $$
(5-29)
$$ \begin{align*}(a^{2r})^c=((a^2)^c)^r=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^r =c_1^{wr(v+1)}a^{2ur^{w+1}\sum_{l=1}^{w}r^l+n},\end{align*} $$
(5-29) $$ \begin{align} w(v+1)(r-1)\equiv0\,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
$$ \begin{align} w(v+1)(r-1)\equiv0\,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
-
(v)
 $\pi $
preserves
$\pi $
preserves
 $c_1^a=a^{2s}c_1^t$
: and
$c_1^a=a^{2s}c_1^t$
: and $$ \begin{align*}(c_1^a)^c={c_1}^{(a^c)}=c_1^{bc_1^w}=(a^{2u}c_1^v)^{c_1^w}=c_1^va^{2ur^{w+1}}\end{align*} $$
that is,
$$ \begin{align*}(c_1^a)^c={c_1}^{(a^c)}=c_1^{bc_1^w}=(a^{2u}c_1^v)^{c_1^w}=c_1^va^{2ur^{w+1}}\end{align*} $$
that is, $$ \begin{align*}(a^{2s}c_1^t)^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^sc_1^t =c_1^{ws(v+1)+t}a^{2sur^{w+1}\sum_{l=1}^{w}r^l+sn},\end{align*} $$
(5-30)
$$ \begin{align*}(a^{2s}c_1^t)^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^sc_1^t =c_1^{ws(v+1)+t}a^{2sur^{w+1}\sum_{l=1}^{w}r^l+sn},\end{align*} $$
(5-30) $$ \begin{align} v\equiv ws(v+1)+t \,\bigg({\mathrm{mod}}\ \frac m3\bigg)\quad\mathrm{{and}}\quad u\equiv su\sum_{l=1}^{w}r^l+\frac{sn}2\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} v\equiv ws(v+1)+t \,\bigg({\mathrm{mod}}\ \frac m3\bigg)\quad\mathrm{{and}}\quad u\equiv su\sum_{l=1}^{w}r^l+\frac{sn}2\,({\mathrm{mod}}\ n). \end{align} $$
-
(vi)
 $\pi $
preserves
$\pi $
preserves
 $c_1^b=a^{2u}c_1^{v}$
: Since
$c_1^b=a^{2u}c_1^{v}$
: Since
 $b^c=(a^nb^{-1})^c=c_1^{-y}a^{1+2x}b$
, and
$b^c=(a^nb^{-1})^c=c_1^{-y}a^{1+2x}b$
, and $$ \begin{align*}(c_1^b)^c={c_1}^{(b^c)}=c_1^{c_1^{-y}a^{1+2x}b}=c_1^{a^{1+2x}b}=c_1^{tv}a^{2(u\sum_{l=1}^tr^l-sr+x(r-1))}\end{align*} $$
that is,
$$ \begin{align*}(c_1^b)^c={c_1}^{(b^c)}=c_1^{c_1^{-y}a^{1+2x}b}=c_1^{a^{1+2x}b}=c_1^{tv}a^{2(u\sum_{l=1}^tr^l-sr+x(r-1))}\end{align*} $$
that is, $$ \begin{align*}(a^{2u}c_1^{v})^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^uc_1^v =c_1^{wu(v+1)+v}a^{2u^2r^{w+1}\sum_{l=1}^{w}r^l+un},\end{align*} $$
(5-31)
$$ \begin{align*}(a^{2u}c_1^{v})^c=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^uc_1^v =c_1^{wu(v+1)+v}a^{2u^2r^{w+1}\sum_{l=1}^{w}r^l+un},\end{align*} $$
(5-31) $$ \begin{align} &tv\equiv wu(v+1)+v \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ &u\sum_{l=1}^tr^l-sr+x(r-1)\equiv u^2r^{w+1}\sum_{l=1}^{w}r^l+\frac{un}2\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} &tv\equiv wu(v+1)+v \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ &u\sum_{l=1}^tr^l-sr+x(r-1)\equiv u^2r^{w+1}\sum_{l=1}^{w}r^l+\frac{un}2\,({\mathrm{mod}}\ n). \end{align} $$
-
(vii)
 $\pi ^3={\mathrm {Inn}}(c_1)$
: Recall
$\pi ^3={\mathrm {Inn}}(c_1)$
: Recall
 ${\mathrm {Inn}}(c_1)(a)=a^{1-2sr}c_1^{1-t}=a^{-1}c_1^{1-t}a^{2-2sr},\,{\mathrm {Inn}}(c_1)(a^2)=a^{2r}$
and
${\mathrm {Inn}}(c_1)(a)=a^{1-2sr}c_1^{1-t}=a^{-1}c_1^{1-t}a^{2-2sr},\,{\mathrm {Inn}}(c_1)(a^2)=a^{2r}$
and
 ${\mathrm {Inn}}(c_1)(b)=c^{3(v-1)}a^{2ur}b$
. that is,
${\mathrm {Inn}}(c_1)(b)=c^{3(v-1)}a^{2ur}b$
. that is, $$ \begin{align*} &a^{-1}c_1^{1-t}a^{2-2sr}={\mathrm{Inn}}(c_1)(a)=\pi^3(a)=(a^{1+2x}bc_1^{w+y})^c =((a^2)^c)^{x}(ab)^cc_1^{w+y}\\ &\quad =(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^x a^{n-1}c_1^{wvt+y}a^{r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)} c_1^{w+y}\\ &\quad =a^{-1}c_1^{vtw(1+x(v+1))+w+2y}a^{n+2ur^{w}\sum_{l=1}^{tw(1+x(v+1))}r^l -r^{w}(2s\sum_{l=1}^{w(1+x(v+1))}r^l+2ux\sum_{l=w+1}^{2w}r^l+xn+2x)}, \end{align*} $$
(5-32)
$$ \begin{align*} &a^{-1}c_1^{1-t}a^{2-2sr}={\mathrm{Inn}}(c_1)(a)=\pi^3(a)=(a^{1+2x}bc_1^{w+y})^c =((a^2)^c)^{x}(ab)^cc_1^{w+y}\\ &\quad =(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^x a^{n-1}c_1^{wvt+y}a^{r^y(2u\sum_{l=1}^{tw}r^l-2s\sum_{l=1}^{w}r^l-2x)} c_1^{w+y}\\ &\quad =a^{-1}c_1^{vtw(1+x(v+1))+w+2y}a^{n+2ur^{w}\sum_{l=1}^{tw(1+x(v+1))}r^l -r^{w}(2s\sum_{l=1}^{w(1+x(v+1))}r^l+2ux\sum_{l=w+1}^{2w}r^l+xn+2x)}, \end{align*} $$
(5-32) $$ \begin{align} &1-t\equiv t(wv+wxv+wx)+w+2y \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ & r^w\bigg(u\sum_{l=1}^{tw(1+x(v+1))}r^l-s\sum_{l=1}^{w(1+x(v+1))}r^l-ux\sum_{l=w+1}^{2w}r^l-\frac{(x+1)n}2-x\bigg)\notag\\ &\qquad \equiv 1-sr\,({\mathrm{mod}}\ n); \end{align} $$
that is,
$$ \begin{align} &1-t\equiv t(wv+wxv+wx)+w+2y \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\notag\\ & r^w\bigg(u\sum_{l=1}^{tw(1+x(v+1))}r^l-s\sum_{l=1}^{w(1+x(v+1))}r^l-ux\sum_{l=w+1}^{2w}r^l-\frac{(x+1)n}2-x\bigg)\notag\\ &\qquad \equiv 1-sr\,({\mathrm{mod}}\ n); \end{align} $$
that is, $$ \begin{align*} a^{2r}&= {\mathrm{Inn}}(c_1)(a^2)=\pi^3(a^2)=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{c^2}\\ &= c_1^{w(v+1)}((c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{ur^w\sum_{l=1}^{w}r^l})^ca^n\\ &= c_1^{w(v+1)+uw^2(v+1)}((c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{(u\sum_{l=1}^{w}r^l)^2}a^{uwn+n}\\ &= c_1^{w(v+1)+uw^2(v+1)(1+uw)}a^{2r^w(u\sum_{l=1}^{w}r^l)^3+n}, \end{align*} $$
(5-33)which implies
$$ \begin{align*} a^{2r}&= {\mathrm{Inn}}(c_1)(a^2)=\pi^3(a^2)=(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{c^2}\\ &= c_1^{w(v+1)}((c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{ur^w\sum_{l=1}^{w}r^l})^ca^n\\ &= c_1^{w(v+1)+uw^2(v+1)}((c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{(u\sum_{l=1}^{w}r^l)^2}a^{uwn+n}\\ &= c_1^{w(v+1)+uw^2(v+1)(1+uw)}a^{2r^w(u\sum_{l=1}^{w}r^l)^3+n}, \end{align*} $$
(5-33)which implies $$ \begin{align}\begin{aligned} &w(v+1) +uw^2(v+1)(uw+1)\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\\ & r\equiv r^w \bigg(u\sum_{l=1}^wr^l\bigg)^3 + \frac n2\,({\mathrm{mod}}\ n), \end{aligned}\end{align} $$
$$ \begin{align}\begin{aligned} &w(v+1) +uw^2(v+1)(uw+1)\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg),\\ & r\equiv r^w \bigg(u\sum_{l=1}^wr^l\bigg)^3 + \frac n2\,({\mathrm{mod}}\ n), \end{aligned}\end{align} $$
 $(u, n/2)=(\sum _{l=1}^wr^l, n/2)=1$
as
$(u, n/2)=(\sum _{l=1}^wr^l, n/2)=1$
as
 $(r,n)=1$
. Moreover, if u is even, then
$(r,n)=1$
. Moreover, if u is even, then
 $n/2$
is odd. Noting (5-30), that is,
$n/2$
is odd. Noting (5-30), that is,
 $u\equiv su\sum _{l=1}^{w}r^l+ {sn}/2 \,({\mathrm {mod}}\ n)$
, and
$u\equiv su\sum _{l=1}^{w}r^l+ {sn}/2 \,({\mathrm {mod}}\ n)$
, and
 $a^{c_1}=b^{c^2}c_1^w$
, we get that
$a^{c_1}=b^{c^2}c_1^w$
, we get that
 $(s, n/2)=1$
and that is,
$(s, n/2)=1$
and that is, $$ \begin{align*} c_1^{v-1}a^{2ur}b&={\mathrm{Inn}}(c_1)(b)=\pi^3(b)=a^n((b^{-1})^{c^2})^c=a^n((a^{c_1}c_1^{-w})^{-1})^c\\ &=c_1^{w}(c_1^{t-1}a^{2sr-1})^ca^n =c_1^{w+t-1}(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{sr}(bc_1^w)^{-1}a^n\\ &=c_1^{t-1+ws(v+1)}a^{2usr\sum_{l=1}^{w}r^l+sn}b, \end{align*} $$
(5-34)
$$ \begin{align*} c_1^{v-1}a^{2ur}b&={\mathrm{Inn}}(c_1)(b)=\pi^3(b)=a^n((b^{-1})^{c^2})^c=a^n((a^{c_1}c_1^{-w})^{-1})^c\\ &=c_1^{w}(c_1^{t-1}a^{2sr-1})^ca^n =c_1^{w+t-1}(c_1^{w(v+1)}a^{2ur^w\sum_{l=1}^{w}r^l+n})^{sr}(bc_1^w)^{-1}a^n\\ &=c_1^{t-1+ws(v+1)}a^{2usr\sum_{l=1}^{w}r^l+sn}b, \end{align*} $$
(5-34) $$ \begin{align} v\equiv t+ws(v+1)\,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
$$ \begin{align} v\equiv t+ws(v+1)\,\bigg({\mathrm{mod}}\ \frac m3\bigg). \end{align} $$
-
(viii) Ensure
 $\langle c\rangle _X=1$
: Since
$\langle c\rangle _X=1$
: Since
 $\langle c\rangle _X\leq M$
, we get
$\langle c\rangle _X\leq M$
, we get
 $\langle c\rangle _X\leq M_X=\langle a^2\rangle \langle c^3\rangle $
. Noting that
$\langle c\rangle _X\leq M_X=\langle a^2\rangle \langle c^3\rangle $
. Noting that
 $\langle c\rangle _X{\kern-1pt}={\kern-1pt}\cap _{x\in X}\langle c\rangle ^x{\kern-1pt}={\kern-1pt}\cap _{x\in G}\langle c\rangle ^x {\kern-1pt}={\kern-1pt}\cap _{x\in G}\langle c^3\rangle ^x{\kern-1pt}={\kern-1pt}\langle c^3\rangle _{X_1}=1$
,
$\langle c\rangle _X{\kern-1pt}={\kern-1pt}\cap _{x\in X}\langle c\rangle ^x{\kern-1pt}={\kern-1pt}\cap _{x\in G}\langle c\rangle ^x {\kern-1pt}={\kern-1pt}\cap _{x\in G}\langle c^3\rangle ^x{\kern-1pt}={\kern-1pt}\langle c^3\rangle _{X_1}=1$
,
 $s\sum _{l=1}^{i}r^l{\kern-1pt}\equiv{\kern-1pt} u\sum _{l=1}^{i}r^l{\kern-1pt}\equiv{\kern-1pt} 0\,({\mathrm {mod}}\ n)$
if and only if
$s\sum _{l=1}^{i}r^l{\kern-1pt}\equiv{\kern-1pt} u\sum _{l=1}^{i}r^l{\kern-1pt}\equiv{\kern-1pt} 0\,({\mathrm {mod}}\ n)$
if and only if
 $i\equiv 0\,({\mathrm {mod}}\ m/3)$
,
$i\equiv 0\,({\mathrm {mod}}\ m/3)$
,
 $(s, n/2)=(u, n/2)=1$
, and both u and s are even only if
$(s, n/2)=(u, n/2)=1$
, and both u and s are even only if
 $n/2$
is odd, we have
$n/2$
is odd, we have
 $2^{{(1+(-1)^u})/2}\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
$2^{{(1+(-1)^u})/2}\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
 $i\equiv 0\,({\mathrm {mod}}\ m/3)$
.
$i\equiv 0\,({\mathrm {mod}}\ m/3)$
.
Now we are ready to determine the parameters by summarizing (5-24)–(5-34) by the following three steps.
Step 1: Show
![]() $t=v=1$
,
$t=v=1$
,
![]() $w=m/6$
,
$w=m/6$
,
![]() $r^w\equiv -1\,({\mathrm {mod}}\ n)$
and
$r^w\equiv -1\,({\mathrm {mod}}\ n)$
and
![]() $s\equiv ({1-r})/{2r}\,({\mathrm {mod}}\ n/2)$
. Since
$s\equiv ({1-r})/{2r}\,({\mathrm {mod}}\ n/2)$
. Since
![]() $(r,n)=(u, n/2)=1$
(after (5-33)), we get from (5-24) that
$(r,n)=(u, n/2)=1$
(after (5-33)), we get from (5-24) that
![]() $2^{({1+(-1)^u})/2} \sum _{l=1}^{v-1}r^l\equiv 0\,({\mathrm {mod}}\ n).$
By item (viii),
$2^{({1+(-1)^u})/2} \sum _{l=1}^{v-1}r^l\equiv 0\,({\mathrm {mod}}\ n).$
By item (viii),
![]() $2^{({1+(-1)^u})/2}\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
$2^{({1+(-1)^u})/2}\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n)$
if and only if
![]() $i\equiv 0\,({\mathrm {mod}}\ m/3)$
, which means
$i\equiv 0\,({\mathrm {mod}}\ m/3)$
, which means
![]() $v\equiv 1\,({\mathrm {mod}}\ m/3)$
.
$v\equiv 1\,({\mathrm {mod}}\ m/3)$
.
Inserting
![]() $v=1$
in (5-24)–(5-34), we get that
$v=1$
in (5-24)–(5-34), we get that
![]() $2w(wu+1)\equiv 0\,({\mathrm {mod}}\ m/3)$
and
$2w(wu+1)\equiv 0\,({\mathrm {mod}}\ m/3)$
and
![]() $t\equiv 1+2wu\equiv 1-2ws\,({\mathrm {mod}}\ m/3)$
by (5-27), (5-30) and (5-31). Then,
$t\equiv 1+2wu\equiv 1-2ws\,({\mathrm {mod}}\ m/3)$
by (5-27), (5-30) and (5-31). Then,
![]() $2w\equiv 0\,({\mathrm {mod}}\ m/3)$
by (5-33), which implies
$2w\equiv 0\,({\mathrm {mod}}\ m/3)$
by (5-33), which implies
![]() $w= m/6$
as
$w= m/6$
as
![]() $w\not \equiv 0\,({\mathrm {mod}}\ m/3)$
. Inserting
$w\not \equiv 0\,({\mathrm {mod}}\ m/3)$
. Inserting
![]() $w= m/6$
in (5-24)–(5-34) again, we get
$w= m/6$
in (5-24)–(5-34) again, we get
![]() $t\equiv 1\,({\mathrm {mod}}\ m/3)$
by (5-31),
$t\equiv 1\,({\mathrm {mod}}\ m/3)$
by (5-31),
![]() $s\equiv ({1-r})/{2r}\,({\mathrm {mod}}\ n/2)$
by (5-24), and
$s\equiv ({1-r})/{2r}\,({\mathrm {mod}}\ n/2)$
by (5-24), and
![]() $r^w\equiv -1\,({\mathrm {mod}}\ n)$
by (5-24) and (5-33).
$r^w\equiv -1\,({\mathrm {mod}}\ n)$
by (5-24) and (5-33).
Step 2: Show
![]() $y=0$
. Since
$y=0$
. Since
![]() $2y\equiv 0\,({\mathrm {mod}}\ m/3)$
by (5-26), we know that y is either
$2y\equiv 0\,({\mathrm {mod}}\ m/3)$
by (5-26), we know that y is either
![]() $0$
or
$0$
or
![]() $m/6$
. Arguing by contradiction, assume that
$m/6$
. Arguing by contradiction, assume that
![]() $y = m/6 =w$
. Then by (5-27), we get that
$y = m/6 =w$
. Then by (5-27), we get that
![]() $n/2$
is odd, and with 5-26) and (5-27), we get
$n/2$
is odd, and with 5-26) and (5-27), we get
![]() $2x\equiv (u-s)\sum _{l=1}^{w}r^l\equiv (n/2) -1\,({\mathrm {mod}}\ n)$
. By (5-30) and
$2x\equiv (u-s)\sum _{l=1}^{w}r^l\equiv (n/2) -1\,({\mathrm {mod}}\ n)$
. By (5-30) and
![]() $(u, n/2)=1$
, we get
$(u, n/2)=1$
, we get
![]() $s\sum _{l=1}^{w}r^l\equiv 1+ ({sn})/2\,({\mathrm {mod}}\ n)$
, then
$s\sum _{l=1}^{w}r^l\equiv 1+ ({sn})/2\,({\mathrm {mod}}\ n)$
, then
![]() $u\sum _{l=1}^{w}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
, which contradicts
$u\sum _{l=1}^{w}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
, which contradicts
![]() $(u, n/2)=(\sum _{l=1}^{w}r^l, n/2)=1$
. So
$(u, n/2)=(\sum _{l=1}^{w}r^l, n/2)=1$
. So
![]() $y=0$
.
$y=0$
.
Step 3: Determine u and x. By (5-27), we get
![]() $s\sum _{l=1}^{w}r^l\equiv 1+ n/2\,({\mathrm {mod}}\ n).$
Then,
$s\sum _{l=1}^{w}r^l\equiv 1+ n/2\,({\mathrm {mod}}\ n).$
Then,
![]() ${(s+u)n}/2 \equiv 0 \,({\mathrm {mod}}\ n)$
in (5-30), which implies
${(s+u)n}/2 \equiv 0 \,({\mathrm {mod}}\ n)$
in (5-30), which implies
![]() $u\equiv s\,({\mathrm {mod}}\ 2)$
. By (5-31), we get
$u\equiv s\,({\mathrm {mod}}\ 2)$
. By (5-31), we get
![]() $x(r-1)\equiv (s-u)r-u^2r\sum _{l=1}^{w}r^l+ ({un}/2)\,({\mathrm {mod}}\ n)$
. If
$x(r-1)\equiv (s-u)r-u^2r\sum _{l=1}^{w}r^l+ ({un}/2)\,({\mathrm {mod}}\ n)$
. If
![]() $n/2$
is even, then
$n/2$
is even, then
![]() $u\sum _{l=1}^{w}r^l$
is even. However, in (5-33), we get
$u\sum _{l=1}^{w}r^l$
is even. However, in (5-33), we get
![]() $r\equiv -(u\sum _{l=1}^wr^l)^3+ n/2 \,({\mathrm {mod}}\ n)$
which implies that
$r\equiv -(u\sum _{l=1}^wr^l)^3+ n/2 \,({\mathrm {mod}}\ n)$
which implies that
![]() $u\sum _{l=1}^{w}r^l$
is odd as
$u\sum _{l=1}^{w}r^l$
is odd as
![]() $2\nmid r$
and
$2\nmid r$
and
![]() $2|n/2$
, which is a contradiction. So
$2|n/2$
, which is a contradiction. So
![]() $n/2$
is odd. Then we get
$n/2$
is odd. Then we get
![]() $u\sum _{l=1}^wr^l$
is even in (5-33) and u is even in (5-31). Then,
$u\sum _{l=1}^wr^l$
is even in (5-33) and u is even in (5-31). Then,
![]() $\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
$\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
![]() $ i\equiv 0\,({\mathrm {mod}}\ m/3)$
. Recall
$ i\equiv 0\,({\mathrm {mod}}\ m/3)$
. Recall
![]() $s\equiv ({r^{-1}-1})/2\,({\mathrm {mod}}\ n/2)$
in (5-24) and
$s\equiv ({r^{-1}-1})/2\,({\mathrm {mod}}\ n/2)$
in (5-24) and
![]() $s\sum _{l=1}^{w}r^l\equiv 1+ n/2\,({\mathrm {mod}}\ n)$
in (5-27). Since
$s\sum _{l=1}^{w}r^l\equiv 1+ n/2\,({\mathrm {mod}}\ n)$
in (5-27). Since
![]() $(\sum _{l=1}^{w}r^l, n/2)=1$
and
$(\sum _{l=1}^{w}r^l, n/2)=1$
and
![]() $x(r-1)\equiv (s-u)r-u^2r\sum _{l=1}^{w}r^l\,({\mathrm {mod}}\ n)$
, we get
$x(r-1)\equiv (s-u)r-u^2r\sum _{l=1}^{w}r^l\,({\mathrm {mod}}\ n)$
, we get
![]() $2x\equiv u\sum _{l=1}^{w}r^l+(u\sum _{l=1}^{w}r^l)^2-1+ n/2\,({\mathrm {mod}}\ n)$
. Additionally, by (5-33), we get
$2x\equiv u\sum _{l=1}^{w}r^l+(u\sum _{l=1}^{w}r^l)^2-1+ n/2\,({\mathrm {mod}}\ n)$
. Additionally, by (5-33), we get
![]() $-r\equiv (u\sum _{l=1}^{w}r^l)^3+ n/2\,({\mathrm {mod}}\ n).$
Take
$-r\equiv (u\sum _{l=1}^{w}r^l)^3+ n/2\,({\mathrm {mod}}\ n).$
Take
![]() $l=-u\sum _{l=1}^{w}r^l+ n/2$
, then
$l=-u\sum _{l=1}^{w}r^l+ n/2$
, then
![]() $r\equiv l^3\,({\mathrm {mod}}\ n)$
,
$r\equiv l^3\,({\mathrm {mod}}\ n)$
,
![]() $u\equiv ({l^3-1})/{2l^2} + n/2\,({\mathrm {mod}}\ n)$
and
$u\equiv ({l^3-1})/{2l^2} + n/2\,({\mathrm {mod}}\ n)$
and
![]() $1+2x\equiv -l+l^2+ n/2 \,({\mathrm {mod}}\ n)$
. Let us rewrite l as r and
$1+2x\equiv -l+l^2+ n/2 \,({\mathrm {mod}}\ n)$
. Let us rewrite l as r and
![]() $1+2x$
as x for the sake of formatting. Then,
$1+2x$
as x for the sake of formatting. Then,
![]() $s\equiv (({r^{-3}-1})/2) + n/2\,({\mathrm {mod}}\ n)$
,
$s\equiv (({r^{-3}-1})/2) + n/2\,({\mathrm {mod}}\ n)$
,
![]() $u\equiv (({r^3-1})/{2r^2}) + n/2 \,({\mathrm {mod}}\ n)$
and
$u\equiv (({r^3-1})/{2r^2}) + n/2 \,({\mathrm {mod}}\ n)$
and
![]() $x\equiv -r+r^2 + n/2 \,({\mathrm {mod}}\ n)$
.
$x\equiv -r+r^2 + n/2 \,({\mathrm {mod}}\ n)$
.
In fact, if we add the conditions
![]() $t=1$
and
$t=1$
and
![]() $w\ne 0$
and delete
$w\ne 0$
and delete
![]() $\langle c\rangle _X=1$
in the above calculation, then we can get the following.
$\langle c\rangle _X=1$
in the above calculation, then we can get the following.
Lemma 5.4. With the above notation, suppose that
![]() $t=1$
and
$t=1$
and
![]() $w\ne 0$
. Then,
$w\ne 0$
. Then,
where
![]() $n\equiv 2\,({\mathrm {mod}}\ 4)$
,
$n\equiv 2\,({\mathrm {mod}}\ 4)$
,
![]() $m\!\equiv \! 0\,({\mathrm {mod}}\ 6)$
,
$m\!\equiv \! 0\,({\mathrm {mod}}\ 6)$
,
![]() $r^{m/2}\equiv -1\,({\mathrm {mod}}\ n)$
,
$r^{m/2}\equiv -1\,({\mathrm {mod}}\ n)$
,
![]() $s\equiv (({r^{-3}-1})/2) + n/2\,({\mathrm {mod}}\ n)$
,
$s\equiv (({r^{-3}-1})/2) + n/2\,({\mathrm {mod}}\ n)$
,
![]() $u\equiv (({r^3-1})/{2r^2}) + n/2\,({\mathrm {mod}}\ n)$
and
$u\equiv (({r^3-1})/{2r^2}) + n/2\,({\mathrm {mod}}\ n)$
and
![]() $x\equiv -r+r^2 + n/2 \,({\mathrm {mod}}\ n)$
.
$x\equiv -r+r^2 + n/2 \,({\mathrm {mod}}\ n)$
.
5.4
 $M=\langle a^4\rangle \langle c\rangle $
and
$M=\langle a^4\rangle \langle c\rangle $
and
 $X/M_X\cong S_4$
$X/M_X\cong S_4$
Lemma 5.5. Suppose that
![]() $X=X(Q)$
,
$X=X(Q)$
,
![]() $M=\langle a^4\rangle \langle c\rangle $
,
$M=\langle a^4\rangle \langle c\rangle $
,
![]() $X/M_X\cong S_4$
and
$X/M_X\cong S_4$
and
![]() $\langle c\rangle _X=1$
. Then,
$\langle c\rangle _X=1$
. Then,
 $X=\langle a,b,c\mid R, (a^4)^{c}=a^{4r}, (c^3)^{a^2}=a^{4s}c^3,(c^3)^b=a^{4u}c^3, (a^2)^c=bc^{{im}/2},b^c=a^{2x}b,c^a=a^{2(1+2z)}c^{1 + ({jm}/3)}\rangle ,$
where either:
$X=\langle a,b,c\mid R, (a^4)^{c}=a^{4r}, (c^3)^{a^2}=a^{4s}c^3,(c^3)^b=a^{4u}c^3, (a^2)^c=bc^{{im}/2},b^c=a^{2x}b,c^a=a^{2(1+2z)}c^{1 + ({jm}/3)}\rangle ,$
where either:
-
(1)
 $i=0$
,
$i=0$
,
 $r=j=1,x=3,s=u=z=0$
; or
$r=j=1,x=3,s=u=z=0$
; or -
(2)
 $i=1$
,
$i=1$
,
 $n\equiv 4\,({\mathrm {mod}}\ 8)$
,
$n\equiv 4\,({\mathrm {mod}}\ 8)$
,
 $6| m$
,
$6| m$
,
 $r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
,
$r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
,
 ${\mathrm {o}}(r)=m$
,
${\mathrm {o}}(r)=m$
,
 $s\equiv ({r^{-3}-1})/2 + n/4 \,({\mathrm {mod}}\ n/2)$
,
$s\equiv ({r^{-3}-1})/2 + n/4 \,({\mathrm {mod}}\ n/2)$
,
 $u\equiv ({r^3-1})/{2r^2} + n/4 \,({\mathrm {mod}}\ n/2)$
,
$u\equiv ({r^3-1})/{2r^2} + n/4 \,({\mathrm {mod}}\ n/2)$
,
 $x \equiv -r+r^2+ n/4\,({\mathrm {mod}}\ n/2)$
,
$x \equiv -r+r^2+ n/4\,({\mathrm {mod}}\ n/2)$
,
 $1+2z\equiv ({1-r})/{2r} \,({\mathrm {mod}}\ n/2)$
,
$1+2z\equiv ({1-r})/{2r} \,({\mathrm {mod}}\ n/2)$
,
 $j\in \{1,2\}$
.
$j\in \{1,2\}$
.
Proof. Under the hypothesis,
![]() $M_X=\langle a^4\rangle \rtimes \langle c^3\rangle $
. Set
$M_X=\langle a^4\rangle \rtimes \langle c^3\rangle $
. Set
![]() $2n={\mathrm {o}}(a)$
and
$2n={\mathrm {o}}(a)$
and
![]() $m={\mathrm {o}}(c)$
. Then, n is even and
$m={\mathrm {o}}(c)$
. Then, n is even and
![]() $3| m$
. If
$3| m$
. If
![]() $n/2$
is odd, then
$n/2$
is odd, then
![]() $\langle {\overline a},{\overline b}\rangle \cong Q_8$
, a contradiction. So
$\langle {\overline a},{\overline b}\rangle \cong Q_8$
, a contradiction. So
![]() $n/2$
is even. Since
$n/2$
is even. Since
![]() $X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong S_4$
, we can choose
$X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong S_4$
, we can choose
![]() ${\overline b}$
such that the form of
${\overline b}$
such that the form of
![]() $X/M_X$
is the following:
$X/M_X$
is the following:
![]() $({\overline a}^2)^{{\overline c}}={\overline b},\,{\overline b}^{{\overline c}}={\overline a}^2{\overline b}$
and
$({\overline a}^2)^{{\overline c}}={\overline b},\,{\overline b}^{{\overline c}}={\overline a}^2{\overline b}$
and
![]() $({\overline c})^{{\overline a}}={\overline a}^2{\overline c}^2.$
Take
$({\overline c})^{{\overline a}}={\overline a}^2{\overline c}^2.$
Take
![]() $a_1=a^2$
and
$a_1=a^2$
and
![]() $c_1=c^3$
. Then, we set
$c_1=c^3$
. Then, we set
![]() $a_1^c=bc_1^w,\, b^c=a_1^xbc_1^{y}$
and
$a_1^c=bc_1^w,\, b^c=a_1^xbc_1^{y}$
and
![]() $c^a=a_1^{1+2z}c^{2+3d}$
, where x is odd.
$c^a=a_1^{1+2z}c^{2+3d}$
, where x is odd.
Suppose
![]() $w\equiv 0\,({\mathrm {mod}}\ m/3)$
. Note that
$w\equiv 0\,({\mathrm {mod}}\ m/3)$
. Note that
![]() ${\mathrm {o}}(a_1)={\mathrm {o}}(a_1^c)={\mathrm {o}}(b)=4$
, which implies
${\mathrm {o}}(a_1)={\mathrm {o}}(a_1^c)={\mathrm {o}}(b)=4$
, which implies
![]() $G\cong Q_{16}$
. Thus, X can only have the following form:
$G\cong Q_{16}$
. Thus, X can only have the following form:
So in what follows, we assume that
![]() $w\not \equiv 0\,({\mathrm {mod}}\ m/3)$
.
$w\not \equiv 0\,({\mathrm {mod}}\ m/3)$
.
Then consider
![]() $X_1=GM_X=\langle a,b\rangle \langle c_1\rangle $
. Noting
$X_1=GM_X=\langle a,b\rangle \langle c_1\rangle $
. Noting
![]() $\langle a\rangle \langle c_1\rangle \leq X_1$
and
$\langle a\rangle \langle c_1\rangle \leq X_1$
and
![]() $\langle c_1\rangle _{X_1}=1$
, by Theorem 1.2, we know
$\langle c_1\rangle _{X_1}=1$
, by Theorem 1.2, we know
![]() $\langle a_1\rangle \lhd X_1$
, which implies that
$\langle a_1\rangle \lhd X_1$
, which implies that
![]() $c_1$
normalizes
$c_1$
normalizes
![]() $\langle a_1\rangle $
. Take
$\langle a_1\rangle $
. Take
![]() $X_2=\langle a_1,b\rangle \langle c\rangle $
. Then we get
$X_2=\langle a_1,b\rangle \langle c\rangle $
. Then we get
![]() $X_2=(\langle a_1,b\rangle \langle c_1\rangle ).\langle c\rangle $
. Note that
$X_2=(\langle a_1,b\rangle \langle c_1\rangle ).\langle c\rangle $
. Note that
![]() $c_1$
normalizes
$c_1$
normalizes
![]() $\langle a_1\rangle $
in
$\langle a_1\rangle $
in
![]() $X_2$
. Thus, by Lemma 5.4, we get
$X_2$
. Thus, by Lemma 5.4, we get
where
 $$ \begin{align*} &n\equiv 4\,({\mathrm{mod}}\ 8), m\equiv 0\,({\mathrm{mod}}\ 6), r^{m/2}\equiv-1\,\bigg({\mathrm{mod}}\ \frac n2\bigg),\notag\\ &s\equiv \frac{r^{-3}-1}2+\frac n4 \,\bigg({\mathrm{mod}}\ \frac n2\bigg), u\equiv\frac{r^3-1}{2r^2}+\frac n4 \,\bigg({\mathrm{mod}}\ \frac n2\bigg), x\equiv -r+r^2+\frac n4 \,\bigg({\mathrm{mod}}\ \frac n2\bigg). \end{align*} $$
$$ \begin{align*} &n\equiv 4\,({\mathrm{mod}}\ 8), m\equiv 0\,({\mathrm{mod}}\ 6), r^{m/2}\equiv-1\,\bigg({\mathrm{mod}}\ \frac n2\bigg),\notag\\ &s\equiv \frac{r^{-3}-1}2+\frac n4 \,\bigg({\mathrm{mod}}\ \frac n2\bigg), u\equiv\frac{r^3-1}{2r^2}+\frac n4 \,\bigg({\mathrm{mod}}\ \frac n2\bigg), x\equiv -r+r^2+\frac n4 \,\bigg({\mathrm{mod}}\ \frac n2\bigg). \end{align*} $$
Note
![]() $X=X_2.\langle a\rangle $
. Thus, X may be defined by R and
$X=X_2.\langle a\rangle $
. Thus, X may be defined by R and
What we should do is to determine the parameters
![]() $r,z$
and d by analysing the last one extension
$r,z$
and d by analysing the last one extension
![]() $X_2.\langle a\rangle $
, where
$X_2.\langle a\rangle $
, where
![]() $c^a=a_1^{1+2z}c^{2+3d}$
. Set
$c^a=a_1^{1+2z}c^{2+3d}$
. Set
![]() $\pi \in {\mathrm {Aut\,}}(X_1) : a_1\to a_1$
,
$\pi \in {\mathrm {Aut\,}}(X_1) : a_1\to a_1$
,
![]() $b\to a_1^{-1}b$
and
$b\to a_1^{-1}b$
and
![]() $c\to a_1^{1+2z}c^{2+3d}$
, where d is odd. We need to carry out the following eight steps.
$c\to a_1^{1+2z}c^{2+3d}$
, where d is odd. We need to carry out the following eight steps.
-
(i)
 $\pi $
preserves
$\pi $
preserves
 $(a_1^2)^{c}=a_1^{2r}$
: that is,
$(a_1^2)^{c}=a_1^{2r}$
: that is, $$ \begin{align*}a_1^{2r}=((a_1^2)^{c})^a=((a_1^2)^a)^{(c^a)}=(a_1^2)^{(c^a)}=(a_1^2)^{a_1^{1+2z}c^{2+3d}}=a_1^{2r^{2+3d}},\end{align*} $$
Since
$$ \begin{align*}a_1^{2r}=((a_1^2)^{c})^a=((a_1^2)^a)^{(c^a)}=(a_1^2)^{(c^a)}=(a_1^2)^{a_1^{1+2z}c^{2+3d}}=a_1^{2r^{2+3d}},\end{align*} $$
Since $$ \begin{align*}r^{1+3d}-1\equiv0\,\bigg({\mathrm{mod}}\ \frac n2\bigg). \end{align*} $$
$$ \begin{align*}r^{1+3d}-1\equiv0\,\bigg({\mathrm{mod}}\ \frac n2\bigg). \end{align*} $$
 $r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
, we get
$r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
, we get
 $r^{({1+3d})/2} -1\equiv -1\,({\mathrm {mod}}\ n/2)$
and so
$r^{({1+3d})/2} -1\equiv -1\,({\mathrm {mod}}\ n/2)$
and so
 $\sum _{l=1}^{1+3d}r^{3l}\equiv 0\,({\mathrm {mod}}\ n/2).$
$\sum _{l=1}^{1+3d}r^{3l}\equiv 0\,({\mathrm {mod}}\ n/2).$
-
(ii)
 $\pi $
preserves
$\pi $
preserves
 $c_1^{a_1}=a_1^{2s}c_1$
, that is,
$c_1^{a_1}=a_1^{2s}c_1$
, that is,
 $a_1^{c_1}=a_1^{1-2sr^3}$
: Since we get
$a_1^{c_1}=a_1^{1-2sr^3}$
: Since we get $$ \begin{align*} c_1^a&= (c^a)^3=a_1^{1+2z}c^{2+3d}a_1^{1+2z}c^{2+3d}a_1^{1+2z}c^{2+3d}\\& = a_1^{1+2z}c_1^{1+d}(c^{-1}a_1^{1+2z}c)c_1^{1+d}(c^{-2}a_1^{1+2z}c^2)c_1^d\\& = a_1^{1+2z}c_1^{1+d}a^{2zr}bc_1^{1+d+ m/6}a_1^xbc_1^{m/6}a_1^{2zr^2}c_1^d\\& =a_1^{1+2z}c_1^{1+d}a^{2zr+n2}(a_1^{2u}c_1)^{1+d+m/6}a_1^{-x}c_1^{d+ m/6}a_1^{2zr}\\& = c_1^{2+3d}a^{2z(r+r^2+r^3)+2-2sr^3+r^2-x(1-2sr^3)^{d+ m/6}+ {nm}/{12}}, \end{align*} $$
that is,
$$ \begin{align*} c_1^a&= (c^a)^3=a_1^{1+2z}c^{2+3d}a_1^{1+2z}c^{2+3d}a_1^{1+2z}c^{2+3d}\\& = a_1^{1+2z}c_1^{1+d}(c^{-1}a_1^{1+2z}c)c_1^{1+d}(c^{-2}a_1^{1+2z}c^2)c_1^d\\& = a_1^{1+2z}c_1^{1+d}a^{2zr}bc_1^{1+d+ m/6}a_1^xbc_1^{m/6}a_1^{2zr^2}c_1^d\\& =a_1^{1+2z}c_1^{1+d}a^{2zr+n2}(a_1^{2u}c_1)^{1+d+m/6}a_1^{-x}c_1^{d+ m/6}a_1^{2zr}\\& = c_1^{2+3d}a^{2z(r+r^2+r^3)+2-2sr^3+r^2-x(1-2sr^3)^{d+ m/6}+ {nm}/{12}}, \end{align*} $$
that is, $$ \begin{align*}(a_1^{c_1})^a=a_1^{c_1^a}=a_1^{(1-2sr^3)^{2+3d}}=a_1^{1-2sr^3},\end{align*} $$
Set
$$ \begin{align*}(a_1^{c_1})^a=a_1^{c_1^a}=a_1^{(1-2sr^3)^{2+3d}}=a_1^{1-2sr^3},\end{align*} $$
Set $$ \begin{align*} (1-2sr^3)^{1+3d}-1\equiv0\,({\mathrm{mod}}\ n). \end{align*} $$
$$ \begin{align*} (1-2sr^3)^{1+3d}-1\equiv0\,({\mathrm{mod}}\ n). \end{align*} $$
 $f=2z(r+r^2+r^3)+2-2sr^3+r^2-x(1-2sr^3)^{d+ (m/6)} + {nm}/{12}$
. Then, f is even and
$f=2z(r+r^2+r^3)+2-2sr^3+r^2-x(1-2sr^3)^{d+ (m/6)} + {nm}/{12}$
. Then, f is even and
 $c_1^a=c_1^{2+3d}a_1^f$
. Recalling
$c_1^a=c_1^{2+3d}a_1^f$
. Recalling
 $s\equiv ({r^{-3}-1})/2 + n/4 \,({\mathrm {mod}}\ n/2)$
and
$s\equiv ({r^{-3}-1})/2 + n/4 \,({\mathrm {mod}}\ n/2)$
and
 $x\equiv -r+r^2+ n/4\,({\mathrm {mod}}\ n/2)$
, we get
$x\equiv -r+r^2+ n/4\,({\mathrm {mod}}\ n/2)$
, we get
 $2f\equiv 2(1+2z)(r+r^2+r^3)+ n/2\,({\mathrm {mod}}\ n)$
.
$2f\equiv 2(1+2z)(r+r^2+r^3)+ n/2\,({\mathrm {mod}}\ n)$
.
-
(iii)
 $\pi $
preserves
$\pi $
preserves
 $c_1^b=a_1^{2u}c_1$
, that is,
$c_1^b=a_1^{2u}c_1$
, that is,
 $b^{c_1}=a_1^{2ur^3}b$
: and
$b^{c_1}=a_1^{2ur^3}b$
: and $$ \begin{align*}(b^{c_1})^a=(b^a)^{(c_1^a)}=(a_1^{-1}b)^{(c_1^a)}=a_1^{2ur^3-r^3+n/2-2f}b=a_1^{2ur^3-r^3-2(1+2z)(r+r^2+r^3)}b\end{align*} $$
that is,
$$ \begin{align*}(b^{c_1})^a=(b^a)^{(c_1^a)}=(a_1^{-1}b)^{(c_1^a)}=a_1^{2ur^3-r^3+n/2-2f}b=a_1^{2ur^3-r^3-2(1+2z)(r+r^2+r^3)}b\end{align*} $$
that is, $$ \begin{align*}(a_1^{2ur^3}b)^a=a_1^{2ur^3-1}b,\end{align*} $$
$$ \begin{align*}(a_1^{2ur^3}b)^a=a_1^{2ur^3-1}b,\end{align*} $$
 $$ \begin{align*} 2(1+2z)(r+r^2+r^3)\equiv 1-r^3\,({\mathrm{mod}}\ n). \end{align*} $$
$$ \begin{align*} 2(1+2z)(r+r^2+r^3)\equiv 1-r^3\,({\mathrm{mod}}\ n). \end{align*} $$
-
(iv)
 $\pi $
preserves
$\pi $
preserves
 $a_1^c=bc^{m/2}$
: and
$a_1^c=bc^{m/2}$
: and $$ \begin{align*}(a_1^c)^a=(a_1^a)^{(c^a)}=a_1^{(c^a)}=a^{a_1^{1+2z}c^{2+3d}}=a_1^{x(1-2sr^3)^d+2u\sum_{l=1}^dr^{3l}}bc^{m/2}\end{align*} $$
that is,
$$ \begin{align*}(a_1^c)^a=(a_1^a)^{(c^a)}=a_1^{(c^a)}=a^{a_1^{1+2z}c^{2+3d}}=a_1^{x(1-2sr^3)^d+2u\sum_{l=1}^dr^{3l}}bc^{m/2}\end{align*} $$
that is, $$ \begin{align*}(bc^{m/2})^a=a_1^{-1}b(c_1^a)^{m/6}= a_1^{(2z(r+r^2+r^3)-2sr^3+r^2-x(1-2sr^3)^{d+ m/6}+2)\sum_{l=0}^{ m/6-1}r^{3l}-1} bc^{ m/2},\end{align*} $$
where
$$ \begin{align*}(bc^{m/2})^a=a_1^{-1}b(c_1^a)^{m/6}= a_1^{(2z(r+r^2+r^3)-2sr^3+r^2-x(1-2sr^3)^{d+ m/6}+2)\sum_{l=0}^{ m/6-1}r^{3l}-1} bc^{ m/2},\end{align*} $$
where $$ \begin{align*} \Delta\cdot\sum_{l=0}^{m/6-1}r^{3l}\equiv x(1-2sr^3)^d+2u\sum_{l=1}^dr^{3l}+1\ \pmod {\ n}, \end{align*} $$
$$ \begin{align*} \Delta\cdot\sum_{l=0}^{m/6-1}r^{3l}\equiv x(1-2sr^3)^d+2u\sum_{l=1}^dr^{3l}+1\ \pmod {\ n}, \end{align*} $$
 $\Delta=r^2-2sr^3-x(1-2sr^3)^{d+ m/6}+2z(r+r^2+r^3)+2 $
.
$\Delta=r^2-2sr^3-x(1-2sr^3)^{d+ m/6}+2z(r+r^2+r^3)+2 $
.
-
(v)
 $\pi $
preserves
$\pi $
preserves
 $b^c=a_1^xb$
: and
$b^c=a_1^xb$
: and $$ \begin{align*} (b^c)^a&= (ba_1)^{(c^a)}=(a_1^{-2(1+2z)}ba_1)^{c^{2+3d}}\\&=a_1^{-2(1+2z)r}(ba_1)^{c^{2+3d}}= a_1^{-2(1+2z)r}(a_1^{(x-1+ n/2)r}b)^{c_1^d}\\ &= a_1^{x-1-2(1+2z)r+ n/2+2u\sum_{l=1}^dr^{3l}}b \end{align*} $$
that is,
$$ \begin{align*} (b^c)^a&= (ba_1)^{(c^a)}=(a_1^{-2(1+2z)}ba_1)^{c^{2+3d}}\\&=a_1^{-2(1+2z)r}(ba_1)^{c^{2+3d}}= a_1^{-2(1+2z)r}(a_1^{(x-1+ n/2)r}b)^{c_1^d}\\ &= a_1^{x-1-2(1+2z)r+ n/2+2u\sum_{l=1}^dr^{3l}}b \end{align*} $$
that is, $$ \begin{align*}(a_1^xb)^a=a_1^{x-1}b,\end{align*} $$
(5-35)
$$ \begin{align*}(a_1^xb)^a=a_1^{x-1}b,\end{align*} $$
(5-35) $$ \begin{align} -2(1+2z)r+\frac n2+2u\sum_{l=1}^dr^{3l}\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
$$ \begin{align} -2(1+2z)r+\frac n2+2u\sum_{l=1}^dr^{3l}\equiv 0\,({\mathrm{mod}}\ n). \end{align} $$
-
(vi)
 ${\mathrm {o}}(\pi (c))=m$
: By
${\mathrm {o}}(\pi (c))=m$
: By
 $r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
again, which implies
$r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
again, which implies $$ \begin{align*}\sum_{l=0}^{ m/3-1}r^{3l}=(1+r^{ m/2})\sum_{l=0}^{ m/6-1}r^{3l}\equiv0\,\bigg({\mathrm{mod}}\ \frac n2\bigg),\end{align*} $$
$$ \begin{align*}\sum_{l=0}^{ m/3-1}r^{3l}=(1+r^{ m/2})\sum_{l=0}^{ m/6-1}r^{3l}\equiv0\,\bigg({\mathrm{mod}}\ \frac n2\bigg),\end{align*} $$
 $f\sum _{l=0}^{m/(3-1)}r^{3l}\equiv 0\,({\mathrm {mod}}\ n)$
as f is even. Then, as desired.
$f\sum _{l=0}^{m/(3-1)}r^{3l}\equiv 0\,({\mathrm {mod}}\ n)$
as f is even. Then, as desired. $$ \begin{align*}(c^a)^m=(c_1^a)^{ m/3}=(c_1^{2+3d}a_1^f)^{ m/3}=c_1^{(2+3d) m/3}a_1^{f\sum_{l=0}^{ m/3-1}r^{3l}}=a_1^{f\sum_{l=0}^{ m/3-1}r^{3l}}=1,\end{align*} $$
$$ \begin{align*}(c^a)^m=(c_1^a)^{ m/3}=(c_1^{2+3d}a_1^f)^{ m/3}=c_1^{(2+3d) m/3}a_1^{f\sum_{l=0}^{ m/3-1}r^{3l}}=a_1^{f\sum_{l=0}^{ m/3-1}r^{3l}}=1,\end{align*} $$
-
(vii)
 $\pi ^2={\mathrm {Inn}}(a_1)$
: Recall
$\pi ^2={\mathrm {Inn}}(a_1)$
: Recall
 ${\mathrm {Inn}}(a_1)(c)=c^{1+ (m/2)} a_1^{n/(2-1)}b$
. that is,
${\mathrm {Inn}}(a_1)(c)=c^{1+ (m/2)} a_1^{n/(2-1)}b$
. that is, $$ \begin{align*} &c^{1+ m/2}a_1^{-1+ n/2}b\\&\quad={\mathrm{Inn}}(a_1)(c)=\pi^2(c)=(a^{1+2z}c^{2+3d})^a=a^{1+2z}(c^a)^2 (c_1^{2+3d}a_1^f)^d\\ &\quad=a^{2+4z}c^{2+3d}a^{1+2z}c^{2+3d(3+3d)}a^{f\sum_{l=0}^{d-1}r^{3l}}\\ &\quad=c^{(3d+2)^2+ m/2}a_1^{-3r-1-2z-4zr+({n(2+\sum_{l=0}^{d-1}r^{3l}+(1-2sr^3)^d)})/4 +(2sr^3-(2zr+1)(1+r+r^2))\sum_{l=0}^{d-1}r^{3l}}b, \end{align*} $$
where
$$ \begin{align*} &c^{1+ m/2}a_1^{-1+ n/2}b\\&\quad={\mathrm{Inn}}(a_1)(c)=\pi^2(c)=(a^{1+2z}c^{2+3d})^a=a^{1+2z}(c^a)^2 (c_1^{2+3d}a_1^f)^d\\ &\quad=a^{2+4z}c^{2+3d}a^{1+2z}c^{2+3d(3+3d)}a^{f\sum_{l=0}^{d-1}r^{3l}}\\ &\quad=c^{(3d+2)^2+ m/2}a_1^{-3r-1-2z-4zr+({n(2+\sum_{l=0}^{d-1}r^{3l}+(1-2sr^3)^d)})/4 +(2sr^3-(2zr+1)(1+r+r^2))\sum_{l=0}^{d-1}r^{3l}}b, \end{align*} $$
where $$ \begin{align*} &(1+d)(1+3d)\equiv 0\ \pmod {\ m/3},\\&-3r-2z-4zr+\alpha\cdot\sum_{l=0}^{d-1}r^{3l}\equiv 2+\frac{n(\sum_{l=0}^{d-1}r^{3l}+(1-2sr^3)^d)}4\ \pmod {\ n},\end{align*} $$
$$ \begin{align*} &(1+d)(1+3d)\equiv 0\ \pmod {\ m/3},\\&-3r-2z-4zr+\alpha\cdot\sum_{l=0}^{d-1}r^{3l}\equiv 2+\frac{n(\sum_{l=0}^{d-1}r^{3l}+(1-2sr^3)^d)}4\ \pmod {\ n},\end{align*} $$
 $\alpha=2sr^3-(2zr+1)(1+r+r^2)$
.
$\alpha=2sr^3-(2zr+1)(1+r+r^2)$
.
-
(viii) Ensure
 $\langle c\rangle _X=1$
: Since
$\langle c\rangle _X=1$
: Since
 $\langle c\rangle _X\leq M$
, we get
$\langle c\rangle _X\leq M$
, we get
 $\langle c\rangle _X\leq M_X=\langle a_1^2\rangle \langle c_1\rangle $
, which implies
$\langle c\rangle _X\leq M_X=\langle a_1^2\rangle \langle c_1\rangle $
, which implies
 $\langle c\rangle _X=\cap _{x\in X}\langle c\rangle ^x=\cap _{x\in G}\langle c\rangle ^x=\cap _{x\in G}\langle c_1\rangle ^x=\langle c_1\rangle _{X_1}$
. Then, it is sufficient to ensure
$\langle c\rangle _X=\cap _{x\in X}\langle c\rangle ^x=\cap _{x\in G}\langle c\rangle ^x=\cap _{x\in G}\langle c_1\rangle ^x=\langle c_1\rangle _{X_1}$
. Then, it is sufficient to ensure
 $\langle c^3\rangle _{X_1}=1$
.
$\langle c^3\rangle _{X_1}=1$
.Recall
where $$ \begin{align*}X_1=\langle a,b,c_1|R,(a_1)^{c_1}=a_1^{r^3}, c_1^b=a_1^{2u}c_1, (c_1)^a=c_1^{2+3d}a_1^{i}\rangle ,\end{align*} $$
$$ \begin{align*}X_1=\langle a,b,c_1|R,(a_1)^{c_1}=a_1^{r^3}, c_1^b=a_1^{2u}c_1, (c_1)^a=c_1^{2+3d}a_1^{i}\rangle ,\end{align*} $$
 $r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2),\,2u\equiv ({r^3-1})/{r^2}\,({\mathrm {mod}}\ n/2)$
and
$r^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2),\,2u\equiv ({r^3-1})/{r^2}\,({\mathrm {mod}}\ n/2)$
and
 $i\equiv ({3(1-r^3)}/2) + n/4 \,({\mathrm {mod}}\ n/2)$
. By (5-35), we get that u is even. Noting
$i\equiv ({3(1-r^3)}/2) + n/4 \,({\mathrm {mod}}\ n/2)$
. By (5-35), we get that u is even. Noting
 $\langle c_1\rangle _{X_1}=1$
and
$\langle c_1\rangle _{X_1}=1$
and
 $(u, n/4)=1$
, by Lemma 5.1, Note that
$(u, n/4)=1$
, by Lemma 5.1, Note that $$ \begin{align*}\sum_{l=1}^{j}r^{3l}\equiv0 \,\bigg({\mathrm{mod}}\ \frac n4\bigg)~\mathrm{if~and~only~if}~ j\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg).\end{align*} $$
$$ \begin{align*}\sum_{l=1}^{j}r^{3l}\equiv0 \,\bigg({\mathrm{mod}}\ \frac n4\bigg)~\mathrm{if~and~only~if}~ j\equiv0 \,\bigg({\mathrm{mod}}\ \frac m3\bigg).\end{align*} $$
 $\sum _{i=1}^{1+3d}r^{3i}\equiv 0\,({\mathrm {mod}}\ n/2)$
. Thus,
$\sum _{i=1}^{1+3d}r^{3i}\equiv 0\,({\mathrm {mod}}\ n/2)$
. Thus,
 $1+3d\equiv 0\,({\mathrm {mod}}\ m/3)$
. Since
$1+3d\equiv 0\,({\mathrm {mod}}\ m/3)$
. Since
 $1+3d\ne 0$
, we get
$1+3d\ne 0$
, we get
 $1+3d$
is either
$1+3d$
is either
 $m/3$
or
$m/3$
or
 ${2m}/3$
. By (5-35), we get
${2m}/3$
. By (5-35), we get
 $1+2z\equiv ({1-r})/{2r} \,({\mathrm {mod}}\ n/2)$
.
$1+2z\equiv ({1-r})/{2r} \,({\mathrm {mod}}\ n/2)$
.
5.5
 $M=\langle a^3\rangle \langle c\rangle $
and
$M=\langle a^3\rangle \langle c\rangle $
and
 $X/M_X\cong S_4$
$X/M_X\cong S_4$
Lemma 5.6. Suppose that
![]() $X=X(Q)$
,
$X=X(Q)$
,
![]() $M=\langle a^3\rangle \langle c\rangle $
,
$M=\langle a^3\rangle \langle c\rangle $
,
![]() $X/M_X\cong S_4$
and
$X/M_X\cong S_4$
and
![]() $\langle c\rangle _X=1$
. Then,
$\langle c\rangle _X=1$
. Then,
where
![]() $m\equiv 4\,({\mathrm {mod}}\ 8)$
and r is of order
$m\equiv 4\,({\mathrm {mod}}\ 8)$
and r is of order
![]() $m/4$
in
$m/4$
in
![]() ${\mathbb {Z}}_{2n}^*$
.
${\mathbb {Z}}_{2n}^*$
.
In this subsection,
![]() $M_X=\langle a^3\rangle \langle c^4\rangle $
. Set
$M_X=\langle a^3\rangle \langle c^4\rangle $
. Set
![]() $a^3=a_1$
and
$a^3=a_1$
and
![]() $c^4=c_1$
so that
$c^4=c_1$
so that
![]() $M_X=\langle a_1\rangle \langle c_1\rangle $
. Set
$M_X=\langle a_1\rangle \langle c_1\rangle $
. Set
![]() ${\mathrm {o}}(a)=2n$
and
${\mathrm {o}}(a)=2n$
and
![]() ${\mathrm {o}}(c)=m$
, where
${\mathrm {o}}(c)=m$
, where
![]() $3| n$
and
$3| n$
and
![]() $4| m$
. Then, we show
$4| m$
. Then, we show
![]() $\langle a_1\rangle \lhd X$
in Lemma 5.7 and get the classification of X in Lemma 5.8.
$\langle a_1\rangle \lhd X$
in Lemma 5.7 and get the classification of X in Lemma 5.8.
Lemma 5.7.
![]() $\langle a_1\rangle \lhd X$
.
$\langle a_1\rangle \lhd X$
.
Proof. Let
![]() $X_1=M_XG$
. Since
$X_1=M_XG$
. Since
![]() $\langle a\rangle \langle c_1\rangle \leq X_1$
and
$\langle a\rangle \langle c_1\rangle \leq X_1$
and
![]() $\langle c_1\rangle _{X_1}=1$
, the subgroup
$\langle c_1\rangle _{X_1}=1$
, the subgroup
![]() $X_1$
has been given in Lemma 5.1:
$X_1$
has been given in Lemma 5.1:
 $$ \begin{align*}&r^{\frac m4}\equiv r^{t-1}\equiv r^{v-1}\equiv1\ \pmod {\ n},\,t^2\equiv 1\ \pmod {\ m/4},\\&6s\sum_{l=1}^tr^{l}+6sr\equiv 6sr+6s\sum_{l=1}^{v}r^l-3u\sum_{l=1}^tr^l+3ur\equiv 2(1-r)\ \pmod {\ 2n},\\&6s\sum_{l=1}^{w}r^l\equiv 3u\sum_{l=1}^{w}\bigg(1-3s\bigg(\sum_{l=1}^tr^l+r\bigg)\bigg)^l\equiv 0\ \pmod {\ 2n}\end{align*} $$
$$ \begin{align*}&r^{\frac m4}\equiv r^{t-1}\equiv r^{v-1}\equiv1\ \pmod {\ n},\,t^2\equiv 1\ \pmod {\ m/4},\\&6s\sum_{l=1}^tr^{l}+6sr\equiv 6sr+6s\sum_{l=1}^{v}r^l-3u\sum_{l=1}^tr^l+3ur\equiv 2(1-r)\ \pmod {\ 2n},\\&6s\sum_{l=1}^{w}r^l\equiv 3u\sum_{l=1}^{w}\bigg(1-3s\bigg(\sum_{l=1}^tr^l+r\bigg)\bigg)^l\equiv 0\ \pmod {\ 2n}\end{align*} $$
only when
![]() $w\equiv 0\ \pmod {\ m/4} $
, and moreover, if
$w\equiv 0\ \pmod {\ m/4} $
, and moreover, if
![]() $2\mid n$
, then
$2\mid n$
, then
![]() $3u(\sum_{l=0}^{v-1}r^l-1)\equiv 0 \pmod {\ 2n}\ \textrm{and}\ v^2\equiv 1\ \pmod {\ m/4}$
;if
$3u(\sum_{l=0}^{v-1}r^l-1)\equiv 0 \pmod {\ 2n}\ \textrm{and}\ v^2\equiv 1\ \pmod {\ m/4}$
;if
![]() $2\nmid n$
, then
$2\nmid n$
, then
![]() $3u\sum_{l=1}^vr^l-3ur\equiv 6sr+(n-1)(1-r) \pmod {\ 2n}\ \textrm{and}\ v^2\equiv t\ \pmod {\ m/4}$
; and if
$3u\sum_{l=1}^vr^l-3ur\equiv 6sr+(n-1)(1-r) \pmod {\ 2n}\ \textrm{and}\ v^2\equiv t\ \pmod {\ m/4}$
; and if
![]() $t\ne 1$
, then
$t\ne 1$
, then
![]() $u$
is even.
$u$
is even.
Now
![]() $X=\langle X_1, c\rangle $
. Since
$X=\langle X_1, c\rangle $
. Since
![]() $X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong S_4$
, the only possibility under our conditions is
$X/M_X=\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle \cong S_4$
, the only possibility under our conditions is
where
![]() $i\in {\mathbb {Z}}_3$
. Observing (5-37) and (5-38), we may relabel
$i\in {\mathbb {Z}}_3$
. Observing (5-37) and (5-38), we may relabel
![]() $a^{i+3x}b$
by b for some
$a^{i+3x}b$
by b for some
![]() $x\in {\mathbb {Z}}$
. Then, in the perimage X, (5-38) corresponds to
$x\in {\mathbb {Z}}$
. Then, in the perimage X, (5-38) corresponds to
Set
necessarily, z is odd, as
![]() ${\mathrm {o}}(a_1)$
is even. Then, X is uniquely determined by (5-37), (5-39) and (5-40). To show
${\mathrm {o}}(a_1)$
is even. Then, X is uniquely determined by (5-37), (5-39) and (5-40). To show
![]() $\langle a_1\rangle \lhd X$
, for the contrary, we assume
$\langle a_1\rangle \lhd X$
, for the contrary, we assume
![]() $d\ne 0$
. Then we need to deal with two cases according to the parameter t of
$d\ne 0$
. Then we need to deal with two cases according to the parameter t of
![]() $X_1$
, separately.
$X_1$
, separately.
Case 1:
![]() $t=1$
. In this case, we get
$t=1$
. In this case, we get
![]() $v=1$
and
$v=1$
and
![]() $1-6sr-r\equiv 0\,({\mathrm {mod}}\ n)$
by
$1-6sr-r\equiv 0\,({\mathrm {mod}}\ n)$
by
![]() $X_1$
. Set
$X_1$
. Set
![]() $r_1=1-6sr$
. Then,
$r_1=1-6sr$
. Then,
![]() $r_1\equiv 1\,({\mathrm {mod}}\ 6),\,a^{c_1}=a^{r_1}$
and
$r_1\equiv 1\,({\mathrm {mod}}\ 6),\,a^{c_1}=a^{r_1}$
and
![]() $b^{c_1}=a_1^{ur_1}b$
. By (5-37), (5-39) and (5-40), we get
$b^{c_1}=a_1^{ur_1}b$
. By (5-37), (5-39) and (5-40), we get
![]() $b^c=a^{2+3x}bc^{4y}$
for some x and y.
$b^c=a^{2+3x}bc^{4y}$
for some x and y.
Since c preserves
![]() $a_1^{c_1}=a_1^{r_1}$
,
$a_1^{c_1}=a_1^{r_1}$
,
and
which gives
![]() $d\equiv dr_1\,({\mathrm {mod}}\ m/4).$
Since c preserves
$d\equiv dr_1\,({\mathrm {mod}}\ m/4).$
Since c preserves
![]() $b^{c_1}=a_1^{ur_1}b$
, there exists some x such that
$b^{c_1}=a_1^{ur_1}b$
, there exists some x such that
![]() $(b^{c_1})^c=a^{(2+3x+3u)r_1}bc^{4y}$
and
$(b^{c_1})^c=a^{(2+3x+3u)r_1}bc^{4y}$
and
![]() $(a_1^{ur_1}b)^c=c_1^{dur_1}a_1^{z\sum _{l=1}^{ur_1}r_1^{dl}}a^{2+3x}bc^{4y},$
which gives
$(a_1^{ur_1}b)^c=c_1^{dur_1}a_1^{z\sum _{l=1}^{ur_1}r_1^{dl}}a^{2+3x}bc^{4y},$
which gives
![]() $du\equiv 0\,({\mathrm {mod}}\ m/4).$
Since
$du\equiv 0\,({\mathrm {mod}}\ m/4).$
Since
![]() $a_1^{c_1}=a_1^{c^4}=a_1^{x_1}c_1^{d(z^3+z^2+z+1)}$
for some
$a_1^{c_1}=a_1^{c^4}=a_1^{x_1}c_1^{d(z^3+z^2+z+1)}$
for some
![]() $x_1$
,
$x_1$
,
By (5-39), we get
![]() $ac=cbc^{3+4w}$
. Then,
$ac=cbc^{3+4w}$
. Then,
![]() $a_1^{ac}=a_1^z c_1^d$
and
$a_1^{ac}=a_1^z c_1^d$
and
![]() $a_1^{cbc^{3+4w}}=a_1^{x_1}c_1^{d-dz(z^2+z+1)},$
which gives
$a_1^{cbc^{3+4w}}=a_1^{x_1}c_1^{d-dz(z^2+z+1)},$
which gives
With (5-41), we know
![]() $d\equiv 0\,({\mathrm {mod}}\ m/4),$
which contradicts
$d\equiv 0\,({\mathrm {mod}}\ m/4),$
which contradicts
![]() $d=0$
.
$d=0$
.
Case 2:
![]() $t\ne 1$
. In this case, we have
$t\ne 1$
. In this case, we have
![]() $t\ne 1$
. If
$t\ne 1$
. If
![]() $\langle a_1^2\rangle \lhd X$
, then by considering
$\langle a_1^2\rangle \lhd X$
, then by considering
![]() ${\overline X}=X/\langle a_1^2\rangle $
and
${\overline X}=X/\langle a_1^2\rangle $
and
![]() $\langle \overline {c_1}\rangle \lhd {\overline X}$
, one may get
$\langle \overline {c_1}\rangle \lhd {\overline X}$
, one may get
![]() $t=1$
, which is a contradiction, as
$t=1$
, which is a contradiction, as
![]() $\langle {\overline c}\rangle \leq C_{{\overline X}}(\langle \overline {c_1}\rangle )={\overline X}$
. In what follows, we show
$\langle {\overline c}\rangle \leq C_{{\overline X}}(\langle \overline {c_1}\rangle )={\overline X}$
. In what follows, we show
![]() $\langle a_1^2\rangle \lhd X$
.
$\langle a_1^2\rangle \lhd X$
.
By (5-37), u is even and
![]() $r\equiv 1\,({\mathrm {mod}}\ 3)$
. By using (5-37), (5-39) and (5-40), we get
$r\equiv 1\,({\mathrm {mod}}\ 3)$
. By using (5-37), (5-39) and (5-40), we get
![]() $b^c=a^{2+6x}bc_1^y$
for some x and y, omitting the details.
$b^c=a^{2+6x}bc_1^y$
for some x and y, omitting the details.
Since c preserves
![]() $(a_1^2)^{c_1}=a_1^{2r}$
, there exists some
$(a_1^2)^{c_1}=a_1^{2r}$
, there exists some
![]() $x_1$
such that
$x_1$
such that
![]() $((a_1^2)^{c_1})^c=a_1^{x_1}c_1^{d(t+1)}$
and
$((a_1^2)^{c_1})^c=a_1^{x_1}c_1^{d(t+1)}$
and
![]() $(a_1^{2r})^c=a_1^{x_1}c_1^{d(t+1)r},$
which gives
$(a_1^{2r})^c=a_1^{x_1}c_1^{d(t+1)r},$
which gives
![]() $d(t+1)(r-1)\equiv 0\,({\mathrm {mod}}\ m/4).$
Since c preserves
$d(t+1)(r-1)\equiv 0\,({\mathrm {mod}}\ m/4).$
Since c preserves
![]() $(c_1)^b=a_1^{u}c_1^v$
, there exists some
$(c_1)^b=a_1^{u}c_1^v$
, there exists some
![]() $x_1$
such that
$x_1$
such that
![]() $(c_1^b)^c=a_1^{x_1}c_1^v$
and
$(c_1^b)^c=a_1^{x_1}c_1^v$
and
![]() $(a_1^{u}c_1^v)^c=a_1^{x_1}c_1^{({du(t+1)}/2) +v},$
which gives
$(a_1^{u}c_1^v)^c=a_1^{x_1}c_1^{({du(t+1)}/2) +v},$
which gives
![]() ${du(t+1)}/2 \equiv 0\,({\mathrm {mod}}\ m/4).$
Since c preserves
${du(t+1)}/2 \equiv 0\,({\mathrm {mod}}\ m/4).$
Since c preserves
![]() $c_1^a=a_1^{2s}c_1^t$
, we get
$c_1^a=a_1^{2s}c_1^t$
, we get
![]() $c_1^{bc^{2+4w}}=a_1^{2s}c_1^t$
. Then, there exists some
$c_1^{bc^{2+4w}}=a_1^{2s}c_1^t$
. Then, there exists some
![]() $x_1$
such that
$x_1$
such that
![]() $c_1^{bc^{2+4w}}=(a_1^{u}c_1^{v})^{c^{2+4w}}=((a_1^zc_1^d)^zc_1^d)^{ur^w}c_1^{v}=a_1^{x_1}c_1^{v},$
which gives
$c_1^{bc^{2+4w}}=(a_1^{u}c_1^{v})^{c^{2+4w}}=((a_1^zc_1^d)^zc_1^d)^{ur^w}c_1^{v}=a_1^{x_1}c_1^{v},$
which gives
![]() $v\equiv t\,({\mathrm {mod}}\ m/4).$
By (5-39) again, we get
$v\equiv t\,({\mathrm {mod}}\ m/4).$
By (5-39) again, we get
![]() $ac^2=cbc^{4(w+1)}$
. Then there exists some
$ac^2=cbc^{4(w+1)}$
. Then there exists some
![]() $x_1$
such that
$x_1$
such that
![]() $(a_1^2)^{ac^2}=a_1^{x_1}c_1^{d(t+1)(z+1)}$
and
$(a_1^2)^{ac^2}=a_1^{x_1}c_1^{d(t+1)(z+1)}$
and
![]() $(a_1^2)^{cbc_1^{w+1}}=a_1^{x_1}c_1^{d(t+1)},$
which gives
$(a_1^2)^{cbc_1^{w+1}}=a_1^{x_1}c_1^{d(t+1)},$
which gives
Since
![]() ${\mathrm {o}}(a_1^c)= {2n}/3$
, we get
${\mathrm {o}}(a_1^c)= {2n}/3$
, we get
![]() ${dn(t+1)}/3 \equiv 0\,({\mathrm {mod}}\ m/4)$
. With
${dn(t+1)}/3 \equiv 0\,({\mathrm {mod}}\ m/4)$
. With
![]() $(n/3, z)=1$
and (5-42), we get
$(n/3, z)=1$
and (5-42), we get
![]() $d(t+1)\equiv 0\,({\mathrm {mod}}\ m/4)$
. Then,
$d(t+1)\equiv 0\,({\mathrm {mod}}\ m/4)$
. Then,
![]() $(a_1^2)^c=(a_1^zc_1^d)^2=a_1^{x_1}c_1^{d(t+1)}=a_1^{x_1}$
for some
$(a_1^2)^c=(a_1^zc_1^d)^2=a_1^{x_1}c_1^{d(t+1)}=a_1^{x_1}$
for some
![]() $x_1$
, which implies
$x_1$
, which implies
![]() $\langle a_1^2\rangle \lhd X$
, as desired.
$\langle a_1^2\rangle \lhd X$
, as desired.
Lemma 5.8. The group X is given by (5-36).
Proof. By the lemma,
![]() $\langle a_1\rangle \lhd X$
, that is,
$\langle a_1\rangle \lhd X$
, that is,
![]() $(a_1)^c=a_1^z$
by (5-40). Since
$(a_1)^c=a_1^z$
by (5-40). Since
![]() $\langle a^2\rangle \lhd X_1$
, we get
$\langle a^2\rangle \lhd X_1$
, we get
![]() $\langle a\rangle \lhd X_1$
and so
$\langle a\rangle \lhd X_1$
and so
![]() $G\lhd X_1$
, that is,
$G\lhd X_1$
, that is,
![]() $t=v=1$
in (5-37). Then, by (5-37), (5-39) and (5-40), we can set
$t=v=1$
in (5-37). Then, by (5-37), (5-39) and (5-40), we can set
![]() $X=\langle a,b,c\mid R,a^{c_1}=a^{r_1},b^{c_1}=a_1^{ur_1}b,(a_1)^c=a_1^z,a^c=bc^{3+4w}\rangle ,$
where
$X=\langle a,b,c\mid R,a^{c_1}=a^{r_1},b^{c_1}=a_1^{ur_1}b,(a_1)^c=a_1^z,a^c=bc^{3+4w}\rangle ,$
where
![]() $r_1=1-6sr,\,r_1^{m/4} - 1\equiv 2(r_1-r)\equiv 0\,({\mathrm {mod}}\ 2n)$
and
$r_1=1-6sr,\,r_1^{m/4} - 1\equiv 2(r_1-r)\equiv 0\,({\mathrm {mod}}\ 2n)$
and
![]() $2s\sum _{l=1}^jr^l\equiv 0\equiv u\sum _{l=1}^jr_1^l\,({\mathrm {mod}}\ {2n}/3)$
if and only if
$2s\sum _{l=1}^jr^l\equiv 0\equiv u\sum _{l=1}^jr_1^l\,({\mathrm {mod}}\ {2n}/3)$
if and only if
![]() $j\equiv 0\,({\mathrm {mod}}\ m/4).$
Note that
$j\equiv 0\,({\mathrm {mod}}\ m/4).$
Note that
![]() $2s\sum _{l=1}^jr^l\equiv 0\,({\mathrm {mod}}\ {2n}/3)$
if and only if
$2s\sum _{l=1}^jr^l\equiv 0\,({\mathrm {mod}}\ {2n}/3)$
if and only if
![]() $r_1^j-1\equiv 0\,({\mathrm {mod}}\ 2n)$
.
$r_1^j-1\equiv 0\,({\mathrm {mod}}\ 2n)$
.
In what follows, we divide the proof into two steps.
Step 1: Show
![]() $m\equiv 4\,({\mathrm {mod}}\ 8)$
. Set
$m\equiv 4\,({\mathrm {mod}}\ 8)$
. Set
![]() $\langle c\rangle =\langle c_2\rangle \times \langle c_3\rangle $
, where
$\langle c\rangle =\langle c_2\rangle \times \langle c_3\rangle $
, where
![]() $\langle c_2\rangle $
is a
$\langle c_2\rangle $
is a
![]() $2$
-group and
$2$
-group and
![]() $\langle c_3\rangle $
is the
$\langle c_3\rangle $
is the
![]() $2'-$
Hall subgroup of
$2'-$
Hall subgroup of
![]() $\langle c\rangle $
. Then,
$\langle c\rangle $
. Then,
![]() $\langle c_1\rangle =\langle c_2^4\rangle \times \langle c_3\rangle $
. To show
$\langle c_1\rangle =\langle c_2^4\rangle \times \langle c_3\rangle $
. To show
![]() $m\equiv 4\,({\mathrm {mod}}\ 8)$
, we only need to show
$m\equiv 4\,({\mathrm {mod}}\ 8)$
, we only need to show
![]() $c_2^4=1$
.
$c_2^4=1$
.
Consider
![]() ${\overline X}=X/\langle a_1\rangle =\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle $
. Then,
${\overline X}=X/\langle a_1\rangle =\langle {\overline a},{\overline b}\rangle \langle {\overline c}\rangle $
. Then,
![]() $C_{{\overline X}}(\langle {\overline c}_1\rangle )={\overline X}$
, which implies
$C_{{\overline X}}(\langle {\overline c}_1\rangle )={\overline X}$
, which implies
![]() $\langle \overline {c_1}\rangle \le Z({\overline X})$
and
$\langle \overline {c_1}\rangle \le Z({\overline X})$
and
![]() ${\overline X}/\langle \overline {c_1}\rangle {\kern-1pt}\cong{\kern-1pt} S_4$
. Note that
${\overline X}/\langle \overline {c_1}\rangle {\kern-1pt}\cong{\kern-1pt} S_4$
. Note that
![]() $\langle {\overline c}_3\rangle {\kern-1.2pt}\leq{\kern-1.2pt} \langle {\overline c}_3\rangle (\langle {\overline c}_2^4\rangle \langle {\overline a}\rangle ){\kern-1.2pt}\leq{\kern-1.2pt} {\overline X}$
, where
$\langle {\overline c}_3\rangle {\kern-1.2pt}\leq{\kern-1.2pt} \langle {\overline c}_3\rangle (\langle {\overline c}_2^4\rangle \langle {\overline a}\rangle ){\kern-1.2pt}\leq{\kern-1.2pt} {\overline X}$
, where
![]() $(|{\overline X}{\kern-1.2pt}:{\kern-1.2pt}\langle {\overline c}_3\rangle (\langle {\overline c}_2^4\rangle \langle {\overline a}\rangle )|, |\langle {\overline c}_3\rangle |){\kern-1pt}={\kern-1pt}1$
. Thus, by Proposition 2.7, we get that
$(|{\overline X}{\kern-1.2pt}:{\kern-1.2pt}\langle {\overline c}_3\rangle (\langle {\overline c}_2^4\rangle \langle {\overline a}\rangle )|, |\langle {\overline c}_3\rangle |){\kern-1pt}={\kern-1pt}1$
. Thus, by Proposition 2.7, we get that
![]() $\langle {\overline c}_3\rangle $
has a complement in
$\langle {\overline c}_3\rangle $
has a complement in
![]() ${\overline X}$
, which implies
${\overline X}$
, which implies
![]() $X=(\langle a,b\rangle \langle c_2\rangle )\rtimes \langle c_3\rangle .$
$X=(\langle a,b\rangle \langle c_2\rangle )\rtimes \langle c_3\rangle .$
Consider
![]() $X_2=\langle a,b\rangle \langle c_2\rangle $
, where
$X_2=\langle a,b\rangle \langle c_2\rangle $
, where
![]() $\langle c_2\rangle _{X_2}=1$
and
$\langle c_2\rangle _{X_2}=1$
and
![]() $\langle a_1\rangle \lhd X_2$
, and
$\langle a_1\rangle \lhd X_2$
, and
![]() $\overline {X_2}=X_2/\langle a_1\rangle =\langle {\overline a},{\overline b}\rangle \langle \overline {c_2}\rangle $
. Note that
$\overline {X_2}=X_2/\langle a_1\rangle =\langle {\overline a},{\overline b}\rangle \langle \overline {c_2}\rangle $
. Note that
![]() $\langle \overline {c_2}^4\rangle \lhd \overline {X_2}$
. Then,
$\langle \overline {c_2}^4\rangle \lhd \overline {X_2}$
. Then,
![]() $C_{{\overline X}_2}(\langle \overline {c_2}^4\rangle )={\overline X}_2$
, which implies that
$C_{{\overline X}_2}(\langle \overline {c_2}^4\rangle )={\overline X}_2$
, which implies that
![]() ${\overline X}_2$
is the central expansion of
${\overline X}_2$
is the central expansion of
![]() $S_4$
. By Lemma 2.8, we get the Schur multiplier of
$S_4$
. By Lemma 2.8, we get the Schur multiplier of
![]() $S_4$
is
$S_4$
is
![]() ${\mathbb {Z}}_2$
, and then
${\mathbb {Z}}_2$
, and then
![]() ${\mathrm {o}}(c_2)$
is either
${\mathrm {o}}(c_2)$
is either
![]() $4$
or
$4$
or
![]() $8$
. Arguing by contradiction, assume that
$8$
. Arguing by contradiction, assume that
![]() ${\mathrm {o}}(c_2)=8$
. Then,
${\mathrm {o}}(c_2)=8$
. Then,
![]() $c_2^4$
normalizes G, and we set
$c_2^4$
normalizes G, and we set
![]() $a^{c_2^4}=a^i$
, where
$a^{c_2^4}=a^i$
, where
![]() $i\equiv 1\,({\mathrm {mod}}\ 3).$
Note that
$i\equiv 1\,({\mathrm {mod}}\ 3).$
Note that
![]() $\langle a\rangle \leq C_{X_2}(\langle a_1\rangle )\lhd X_2$
. Then,
$\langle a\rangle \leq C_{X_2}(\langle a_1\rangle )\lhd X_2$
. Then,
![]() $\langle a,bc_2,c_2^2\rangle \leq C_{X_2}(\langle a_1\rangle ),$
which implies
$\langle a,bc_2,c_2^2\rangle \leq C_{X_2}(\langle a_1\rangle ),$
which implies
![]() $\langle a_1\rangle \times \langle c_2^4\rangle \lhd X_2$
. Since
$\langle a_1\rangle \times \langle c_2^4\rangle \lhd X_2$
. Since
![]() $i\equiv 1\,({\mathrm {mod}}\ {2n}/3)$
and
$i\equiv 1\,({\mathrm {mod}}\ {2n}/3)$
and
![]() $i^2\equiv 1\,({\mathrm {mod}}\ 2n)$
, we get
$i^2\equiv 1\,({\mathrm {mod}}\ 2n)$
, we get
![]() $i=1$
, which implies
$i=1$
, which implies
![]() $[a,c_2^4]=1$
. Then we have
$[a,c_2^4]=1$
. Then we have
![]() $\langle a,c_2^2\rangle \leq C_{X_2}(\langle a_1\rangle \times \langle c_2^4\rangle )\lhd X_2$
, which implies
$\langle a,c_2^2\rangle \leq C_{X_2}(\langle a_1\rangle \times \langle c_2^4\rangle )\lhd X_2$
, which implies
![]() $bc_2\in C_{X_2}(\langle a_1\rangle \times \langle c_2^4\rangle )$
. So
$bc_2\in C_{X_2}(\langle a_1\rangle \times \langle c_2^4\rangle )$
. So
![]() $(c_2^4)^b=c_2^4$
. Then,
$(c_2^4)^b=c_2^4$
. Then,
![]() $c_2^4\lhd X_2$
is a contradiction. So
$c_2^4\lhd X_2$
is a contradiction. So
![]() ${\mathrm {o}}(c_2)=4$
, which implies
${\mathrm {o}}(c_2)=4$
, which implies
![]() $\langle c_1\rangle =\langle c_3\rangle $
. Then,
$\langle c_1\rangle =\langle c_3\rangle $
. Then,
![]() $X=(\langle a,b\rangle \langle c_2\rangle )\rtimes \langle c_1\rangle .$
$X=(\langle a,b\rangle \langle c_2\rangle )\rtimes \langle c_1\rangle .$
Step 2: Determine the parameters
![]() $r_1,u,w$
and z. In
$r_1,u,w$
and z. In
![]() $X_2=\langle a,b\rangle \langle c_2\rangle $
, we know
$X_2=\langle a,b\rangle \langle c_2\rangle $
, we know
![]() $a^{c_2}=bc_2^3$
by (5-39). Consider
$a^{c_2}=bc_2^3$
by (5-39). Consider
![]() $\langle a\rangle \leq C_{X_2}(\langle a_1\rangle )\lhd X_2$
. Then,
$\langle a\rangle \leq C_{X_2}(\langle a_1\rangle )\lhd X_2$
. Then,
![]() $C_{X_2}(\langle a_1\rangle )$
is either
$C_{X_2}(\langle a_1\rangle )$
is either
![]() $\langle a,bc_2,c_2^2\rangle $
or
$\langle a,bc_2,c_2^2\rangle $
or
![]() $X_2$
.
$X_2$
.
Suppose that
![]() $C_{X_2}(\langle a_1\rangle )=X$
. Then we know
$C_{X_2}(\langle a_1\rangle )=X$
. Then we know
![]() $a_1=b^2$
as
$a_1=b^2$
as
![]() $[a_1,b]=1$
, that is,
$[a_1,b]=1$
, that is,
![]() $n=3$
and
$n=3$
and
![]() $a_1=a_1^{-1}$
. Then,
$a_1=a_1^{-1}$
. Then,
![]() $m=4$
and
$m=4$
and
![]() $z,r=1$
, as desired.
$z,r=1$
, as desired.
Suppose that
![]() $C_{X_2}(\langle a_1\rangle )=\langle a,bc_2,c_2^2\rangle $
. Then,
$C_{X_2}(\langle a_1\rangle )=\langle a,bc_2,c_2^2\rangle $
. Then,
![]() $a_1=a_1^{bc_2}=(a_1^{-1})^{c_2}$
, which implies
$a_1=a_1^{bc_2}=(a_1^{-1})^{c_2}$
, which implies
![]() $z=-1$
. In
$z=-1$
. In
![]() $X_1=\langle a,b\rangle \rtimes \langle c_1\rangle $
, we know
$X_1=\langle a,b\rangle \rtimes \langle c_1\rangle $
, we know
![]() $X_1=\langle a,b,c_1\mid R,a^{c_1}=a^{r_1},b^{c_1}=a_1^{ur_1}b\rangle $
. Since
$X_1=\langle a,b,c_1\mid R,a^{c_1}=a^{r_1},b^{c_1}=a_1^{ur_1}b\rangle $
. Since
![]() $c_2$
preserves
$c_2$
preserves
![]() $a^{c_1}=a^{r_1}$
, we get
$a^{c_1}=a^{r_1}$
, we get
which gives
Recall that
![]() $r_1^j-1\equiv 0\equiv 3u\sum _{l=1}^jr_1^l\,({\mathrm {mod}}\ 2n)$
if and only if
$r_1^j-1\equiv 0\equiv 3u\sum _{l=1}^jr_1^l\,({\mathrm {mod}}\ 2n)$
if and only if
![]() $j\equiv 0\,({\mathrm {mod}}\ m/4)$
. Then, we get that
$j\equiv 0\,({\mathrm {mod}}\ m/4)$
. Then, we get that
![]() $r_1^j-1\equiv 0\,({\mathrm {mod}}\ 2n)$
if and only if
$r_1^j-1\equiv 0\,({\mathrm {mod}}\ 2n)$
if and only if
![]() $j\equiv 0\,({\mathrm {mod}}\ m/4)$
, which implies
$j\equiv 0\,({\mathrm {mod}}\ m/4)$
, which implies
![]() ${\mathrm {o}}(r_1)= m/4$
. For the purpose of formatting uniformity, replacing
${\mathrm {o}}(r_1)= m/4$
. For the purpose of formatting uniformity, replacing
![]() $r_1$
by r, then we get (5-36), as desired.
$r_1$
by r, then we get (5-36), as desired.
6 Proof of Theorem 1.4
As mentioned before, the group
![]() $X(D)$
where
$X(D)$
where
![]() $\langle c\rangle _{X(D)}=1$
has been classified in [Reference Hu, Kovács and Kwon8, Theorem 1.2] by using computational methods in skew morphisms. Actually, with almost the same methods as those that we used in
$\langle c\rangle _{X(D)}=1$
has been classified in [Reference Hu, Kovács and Kwon8, Theorem 1.2] by using computational methods in skew morphisms. Actually, with almost the same methods as those that we used in
![]() $X(Q)$
, we may get a classification of
$X(Q)$
, we may get a classification of
![]() $X(D)$
, which has a different representation from [Reference Hu, Kovács and Kwon8, Theorem 1.2]. Here is our classification. One may see [Reference Du, Luo and Yu4] for its computation in detail.
$X(D)$
, which has a different representation from [Reference Hu, Kovács and Kwon8, Theorem 1.2]. Here is our classification. One may see [Reference Du, Luo and Yu4] for its computation in detail.
Lemma 6.1. Let
![]() $G=D$
and
$G=D$
and
![]() $X=X(D)=G\langle c\rangle $
, where
$X=X(D)=G\langle c\rangle $
, where
![]() $m={\mathrm {o}}(c)\ge 2$
,
$m={\mathrm {o}}(c)\ge 2$
,
![]() $G\cap \langle c\rangle =1$
and
$G\cap \langle c\rangle =1$
and
![]() $\langle c\rangle _X=1.$
Set
$\langle c\rangle _X=1.$
Set
![]() $R:=\{a^{n}=b^2=c^m=1,\,a^b=a^{-1}\}$
. Then one of the following holds.
$R:=\{a^{n}=b^2=c^m=1,\,a^b=a^{-1}\}$
. Then one of the following holds.
-
(1)
 $X{\kern-1pt}={\kern-1pt}\langle a,b,c\mid R, (a^2)^c{\kern-1pt}={\kern-1pt}a^{2r}, c^a{\kern-1pt}={\kern-1pt}a^{2s}c^t,c^b{\kern-1pt}={\kern-1pt}a^uc^v\rangle ,$
whereif and only if
$X{\kern-1pt}={\kern-1pt}\langle a,b,c\mid R, (a^2)^c{\kern-1pt}={\kern-1pt}a^{2r}, c^a{\kern-1pt}={\kern-1pt}a^{2s}c^t,c^b{\kern-1pt}={\kern-1pt}a^uc^v\rangle ,$
whereif and only if $$ \begin{align*}&2(r^{t-1}-1)\equiv 2(r^{v-1}-1)\equiv u\bigg(\sum_{l=0}^{v-1}r^l-1\bigg)\equiv0\ \pmod {\ n},\\ &t^2\equiv v^2\equiv1\ \pmod {\ m},\\ &2s\sum_{l=1}^tr^{l}+2sr\equiv 2sr+2s\sum_{l=1}^{v}r^l-u\sum_{l=1}^tr^l+ur\equiv 2(1-r)\ \pmod {\ n},\\ &2s\sum_{l=1}^{w}r^l\equiv u\sum_{l=1}^{w}\bigg(1-s\bigg(\sum_{l=1}^tr^{l}+r\bigg)\bigg)^l\equiv0\ \pmod {\ n}\end{align*} $$
$$ \begin{align*}&2(r^{t-1}-1)\equiv 2(r^{v-1}-1)\equiv u\bigg(\sum_{l=0}^{v-1}r^l-1\bigg)\equiv0\ \pmod {\ n},\\ &t^2\equiv v^2\equiv1\ \pmod {\ m},\\ &2s\sum_{l=1}^tr^{l}+2sr\equiv 2sr+2s\sum_{l=1}^{v}r^l-u\sum_{l=1}^tr^l+ur\equiv 2(1-r)\ \pmod {\ n},\\ &2s\sum_{l=1}^{w}r^l\equiv u\sum_{l=1}^{w}\bigg(1-s\bigg(\sum_{l=1}^tr^{l}+r\bigg)\bigg)^l\equiv0\ \pmod {\ n}\end{align*} $$
 $w\equiv0\ \pmod {\ m}$
, and if
$w\equiv0\ \pmod {\ m}$
, and if
 $t\ne 1$
, then
$t\ne 1$
, then
 $u\equiv0\ \pmod {\ 2}$
.
$u\equiv0\ \pmod {\ 2}$
.
-
(2)
 $X=\langle a,b,c\mid R,(a^2)^{c^2}=a^{2r}, (c^2)^b{\kern-1pt}={\kern-1pt}a^{2s}c^{2}, (c^2)^a{\kern-1pt}={\kern-1pt}a^{2u}c^{2v}, a^c{\kern-1pt}={\kern-1pt}bc^{2w}\rangle ,$
where either
$X=\langle a,b,c\mid R,(a^2)^{c^2}=a^{2r}, (c^2)^b{\kern-1pt}={\kern-1pt}a^{2s}c^{2}, (c^2)^a{\kern-1pt}={\kern-1pt}a^{2u}c^{2v}, a^c{\kern-1pt}={\kern-1pt}bc^{2w}\rangle ,$
where either
 $w{\kern-1pt}={\kern-1pt}s{\kern-1pt}={\kern-1pt}u{\kern-1pt}={\kern-1pt}0$
and
$w{\kern-1pt}={\kern-1pt}s{\kern-1pt}={\kern-1pt}u{\kern-1pt}={\kern-1pt}0$
and
 $r{\kern-1pt}={\kern-1pt}t{\kern-1pt}={\kern-1pt}1$
; or
$r{\kern-1pt}={\kern-1pt}t{\kern-1pt}={\kern-1pt}1$
; or
 $w{\kern-1pt}\neq{\kern-1pt} 0,\,s{\kern-1pt}={\kern-1pt}u^2\sum _{l=0}^{w-1}r^l,\,t{\kern-1pt}={\kern-1pt}1{\kern-1pt}+{\kern-1pt}2wu$
,
$w{\kern-1pt}\neq{\kern-1pt} 0,\,s{\kern-1pt}={\kern-1pt}u^2\sum _{l=0}^{w-1}r^l,\,t{\kern-1pt}={\kern-1pt}1{\kern-1pt}+{\kern-1pt}2wu$
,
 $nw{\kern-1pt}\equiv{\kern-1pt} 2w(r{\kern-1pt}-{\kern-1pt}1){\kern-1pt}\equiv 2w(1+uw)\equiv 0\,({\mathrm {mod}}\ m/2)$
,
$nw{\kern-1pt}\equiv{\kern-1pt} 2w(r{\kern-1pt}-{\kern-1pt}1){\kern-1pt}\equiv 2w(1+uw)\equiv 0\,({\mathrm {mod}}\ m/2)$
,
 $r^{2w}-1\equiv (u\sum _{l=1}^{w}r^l)^2-r\equiv (r^w+1)(1+s\sum _{l=0}^{w-1}r^l) \equiv 0\,({\mathrm {mod}}\ n/2)$
and
$r^{2w}-1\equiv (u\sum _{l=1}^{w}r^l)^2-r\equiv (r^w+1)(1+s\sum _{l=0}^{w-1}r^l) \equiv 0\,({\mathrm {mod}}\ n/2)$
and
 $\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
$\sum _{l=1}^{i}r^l\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
 $i\equiv 0\,({\mathrm {mod}}\ m/2).$
$i\equiv 0\,({\mathrm {mod}}\ m/2).$
-
(3)
 $X=\langle a,b,c\mid R, a^{c^3}\hspace{-1pt}=\hspace{-1pt}a^r,(c^3)^b\hspace{-1pt}=\hspace{-1pt}a^{2u}c^{3}, a^c=bc^{{im}/2},b^c\hspace{-1pt}=\hspace{-1pt}a^xb\rangle ,$
where
$X=\langle a,b,c\mid R, a^{c^3}\hspace{-1pt}=\hspace{-1pt}a^r,(c^3)^b\hspace{-1pt}=\hspace{-1pt}a^{2u}c^{3}, a^c=bc^{{im}/2},b^c\hspace{-1pt}=\hspace{-1pt}a^xb\rangle ,$
where
 $n\hspace{-1pt}\equiv\hspace{-1pt} 2\,({\mathrm {mod}}\ 4)$
and either
$n\hspace{-1pt}\equiv\hspace{-1pt} 2\,({\mathrm {mod}}\ 4)$
and either
 $i=u=0$
and
$i=u=0$
and
 $r=x=1$
; or
$r=x=1$
; or
 $i=1$
,
$i=1$
,
 $6| m$
,
$6| m$
,
 $l^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
with
$l^{m/2}\equiv -1\,({\mathrm {mod}}\ n/2)$
with
 ${\mathrm {o}}(l)=m$
,
${\mathrm {o}}(l)=m$
,
 $r=l^3$
,
$r=l^3$
,
 $u= ({l^3-1})/{2l^2}$
and
$u= ({l^3-1})/{2l^2}$
and
 $x\equiv -l+l^2+ n/2\,({\mathrm {mod}}\ n)$
.
$x\equiv -l+l^2+ n/2\,({\mathrm {mod}}\ n)$
. -
(4)
 $X=\langle a,b,c\mid R, (a^2)^{c^3}=a^{2r}, (c^3)^b=a^{ ({2(l^3-1)})/{l^2}} c^3, (a^2)^c=bc^{ {im}/2}, b^c=a^{2(-l+l^2+ n/4)} b,c^a=a^{2+4z}c^{2+3d}\rangle ,$
where either
$X=\langle a,b,c\mid R, (a^2)^{c^3}=a^{2r}, (c^3)^b=a^{ ({2(l^3-1)})/{l^2}} c^3, (a^2)^c=bc^{ {im}/2}, b^c=a^{2(-l+l^2+ n/4)} b,c^a=a^{2+4z}c^{2+3d}\rangle ,$
where either
 $i=z=d=0$
and
$i=z=d=0$
and
 $l=1$
; or
$l=1$
; or
 $i=1$
,
$i=1$
,
 $n\equiv 4\,({\mathrm {mod}}\ 8),\, m\equiv 0\,({\mathrm {mod}}\ 6)$
,
$n\equiv 4\,({\mathrm {mod}}\ 8),\, m\equiv 0\,({\mathrm {mod}}\ 6)$
,
 $l^{m/2}\equiv -1\,({\mathrm {mod}}\ n/4)$
with
$l^{m/2}\equiv -1\,({\mathrm {mod}}\ n/4)$
with
 ${\mathrm {o}}(l)=m$
,
${\mathrm {o}}(l)=m$
,
 $r=l^3$
,
$r=l^3$
,
 $z= ({1-3l})/{4l}$
,
$z= ({1-3l})/{4l}$
,
 $1+3d\equiv 0\,({\mathrm {mod}}\ m/3)$
and
$1+3d\equiv 0\,({\mathrm {mod}}\ m/3)$
and
 $\sum _{i=1}^{j}r^i\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
$\sum _{i=1}^{j}r^i\equiv 0\,({\mathrm {mod}}\ n/2)$
if and only if
 $ j\equiv 0\,({\mathrm {mod}}\ m/3)$
.
$ j\equiv 0\,({\mathrm {mod}}\ m/3)$
. -
(5)
 $X=\langle a,b,c\mid R,a^{c^4}=a^r, b^{c^4}=a^{1-r}b, (a^3)^{c^{m/4}}=a^{-3}, a^{c^{m/4}}=bc^{{3m}/4}\rangle ,$
where
$X=\langle a,b,c\mid R,a^{c^4}=a^r, b^{c^4}=a^{1-r}b, (a^3)^{c^{m/4}}=a^{-3}, a^{c^{m/4}}=bc^{{3m}/4}\rangle ,$
where
 $m\equiv 4\,({\mathrm {mod}}\ 8)$
and r is of order
$m\equiv 4\,({\mathrm {mod}}\ 8)$
and r is of order
 $m/4$
in
$m/4$
in
 ${\mathbb {Z}}_{n}^*$
.
${\mathbb {Z}}_{n}^*$
.
Moreover, in the families of groups (1)–(5), for any given parameters satisfying the relevant equations, there exists
![]() $X=X(D)$
.
$X=X(D)$
.
Proof. Comparing Theorem 1.3 and Lemma 6.1, we get that
![]() $\langle a^n\rangle \lhd X(Q)$
for all cases in groups (2), (3) and some cases in group (1) under the hypothesis
$\langle a^n\rangle \lhd X(Q)$
for all cases in groups (2), (3) and some cases in group (1) under the hypothesis
![]() $\langle c\rangle _X=1$
. Moreover, corresponding to
$\langle c\rangle _X=1$
. Moreover, corresponding to
![]() $D\cong Q/\langle a^n\rangle $
, we have
$D\cong Q/\langle a^n\rangle $
, we have
![]() $X(D)=X(Q)/\langle a^n, c_1\rangle $
, where
$X(D)=X(Q)/\langle a^n, c_1\rangle $
, where
![]() $\langle a^n\rangle \lhd X(Q)$
and
$\langle a^n\rangle \lhd X(Q)$
and
![]() $\langle a^n, c_1\rangle =\langle a^n, c\rangle _{X(Q)}$
. Thus, Theorem 1.4 is proved.
$\langle a^n, c_1\rangle =\langle a^n, c\rangle _{X(Q)}$
. Thus, Theorem 1.4 is proved.
Acknowledgements
The authors would like to thank the reviewers for their valuable comments and suggestions.