Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-12-02T22:16:18.328Z Has data issue: false hasContentIssue false
  • English
  • Français

A note on cumulative sums of markovian variables

Published online by Cambridge University Press:  09 April 2009

R. M. Phatarfod
R. M. Phatarfod
Affiliation:
Monash University, Victoria
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Consider a positive regular Markov chain X0, X1, X2,… with s(s finite) number of states E1, E2,… E8, and a transition probability matrix P = (pij) where = 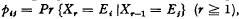, and an initial probability distribution given by the vector p0. Let {Zr} be a sequence of random variables such that and consider the sum SN = Z1+Z2+ … ZN. It can easily be shown that (cf. Bartlett [1] p. 37), 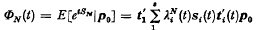where λ1(t), λ2(t)…λ1(t) are the latent roots of P(t) ≡ (pijethij) and si(t) and t′i(t) are the column and row vectors corresponding to λi(t), and so constructed as to give t′i(t)Si(t) = 1 and t′i(t), si(o) = si where t′i(t) and si are the corresponding column and row vectors, considering the matrix .

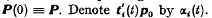Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 5 , Issue 2 , May 1965 , pp. 285 - 287
Copyright
Copyright © Australian Mathematical Society 1965

References

[1]Bartlett, M. S., An introduction to Stochastic Processes, Cambridge University Press (1955).Google Scholar
[2]Phatarfod, R. M., Sequential Analysis of dependent observations – I (to be published in Biometrika).Google Scholar
[3]Wald, A., Sequential Tests of Statistical Hypotheses, Annals, of Math. Statistics 16 (1945), 115.CrossRefGoogle Scholar