Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-25T07:26:35.043Z Has data issue: false hasContentIssue false
  • English
  • Français

LOCAL PROPERTIES OF THE HOCHSCHILD COHOMOLOGY OF C*-ALGEBRAS

Part of: Special classes of linear operators Selfadjoint operator algebras

Published online by Cambridge University Press:  01 February 2008

EBRAHIM SAMEI
EBRAHIM SAMEI*
Affiliation:
EPFL-SB-IACS, Station 8, CH-1015 Lausanne, Suisse
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let A be a C*-algebra, and let X be a Banach A-bimodule. Johnson [B. E. Johnson, ‘Local derivations on C*-algebras are derivations’, Trans. Amer. Math. Soc. 353 (2000), 313–325] showed that local derivations from A into X are derivations. We extend this concept of locality to the higher cohomology of a C*-algebra and show that, for every 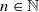, bounded local n-cocycles from A(n) into X are n-cocycles.

Keywords

local derivationslocal operatorslocal n-cocycleshyperlocal mapshyper-Tauberian algebrasC*-algebrasamenability and weak amenability

MSC classification

Secondary: 47B47: Commutators, derivations, elementary operators, etc. 46L57: Derivations, dissipations and positive semigroups in $C^*$-algebras
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 84 , Issue 1 , February 2008 , pp. 117 - 130
Copyright
Copyright © 2008 Australian Mathematical Society

References

[1]Dales, H. G., Banach algebras and automatic continuity (Oxford University Press, New York, 2000).Google Scholar
[2]Hadwin, D. and Li, J., ‘Local derivations and local automorphism’, J. Math. Anal. Appl. 290 (2004), 702–714.CrossRefGoogle Scholar
[3]Johnson, B. E., ‘Local derivations on C *-algebras are derivations’, Trans. Amer. Math. Soc. 353 (2000), 313–325.CrossRefGoogle Scholar
[4]Kadison, R. V., ‘Local derivations’, J. Algebra 130 (1990), 494–509.CrossRefGoogle Scholar
[5]Kadison, R. V. and Ringrose, J. R., ‘Cohomology of operator algebras. I, Type 1 Von Neumann algebras’, Acta Math. 126 (1971), 227–243.CrossRefGoogle Scholar
[6]Larson, D. R., ‘Reflexivity, algebraic reflexivity and linear interpolation’, Amer. J. Math. 110 (1988), 283–299.CrossRefGoogle Scholar
[7]Samei, E., ‘Bounded and completely bounded local derivations from certain commutative semisimple Banach algebras’, Proc. Amer. Math. Soc. 133 (2005), 229–238.CrossRefGoogle Scholar
[8]Samei, E., ‘Approximately local derivations’, J. London Math. Soc. (2) 71(3) (2005), 759–778.CrossRefGoogle Scholar
[9]Samei, E., ‘Hyper-Tauberian algebras and weak amenability of Figà-Talamanca-Herz algebras’, J. Funct. Anal. 231(1) (2006), 195–220.CrossRefGoogle Scholar
[10]Schweizer, J., ‘An analogue of Peetr’s theorem in non-commutative topology’, Quart. J. Math. 52 (2001), 499–506.CrossRefGoogle Scholar
[11]Shulman, V. S., ‘Spectral synthesis and the Fuglede–Putnam–Rosenblum theorem’, Teor. Funktsiĭ Funktsional. Anal. i Prilozhen. 54 (1990), 25–36 (in Russian) (Engl. Transl. J. Soviet Math. 58(4) (1992), 312–318).CrossRefGoogle Scholar