Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-25T07:41:22.141Z Has data issue: false hasContentIssue false
  • English
  • Français

LENS SPACE SURGERIES ALONG CERTAIN 2-COMPONENT LINKS RELATED WITH PARK’S RATIONAL BLOW DOWN, AND REIDEMEISTER-TURAEV TORSION

Part of: Algebraic number theory: global fields Low-dimensional topology

Published online by Cambridge University Press:  15 October 2013

TERUHISA KADOKAMI  and
YUICHI YAMADA
TERUHISA KADOKAMI*
Affiliation:
Department of Mathematics, East China Normal University, Dongchuan-lu 500, Shanghai, 200241, PR China
YUICHI YAMADA
Affiliation:
Department of Mathematics, The University of Electro-Communications, 1-5-1, Chofugaoka, Chofu, Tokyo email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We study lens space surgeries along two different families of 2-component links, denoted by 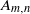${A}_{m, n} $ and 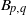${B}_{p, q} $, related with the rational homology 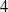$4$-ball used in J. Park’s (generalized) rational blow down. We determine which coefficient $r$ of the knotted component of the link yields a lens space by Dehn surgery. The link 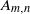${A}_{m, n} $ yields a lens space only by the known surgery with $r= mn$ and unexpectedly with 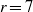$r= 7$ for 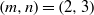$(m, n)= (2, 3)$. On the other hand, ${B}_{p, q} $ yields a lens space by infinitely many $r$. Our main tool for the proof are the Reidemeister-Turaev torsions, that is, Reidemeister torsions with combinatorial Euler structures. Our results can be extended to the links whose Alexander polynomials are same as those of 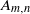${A}_{m, n} $ and 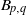${B}_{p, q} $.

Keywords

Dehn surgeryAlexander polynomialReidemeister torsioncombinatorial Euler structuregeneralized rational blow down

MSC classification

Secondary: 57M25: Knots and links in $S^3$ 57M27: Invariants of knots and 3-manifolds 57M50: Geometric structures on low-dimensional manifolds 11R04: Algebraic numbers; rings of algebraic integers 11R27: Units and factorization
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 96 , Issue 1 , February 2014 , pp. 78 - 126
Copyright
Copyright ©2013 Australian Mathematical Publishing Association Inc. 

References

Akbulut, S., ‘On two-dimensional homology classes of 4-manifolds’, Math. Proc. Cambridge Philos. Soc. 82 (1977), 99–106.Google Scholar
Baker, K., ‘Knots on once-punctured torus fibers’, Dissertation, The University of Texas at Austin, 2004.Google Scholar
Baker, K., Buck, D. and Lecuona, A., ‘Some knots in ${S}^{1} \times {S}^{2} $ with lens space surgeries’, arXiv:math.GT/1302.7011.Google Scholar
Berge, J., ‘Some knots with surgeries yielding lens spaces’ (unpublished manuscript, 1990).Google Scholar
Birman, J. S., Braids, Links, and Mapping Class Groups, Annals of Mathematics Studies, Vol. 82 (Princeton University Press, Princeton, NJ, 1974), University of Tokyo Press, Tokyo.Google Scholar
Boyer, S. and Lines, D., ‘Surgery formulae for Casson’s invariant and extensions to homology lens spaces’, J. reine angew. Math. 45 (1990), 181–220.Google Scholar
Burde, G. and Murasugi, K., ‘Links and Seifert fiber spaces’, Duke Math. J. 37 (1970), 89–93.CrossRefGoogle Scholar
Casson, A. and Harer, J., ‘Some homology lens spaces which bound rational homology balls’, Pacific J. Math. 96 (1) (1981), 23–36.Google Scholar
Culler, M., Gordon, M., Luecke, J. and Shalen, P., ‘Dehn surgery on knots’, Ann. of Math. (2) 125 (1987), 237–300.CrossRefGoogle Scholar
Deruelle, A., Miyazaki, K. and Motegi, K., ‘Networking Seifert surgeries on knots II: the Berge’s lens surgeries’, Topology Appl. 156 (6) (2009), 1083–1113.Google Scholar
Deruelle, A., Miyazaki, K. and Motegi, K., ‘Networking Seifert surgeries on knots’, Mem. Amer. Math. Soc. 217 (2012).Google Scholar
Endo, H., Mark, T. and Horn-Morris, J., ‘Monodromy substitutions and rational blowdowns’, J. Topol. 4 (1) (2011), 227–253.Google Scholar
Fintushel, R. and Stern, R., ‘Rational blow downs of smooth 4-manifolds’, J. Differential Geom. 46 (2) (1997), 181–235.CrossRefGoogle Scholar
Franz, W., ‘Über die torsion einer überdeckung’, J. reine angew. Math. 173 (1935), 245–254.CrossRefGoogle Scholar
Gompf, R. and Stipsicz, A., 4-manifolds and Kirby Calculus, Graduate Studies in Mathematics, 20 (American Mathematical Society Providence, RI, 1999).Google Scholar
Gordon, C. McA., ‘Dehn surgery and satellite knots’, Trans. Amer. Math. Soc. 275 (2) (1983), 687–708.CrossRefGoogle Scholar
Ichihara, K. and Saito, T., ‘Surgical distance between lens spaces’, Tokyo J. Math. 34 (1) (2011), 153–164.Google Scholar
Kadokami, T., ‘Reidemeister torsion and lens surgeries on knots in homology 3-spheres I’, Osaka J. Math. 43 (4) (2006), 823–837.Google Scholar
Kadokami, T., ‘Reidemeister torsion of Seifert fibered homology lens spaces and Dehn surgery’, Algebr. Geom. Topol. 7 (2007), 1509–1529.CrossRefGoogle Scholar
Kadokami, T., ‘Reidemeister torsion and lens surgeries on knots in homology 3-spheres II’, Topology Appl. 155 (15) (2008), 1699–1707.CrossRefGoogle Scholar
Kadokami, T., Hyperbolicity and identification of Berge knots of types VII and VIII, arXiv:math.GT/1107.0379.Google Scholar
Kadokami, T. and Shimozawa, M., ‘Dehn surgery along torus links’, J. Knot Theory Ramifications 19 (4) (2010), 489–502.Google Scholar
Kadokami, T., Maruyama, N. and Shimozawa, M., ‘Lens surgeries along the $n$-twisted Whitehead link’, Kyungpook Math. J. 52 (3) (2012), 245–264.CrossRefGoogle Scholar
Kadokami, T. and Yamada, Y., ‘Reidemeister torsion and lens surgeries on $(- 2, m, n)$-pretzel knots’, Kobe J. Math. 23 (1–2) (2006), 65–78.Google Scholar
Kadokami, T. and Yamada, Y., ‘A deformation of the Alexander polynomials of knots yielding lens spaces’, Bull. Aust. Math. Soc. 75 (1) (2007), 75–89.Google Scholar
Kadokami, T. and Yamada, Y., ‘Lens space surgeries along two component links and Reidemeister-Turaev torsion’, RIMS Kôkyûroku, 1747 Twisted Topological Invariants and Topology of Low-dimensional Manifolds, T. Morifuji (ed.), (2011), 67–76.Google Scholar
Kawauchi, A., A Survey of Knot Theory (Birkhäuser, Basel, 1996), translated and revised from the 1990 Japanese original by the author.Google Scholar
Kirby, R., ‘A calculus for framed links in ${S}^{3} $’, Invent. Math. 45 (1) (1978), 35–56.CrossRefGoogle Scholar
Lekili, Y. and Maydanskiy, M., ‘The symplectic topology of some rational homology balls’, Comment. Math. Helv., to appear, arXiv:math.GT/1202.5625.Google Scholar
Lisca, P., ‘On symplectic fillings of lens spaces’, Trans. Amer. Math. Soc. 360 (2) (2008), 765–799.CrossRefGoogle Scholar
Milnor, J. W., ‘A duality theorem for Reidemeister torsion’, Ann. of Math. (2) 76 (1962), 137–147.Google Scholar
Moser, L., ‘Elementary surgery along a torus knot’, Pacific J. Math. 38 (1971), 737–745.CrossRefGoogle Scholar
Park, J., ‘Seiberg–Witten invariants of generalised rational blow-downs’, Bull. Aust. Math. Soc. 56 (3) (1997), 363–384.Google Scholar
Rolfsen, D., ‘Rational surgery calculus: extension of Kirby’s theorem’, Pacific J. Math. 110 (1984), 377–386.Google Scholar
Sakai, T., ‘Reidemeister torsion of a homology lens space’, Kobe J. Math. 1 (1) (1984), 47–50.Google Scholar
Stipsicz, A. I., Szabó, Z. and Wahl, J., ‘Rational blow downs and smoothings of surface singularities’, J. Topol. 1 (2) (2008), 477–517.Google Scholar
Torres, G., ‘On the Alexander polynomial’, Ann. of Math. (2) 57 (1953), 57–89.CrossRefGoogle Scholar
Turaev, V. G., ‘Reidemeister torsion in knot theory’, Russian Math. Surveys 41-1 (1986), 119–182.Google Scholar
Turaev, V. G., Introduction to Combinatorial Torsions, Lectures in Mathematics (ETH Zürich, Birkhäuser, Basel, 2001).CrossRefGoogle Scholar
Washington, L. C., Introduction to Cyclotomic Fields, Graduate Texts in Mathematics, 83 (Springer, New York, 1982).Google Scholar
Yamada, Y., ‘Berge’s knots in the fiber surfaces of genus one, lens spaces and framed links’, J. Knot Theory Ramifications 14 (2) (2005), 177–188.Google Scholar
Yamada, Y., ‘Lens space surgeries as A’Campo’s divide knots’, Algebr. Geom. Topol. 9 (2009), 397–428.Google Scholar
Yamada, Y., Generalized rational blow-down, torus knots and Euclidean algorithm, arXiv:math.GT/0708.2316.Google Scholar
Yamada, Y., ‘Canonical forms of the knots in the genus one fiber surfaces’, Bull. Univ. Electro-Comm. 22 (1) (2010), 25–31.Google Scholar