Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Wong, W. J.
1982.
Abelian unipotent subgroups of finite orthogonal groups.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 32,
Issue. 2,
p.
223.
Wong, W. J.
1982.
Abelian Unipotent Subgroups of Finite Unitary and Symplectic Groups.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 33,
Issue. 3,
p.
331.
Kondrat'ev, A. S.
Makhnev, A. A.
and
Starostin, A. I.
1989.
Finite groups.
Journal of Soviet Mathematics,
Vol. 44,
Issue. 3,
p.
237.
Vdovin, E. P.
1999.
Maximal orders of Abelian subgroups in finite simple groups.
Algebra and Logic,
Vol. 38,
Issue. 2,
p.
67.
Вдовин, Евгений Петрович
and
Vdovin, Evgenii Petrovitch
2001.
Максимальные порядки абелевых подгрупп в конечных группах Шевалле.
Математические заметки,
Vol. 69,
Issue. 4,
p.
524.
Zeng, Bei
Chen, Xie
and
Chuang, Isaac L.
2008.
Semi-Clifford operations, structure ofCkhierarchy, and gate complexity for fault-tolerant quantum computation.
Physical Review A,
Vol. 77,
Issue. 4,
Levchuk, V. M.
and
Suleimanova, G. S.
2009.
Automorphisms and normal structure of unipotent subgroups of finitary Chevalley groups.
Proceedings of the Steklov Institute of Mathematics,
Vol. 267,
Issue. S1,
p.
118.
Suleimanova, G. S.
2010.
On conjugacy in a Chevalley group of large Abelian subgroups of the unipotent subgroup.
Journal of Mathematical Sciences,
Vol. 169,
Issue. 5,
p.
696.
Levchuk, Vladimir M.
and
Suleimanova, Galina S.
2012.
Extremal and maximal normal abelian subgroups of a maximal unipotent subgroup in groups of Lie type.
Journal of Algebra,
Vol. 349,
Issue. 1,
p.
98.
Levchuk, V. M.
and
Suleimanova, G. S.
2012.
The normal structure of the unipotent subgroup in Lie type groups and its extremal subgroups.
Journal of Mathematical Sciences,
Vol. 185,
Issue. 3,
p.
448.
Warner, Jared
2015.
Rational points and orbits on the variety of elementary subalgebras.
Journal of Pure and Applied Algebra,
Vol. 219,
Issue. 8,
p.
3355.
Potočnik, Primož
Spiga, Pablo
and
Verret, Gabriel
2015.
Bounding the order of the vertex-stabiliser in 3-valent vertex-transitive and 4-valent arc-transitive graphs.
Journal of Combinatorial Theory, Series B,
Vol. 111,
Issue. ,
p.
148.
Carlson, Jon F.
Friedlander, Eric M.
and
Pevtsova, Julia
2015.
Elementary subalgebras of Lie algebras.
Journal of Algebra,
Vol. 442,
Issue. ,
p.
155.
Popov, Vladimir L.
2015.
Finite subgroups of diffeomorphism groups.
Proceedings of the Steklov Institute of Mathematics,
Vol. 289,
Issue. 1,
p.
221.
Spiga, Pablo
and
Verret, Gabriel
2016.
On the order of vertex-stabilisers in vertex-transitive graphs with local group Cp×Cp or CpwrC2.
Journal of Algebra,
Vol. 448,
Issue. ,
p.
174.
Pevtsova, Julia
and
Stark, Jim
2018.
Geometric and Topological Aspects of the Representation Theory of Finite Groups.
Vol. 242,
Issue. ,
p.
339.
Spiga, Pablo
2018.
Cubic graphical regular representations of finite non-abelian simple groups.
Communications in Algebra,
Vol. 46,
Issue. 6,
p.
2440.
Kondrat’ev, A. S.
and
Trofimov, V. I.
2019.
Stabilizers of Vertices of Graphs with Primitive Automorphism Groups and a Strong Version of the Sims Conjecture. IV.
Proceedings of the Steklov Institute of Mathematics,
Vol. 307,
Issue. S1,
p.
64.
de Silva, Nadish
2021.
Efficient quantum gate teleportation in higher dimensions.
Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences,
Vol. 477,
Issue. 2251,
Yang, Yong
2022.
Abelian quotient of subgroups of solvable linear groups.
Communications in Algebra,
Vol. 50,
Issue. 12,
p.
5444.


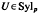 . In this paper Ab(
. In this paper Ab(