No CrossRef data available.
Article contents
Intersections of α-spaces
Published online by Cambridge University Press: 09 April 2009
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
Let 
be an infinite r.e. repère,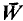 
an infinite dimensional r.e. space such that 
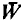≦ L(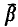
). A condition is derived that is both necessary and sufficient for the existence of an infinite subset β ⊂ 
such that L(β)∪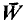
is not an α-space. Examples which satisfy this condition are exhibited, proving that the class of α-spaces is not closed under intersections.
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 20 , Issue 4 , November 1975 , pp. 398 - 418
- Copyright
- Copyright © Australian Mathematical Society 1975
References
Dekker, J. C. E. (1969), ‘Countable Vector Spaces with Recursive Operations, Part I’, The Journal of Symbolic Logic 34, 363–387.CrossRefGoogle Scholar
Dekker, J. C. E. (1971), ‘Countable Vector Spaces with Recursive Operations, Part II’, J. Symbolic Logic 36, 477–493.CrossRefGoogle Scholar
Fowler, N. (to appear), ‘agr;-Decompositions of agr;-Spaces’.Google Scholar
Guhl, R. (to appear), ‘A Theorem on Recursively Enumerable Vector Spaces’, Notre Dame Joural of Formal Logic.Google Scholar
Soare, R. I. (1974), ‘Isomorphism on Countable Vector Spaces with Recursive Operations’, J. Austral Math. Soc. 18, 230–235.CrossRefGoogle Scholar
You have
Access