Hostname: page-component-78c5997874-m6dg7 Total loading time: 0 Render date: 2024-11-08T18:29:08.430Z Has data issue: false hasContentIssue false
  • English
  • Français

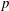GROUND STATE SOLUTIONS FOR $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}p$-SUPERLINEAR 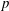$p$-LAPLACIAN EQUATIONS

Part of: Elliptic equations and systems

Published online by Cambridge University Press:  15 May 2014

YI CHEN  and
X. H. TANG
YI CHEN
Affiliation:
School of Mathematics and Statistics, Central South University, Changsha, Hunan 410083, PR China email [email protected]
X. H. TANG*
Affiliation:
School of Mathematics and Statistics, Central South University, Changsha, Hunan 410083, PR China email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

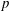In this paper, we deduce new conditions for the existence of ground state solutions for the $p$-Laplacian equation

$$\begin{equation*} \left \{ \begin{array}{@{}ll} -\mathrm {div}(|\nabla u|^{p-2}\nabla u)+V(x)|u|^{p-2}u=f(x, u), \quad x\in {\mathbb {R}}^{N},\\[5pt] u\in W^{1, p}({\mathbb {R}}^{N}), \end{array} \right . \end{equation*}$$
which weaken the Ambrosetti–Rabinowitz type condition and the monotonicity condition for the function $t\mapsto f(x, t)/|t|^{p-1}$. In particular, both 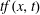$tf(x, t)$ and 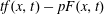$tf(x, t)-pF(x, t)$ are allowed to be sign-changing in our assumptions.

Keywords

p-Laplacian equationp-superlinearground state solutions

MSC classification

Secondary: 35J30: Higher-order elliptic equations
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 97 , Issue 1 , August 2014 , pp. 48 - 62
Copyright
Copyright © 2014 Australian Mathematical Publishing Association Inc. 

References

Alves, C. O. and Figueiredo, G. M., ‘Existence and multiplicity of positive solutions to ap-Laplacian equation in ℝN’, Differential Integral Equations 19 (2006), 143–162.CrossRefGoogle Scholar
Alves, C. O. and Figueiredo, G. M., ‘On multiplicity and concentration of positive solutions for a class of quasilinear problems with critical exponential growth in ℝN’, J. Differential Equations 246 (2009), 1288–1311.CrossRefGoogle Scholar
Ambrosetti, A. and Rabinowitz, P. H., ‘Dual variational methods in critical point theory and applications’, J. Funct. Anal. 14 (1973), 349–381.Google Scholar
Bartsch, T. and Liu, Z. L., ‘On a superlinear elliptic p-Laplacian equation’, J. Differential Equations 198 (2004), 149–175.CrossRefGoogle Scholar
Bartsch, T. and Wang, Z.-Q., ‘Existence and multiplicity results for some superlinear elliptic problems on ℝN’, Comm. Partial Differential Equations 20 (1995), 1725–1741.CrossRefGoogle Scholar
Coti Zelati, V. and Rabinowitz, P. H., ‘Homoclinic type solutions for a semilinear elliptic PDE on ℝN’, Comm. Pure Appl. Math. XIV (1992), 1217–1269.Google Scholar
Degiovanni, M. and Lancelotti, S., ‘Linking over cones and nontrivial solutions for p-Laplace equations with p-superlinear nonlinearity’, Ann. Inst. H. Poincaré Anal. Non Linéaire 24 (2007), 907–919.CrossRefGoogle Scholar
Ding, Y. and Lee, C., ‘Multiple solutions of Schrödinger equations with indefinite linear part and super or asymptotically linear terms’, J. Differential Equations 222 (2006), 137–163.CrossRefGoogle Scholar
Ding, Y. and Szulkin, A., ‘Bound states for semilinear Schrödinger equations with sign-changing potential’, Calc. Var. Partial Differential Equations 29 (2007), 397–419.Google Scholar
El Khalil, A., El Manouni, S. and Ouanan, M., ‘On some nonlinear elliptic problems for p-Laplacian in ℝN’, NoDEA Nonlinear Differential Equations Appl. 15 (2008), 295–307.CrossRefGoogle Scholar
Fang, F. and Liu, S. B., ‘Nontrivial solutions of superlinear p-Laplacian equations’, J. Math. Anal. Appl. 351 (2009), 138–146.CrossRefGoogle Scholar
Jeanjean, L., ‘On the existence of bounded Palais–Smale sequences and application to a Landesman–Lazer type problem set on ℝN’, Proc. Roy. Soc. Edinburgh 129 (1999), 787–809.Google Scholar
Jeanjean, L. and Tanaka, K., ‘A positive solution for asymptotically linear elliptic problem on ℝN autonomous at infinity’, ESAIM Control Optim. Calc. Var. 7 (2002), 597–614.Google Scholar
Jeanjean, L. and Tanaka, K., ‘Singularly perturbed elliptic problems with superlinear or asymptotically linear nonlinearities’, Calc. Var. Partial Differential Equations 21 (2004), 287–318.Google Scholar
Li, G. B. and Szulkin, A., ‘An asymptotically periodic Schrödinger equation with indefinite linear part’, Commun. Contemp. Math. 4 (2002), 763–776.CrossRefGoogle Scholar
Li, Y. Q., Wang, Z.-Q. and Zeng, J., ‘Ground states of nonlinear Schrödinger equations with potentials’, Ann. Inst. H. Poincaré Anal. Non Linéaire 23 (2006), 829–837.Google Scholar
Lions, P. L., ‘The concentration–compactness principle in the calculus of variations. The locally compact case, part 2’, Ann. Inst. H. Poincaré Anal. Non Linéaire 1 (1984), 223–283.CrossRefGoogle Scholar
Liu, S. B., ‘Existence of solutions to a superlinear p-Laplacian equation’, Electron. J. Differential Equations 66 (2001), 1–6.Google Scholar
Liu, S. B., ‘On ground states of superlinear p-Laplacian equations in ℝN’, J. Math. Anal. Appl. 361 (2010), 48–58.CrossRefGoogle Scholar
Liu, S. B. and Li, S. J., ‘Infinitely many solutions for a superlinear elliptic equation’, Acta Math. Sinica (Chin. Ser.) 46 (2003), 625–630 (in Chinese).Google Scholar
Liu, Z. L. and Wang, Z.-Q., ‘On the Ambrosetti–Rabinowitz superlinear condition’, Adv. Nonlinear Stud. 4 (2004), 561–572.CrossRefGoogle Scholar
Perera, K., ‘Nontrivial critical groups in p-Laplacian problems via the Yang index’, Topol. Methods Nonlinear Anal. 21 (2003), 301–309.Google Scholar
Rabinowitz, P. H., ‘On a class of nonlinear Schrödinger equations’, Z. Angew. Math. Phys. 43 (1992), 270–291.Google Scholar
Schechter, M., Minimax Systems and Critical Point Theory (Birkhäuser, Boston, MA, 2009).CrossRefGoogle Scholar
Szulkin, A. and Weth, T., ‘Ground state solutions for some indefinite variational problems’, J. Funct. Anal. 257 (2009), 3802–3822.CrossRefGoogle Scholar
Tang, X. H., ‘Infinitely many solutions for semilinear Schrödinger equations with sign-changing potential and nonlinearity’, J. Math. Anal. Appl. 401 (2013), 407–415.CrossRefGoogle Scholar
Willem, M., Minimax Theorems (Birkhäuser, Boston, MA, 1996).CrossRefGoogle Scholar
Yang, M., ‘Ground state solutions for a periodic Schrödinger equation with superlinear nonlinearities’, Nonlinear Anal. 72 (2010), 2620–2627.Google Scholar
Zou, W. M., ‘Variant fountain theorems and their applications’, Manuscripta Math. 104 (2001), 343–358.CrossRefGoogle Scholar