Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-25T07:45:22.287Z Has data issue: false hasContentIssue false
  • English
  • Français

GENERALIZED MORREY SPACES OVER NONHOMOGENEOUS METRIC MEASURE SPACES

Part of: Analysis on metric spaces Harmonic analysis in several variables

Published online by Cambridge University Press:  27 October 2016

GUANGHUI LU  and
SHUANGPING TAO
GUANGHUI LU
Affiliation:
College of Mathematics and Statistics, Northwest Normal University, 967 Anning East Road, Lanzhou 730070, PR China email [email protected]
SHUANGPING TAO*
Affiliation:
College of Mathematics and Statistics, Northwest Normal University, 967 Anning East Road, Lanzhou 730070, PR China email [email protected]
*
✉[email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

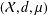Let $({\mathcal{X}},d,\unicode[STIX]{x1D707})$ be a nonhomogeneous metric measure space satisfying the so-called upper doubling and the geometric doubling conditions. In this paper, the authors give the natural definition of the generalized Morrey spaces on 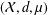$({\mathcal{X}},d,\unicode[STIX]{x1D707})$, and then investigate some properties of the maximal operator, the fractional integral operator and its commutator, and the Marcinkiewicz integral operator.

Keywords

nonhomogeneous metric measure spacegeneralized Morrey spacemaximal operatorfractional integral operatorMarcinkiewicz integral operator

MSC classification

Primary: 42B25: Maximal functions, Littlewood-Paley theory
Secondary: 42B35: Function spaces arising in harmonic analysis 30L99: None of the above, but in this section
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 103 , Issue 2 , October 2017 , pp. 268 - 278
Copyright
© 2016 Australian Mathematical Publishing Association Inc. 

Footnotes

This work was supported by National Natural Foundation of China (Grant No. 11561062).

References

Cao, Y. and Zhou, J., ‘Morrey spaces for nonhomogeneous metric measure spaces’, Abstr. Appl. Anal. 2013 (2013), 1–8.Google Scholar
Chen, W. and Sawyer, E., ‘A note on commutators of fractional integrals with RBMO(𝜇) functions’, Illinois J. Math. 46 (2002), 1287–1298.CrossRefGoogle Scholar
Coifman, R. R. and Weiss, G., ‘Analyse harmonique non-commutative sur certain espaces homogènes’, Lecture Notes in Mathematics, 242 (Springer, Berlin; New York, 1971).Google Scholar
Coifman, R. R. and Weiss, G., ‘Extensions of Hardy spaces and their use in analysis’, Bull. Amer. Math. Soc. 83 (1977), 569–645.Google Scholar
Eridani, Gunawan, H., Nakai, E. and Sawano, Y., ‘Characterizations for the generalized fractional integral operators on Morrey spaces’, Math. Inequal. Appl. 17 (2014), 761–777.Google Scholar
Fu, X., Yang, D. and Yuan, W., ‘Generalized fractional integrals and their commutators over non-homogeneous metric measure spaces’, Taiwanese J. Math. 18 (2014), 509–557.Google Scholar
Fu, X., Yang, D. and Yuan, W, ‘Boundedness on Orlicz spaces for multilinear commutators of Calderón-Zygmund operators on non-homogeneous spaces’, Taiwanese J. Math. 16 (2012), 2203–2238.Google Scholar
García-Cuerva, J. and Gatto, A. E., ‘‘Boundedness properties of fractional integral operators associated to non-doubling measures’, Studia Math. 162 (2004), 245–261.Google Scholar
Hakim, D. I., Nakai, E. and Sawano, Y., ‘Generalized fractional maximal operators and vector-valued inequalities on generalized Orlicz–Morrey spaces’, Rev. Mat. Complut. 29 (2016), 59–90.CrossRefGoogle Scholar
Hu, G., Lin, H. and Yang, D., ‘Marcinkiewicz integrals with non-doubling measures’, Integr. Equat. Oper. Th. 58 (2007), 205–238.CrossRefGoogle Scholar
Hytönen, T., ‘‘A framework for non-homogeneous analysis on metric spaces, and RBMO space of Tolsa’, Publ. Mat. 54 (2010), 485–504.CrossRefGoogle Scholar
Hytönen, T., Yang, Da. and Yang, Do., ‘The Hardy space H 1 on non-homogeneous metric measure spaces’, Math. Proc. Cambridge Philos. Soc. 153 (2012), 9–31.CrossRefGoogle Scholar
Lin, H. and Yang, D., ‘Equivalent boundedness of Marcinkiewicz on non-homogeneous metric measure spaces’, Sci. China Math. 57 (2014), 123–144.CrossRefGoogle Scholar
Lu, G. and Tao, S., ‘Estimates for parameter Littlewood–Paley g 𝜅 ∗ functions on non-homogeneous metric measure spaces’, J. Funct. Spaces Appl. 2016 (2016), 1–12.Google Scholar
Lu, G. and Zhou, J., ‘Estimates for fractional type Marcinkiewicz integrals with non-doubling measures’, J. Inequal. Appl. 2014 (2014), 1–14.Google Scholar
Sawano, Y., ‘Generalized Morrey spaces for non-doubling measures’, NoDEA Nonlinear Differential Equations Appl. 15 (2008), 413–425.Google Scholar
Sawano, Y. and Tanaka, H., ‘Morrey spaces for non-doubling measures’, Acta Math. Sinica 21 (2005), 1535–1544.CrossRefGoogle Scholar
Sawano, Y. and Tanaka, H., ‘Sharp maximal inequalities and commutators on Morrey spaces with non-doubling measures’, Taiwanese J. Math. 11 (2007), 1091–1112.Google Scholar
Sihwaningrum, I. and Sawano, Y., ‘Weak and strong type estimates for fractional integral operators on Morrey spaces over metric measure spaces’, Eurasian Math. J. 4 (2013), 76–81.Google Scholar
Tolsa, X., ‘The space H 1 for nondoubling measures in terms of a grand maximal operator’, Trans. Amer. Math. Soc. 355 (2003), 315–348.CrossRefGoogle Scholar
Tolsa, X., ‘Littlewood–Paley theory and the T (1) theorem with non-doubling measures’, Adv. Math. 164 (2001), 57–116.Google Scholar