Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Yu, Yaoming
and
Wang, Guorong
2009.
DFT calculation for the {2}-inverse of a polynomial matrix with prescribed image and kernel.
Applied Mathematics and Computation,
Vol. 215,
Issue. 7,
p.
2741.
Xu, Qingxiang
and
Zhang, Xiaobo
2010.
THE GENERALIZED INVERSES A(1,2)T,SOF THE ADJOINTABLE OPERATORS ON THE HILBERT C^*-MODULES.
Journal of the Korean Mathematical Society,
Vol. 47,
Issue. 2,
p.
363.
Song, Guang-Jing
and
Wang, Qing-Wen
2011.
Condensed Cramer rule for some restricted quaternion linear equations.
Applied Mathematics and Computation,
Vol. 218,
Issue. 7,
p.
3110.
Song, Guang-Jing
Wang, Qing-Wen
and
Chang, Hai-Xia
2011.
Cramer rule for the unique solution of restricted matrix equations over the quaternion skew field.
Computers & Mathematics with Applications,
Vol. 61,
Issue. 6,
p.
1576.
Khan, Israr Ali
and
Wang, Qing-Wen
2011.
The Drazin inverse in an arbitrary semiring.
Linear and Multilinear Algebra,
Vol. 59,
Issue. 9,
p.
1019.
Yu, Yaoming
2011.
The reverse order law for the generalized inverse over a skew field.
Applied Mathematics and Computation,
Vol. 217,
Issue. 24,
p.
10158.
Song, Guang-Jing
2012.
Determinantal representation of the generalized inverses over the quaternion skew field with applications.
Applied Mathematics and Computation,
Vol. 219,
Issue. 2,
p.
656.
Song, Guang-Jing
2012.
Determinantal representations of the generalized inverses $A_{T,S}^{(2)}$ over the quaternion skew field with applications.
Journal of Applied Mathematics and Computing,
Vol. 39,
Issue. 1-2,
p.
201.
Song, Guang-Jing
and
Yu, Shaowen
2014.
The block independence in the generalized inverse AT,S(2) for some ordered matrices and applications.
Applied Mathematics and Computation,
Vol. 232,
Issue. ,
p.
399.
Ke, Yuanyuan
Chen, Jianlong
Stanimirović, Predrag
and
Ćirić, Miroslav
2021.
Characterizations and representations of outer inverse for matrices over a ring.
Linear and Multilinear Algebra,
Vol. 69,
Issue. 1,
p.
155.
 and some explicit expressions for
and some explicit expressions for  of a matrix A over an associative ring, which reduce to the group inverse or {1} inverses. In addition, we show that for an arbitrary matrix A over an associative ring, the Drazin inverse Ad, the group inverse Ag and the Moore-Penrose inverse
of a matrix A over an associative ring, which reduce to the group inverse or {1} inverses. In addition, we show that for an arbitrary matrix A over an associative ring, the Drazin inverse Ad, the group inverse Ag and the Moore-Penrose inverse 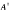 . if they exist, are all the generalized inverse A(2)T, S.
. if they exist, are all the generalized inverse A(2)T, S.