Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-25T09:15:05.977Z Has data issue: false hasContentIssue false
  • English
  • Français

A generalization of the Hall semigroup of a band

Part of: Semigroups

Published online by Cambridge University Press:  09 April 2009

Mária B. Szendrei
Mária B. Szendrei
Affiliation:
József Attila University Bolyai Institute, H-6720 Szeged Aradi vértanúk tere 1 Hungary
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let E be a band and ε a compatible partition on it. If S is an orthodox semigroup with band of idempotents E such that there exists a congruence on S inducing the partition ε then we define a homomorphism of S into a Hall semigroup whose kernel is the greatest congruence on S inducing the partition ε. On the other hand, we define a subsemigroup 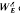of the Hall semigroup WE possessing the property that S is an othodox semigroup with band of idempotents E which has a congruence inducing ε if and only if the range of the Hall homomoprhism of S into WE is contained in .

MSC classification

Secondary: 20M10: General structure theory
Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 30 , Issue 1 , September 1980 , pp. 65 - 72
Copyright
Copyright © Australian Mathematical Society 1980

References

Clifford, A. H. and Preston, G. B. (1961, 1967), The algebraic theory of semigroups. Volumes I, II (Math. Surveys 7, Amer. Math. Soc., Providence, R.I.).Google Scholar
Feigenbaum, R. (1976), ‘Kernels of orthodox semigroup homomorphisms’, J. Austral. Math. Soc. 22, 234–245.CrossRefGoogle Scholar
Howie, J. M. (1976), An introduction to semigroup theory (Academic Press, London, New York, San Francisco).Google Scholar
Meakin, J. (1970), ‘Idempotent-equivalent congruences on orthodox semigroups’, J. Austal. Math. Soc. 11, 221–241.CrossRefGoogle Scholar
Reilly, N. R. and Scheiblich, H. E. (1967), ‘Congruences on regular semigroups’, Pacific J. Math. 23, 349–360.CrossRefGoogle Scholar