Convolution, fixed point, and approximation in Stieltjes-Volterra integral equations

Extract

This paper focuses primarily on two aspects of Stieltjes-Volterra integral equation theory. One is a theory for convolution integrals — that is, integrals of the form 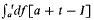— and the other is a fixed point theorem for a mapping which is induced by an integral equation. Throughout the paper I will denote the identity function whose range of definition should be clear from the context and all integrals will be left integrals, written 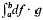, whose simplest approximating sum is [f(b) – f(a)]·g(a) and whose value is the limit of approximating sums with respect to successive refinements of the interval. Also, N will denote the set of elements of a complete normed ring with unity 1 and S will denote a set linearly ordered by ≦.