Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-25T08:41:58.418Z Has data issue: false hasContentIssue false
  • English
  • Français

A characterization of the orders of regressive ω-groups

Published online by Cambridge University Press:  09 April 2009

Matthew J. Hassett
Matthew J. Hassett
Affiliation:
Arizona State UniversityTempe, Arizona, U.S.A.
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let IE, ∧ and ∧R denote the collections of all non-negative integers, isols and regressive isols respectively. An ω-group is a pair (α, p) where (1) α ⊆ E, (2) p(x, y) is a partial recursive group multiplication for α and (3) the function which maps each element of α to its inverse under p has a partial recursive extension. If G = (α, p) is an ω-group, we call the recursive equivalence type of a the RET or order of G (written o(G)). Let GR = {T∈∧R′T = o(G) for some ω-group G}. It follows from the version of the Lagrange Theorem given in [4] that ∧R − GR is non-empty and has cardinality c. In this paper we characterise the isols in GR as follows: A regressive isol T belongs to GR if and only if T∈E or T is infinite and there exist a regressive isol U ≦ T and a function an from E into E − {0} such that U ≦*an and T = ΠUan. (The “≦*” is denned in [2]). In presenting the proof of this result, we shall assume that the reader is familiar with either [3] or [4]. The proof that, given an and U ≦*an, a group of order ΠUan exists is based on the natural trick—one constructs a direct product of disjoint cyclic groups of order a0, a1,… indexed by elements of a set of RET U. The proof that any regressive group G has order of the form ΠUan is trivial for finite groups; the proof for infinite regressive groups is based upon the construction of an ascending chain of finite subgroups Gi of G such that 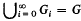and .

Type
Research Article
Information
Journal of the Australian Mathematical Society , Volume 14 , Issue 3 , November 1972 , pp. 379 - 382
Copyright
Copyright © Australian Mathematical Society 1972

References

[1]Dekker, J. C. E., Infinite series of Isols (Proc. Sympos. Pure Math., V (1962), Amer. Math. Soc., Providence, R. I.).Google Scholar
[2]Dekker, J. C. E., ‘The Minimum of Two Regressive Isols’, Math. Zeitschr. 83 (1964), 345–366.CrossRefGoogle Scholar
[3]Ellentuck, E., ‘Infinite Products of Isols’, Pacific J. Math. 14 (1964), 49–52.CrossRefGoogle Scholar
[4]Ferguson, D., ‘Infinite Products Recursive Equivalence Types’, J. Symbolic Logic 33 (1968), 221–230.CrossRefGoogle Scholar
[5]Hassett, M., ‘Recursive Equivalence Types and Groups’, J. Symbolic Logic 34 (1969), 13–20.CrossRefGoogle Scholar
[6]Myhill, J., ‘Recursive Equivalence Types and Combinatorial Function’, Bull. Am. Math. Soc. 64 (1958), 373–376.CrossRefGoogle Scholar
[7]Nerode, A., ‘Extensions to Isols’, Annals of Math. 73 (1961), 362–403.CrossRefGoogle Scholar