Article contents
SMALL-BOUND ISOMORPHISMS OF FUNCTION SPACES
Published online by Cambridge University Press: 18 March 2020
Abstract
Let 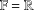 $\mathbb{F}=\mathbb{R}$ or
$\mathbb{F}=\mathbb{R}$ or 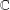 $\mathbb{C}$. For
$\mathbb{C}$. For  $i=1,2$, let
$i=1,2$, let 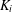 $K_{i}$ be a locally compact (Hausdorff) topological space and let
$K_{i}$ be a locally compact (Hausdorff) topological space and let  ${\mathcal{H}}_{i}$ be a closed subspace of
${\mathcal{H}}_{i}$ be a closed subspace of 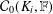 ${\mathcal{C}}_{0}(K_{i},\mathbb{F})$ such that each point of the Choquet boundary
${\mathcal{C}}_{0}(K_{i},\mathbb{F})$ such that each point of the Choquet boundary 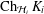 $\operatorname{Ch}_{{\mathcal{H}}_{i}}K_{i}$ of
$\operatorname{Ch}_{{\mathcal{H}}_{i}}K_{i}$ of 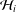 ${\mathcal{H}}_{i}$ is a weak peak point. We show that if there exists an isomorphism
${\mathcal{H}}_{i}$ is a weak peak point. We show that if there exists an isomorphism  $T:{\mathcal{H}}_{1}\rightarrow {\mathcal{H}}_{2}$ with
$T:{\mathcal{H}}_{1}\rightarrow {\mathcal{H}}_{2}$ with 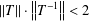 $\left\Vert T\right\Vert \cdot \left\Vert T^{-1}\right\Vert <2$, then
$\left\Vert T\right\Vert \cdot \left\Vert T^{-1}\right\Vert <2$, then 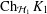 $\operatorname{Ch}_{{\mathcal{H}}_{1}}K_{1}$ is homeomorphic to
$\operatorname{Ch}_{{\mathcal{H}}_{1}}K_{1}$ is homeomorphic to 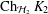 $\operatorname{Ch}_{{\mathcal{H}}_{2}}K_{2}$. We then provide a one-sided version of this result. Finally we prove that under the assumption on weak peak points the Choquet boundaries have the same cardinality provided
$\operatorname{Ch}_{{\mathcal{H}}_{2}}K_{2}$. We then provide a one-sided version of this result. Finally we prove that under the assumption on weak peak points the Choquet boundaries have the same cardinality provided  ${\mathcal{H}}_{1}$ is isomorphic to
${\mathcal{H}}_{1}$ is isomorphic to 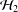 ${\mathcal{H}}_{2}$.
${\mathcal{H}}_{2}$.
MSC classification
- Type
- Research Article
- Information
- Journal of the Australian Mathematical Society , Volume 111 , Issue 3 , December 2021 , pp. 412 - 429
- Copyright
- © 2020 Australian Mathematical Publishing Association Inc.
Footnotes
Communicated by A. Sims
The research was supported by the research grant GAČR 17-00941S.
References
- 1
- Cited by



