No CrossRef data available.
Article contents
WEAKLY 2-RANDOMS AND 1-GENERICS IN SCOTT SETS
Published online by Cambridge University Press: 01 May 2018
Abstract
Let  ${\cal S}$ be a Scott set, or even an ω-model of WWKL. Then for each A ε S, either there is X ε S that is weakly 2-random relative to A, or there is X ε S that is 1-generic relative to A. It follows that if A1,…,An ε S are noncomputable, there is X ε S such that each Ai is Turing incomparable with X, answering a question of Kučera and Slaman. More generally, any ∀∃ sentence in the language of partial orders that holds in
${\cal S}$ be a Scott set, or even an ω-model of WWKL. Then for each A ε S, either there is X ε S that is weakly 2-random relative to A, or there is X ε S that is 1-generic relative to A. It follows that if A1,…,An ε S are noncomputable, there is X ε S such that each Ai is Turing incomparable with X, answering a question of Kučera and Slaman. More generally, any ∀∃ sentence in the language of partial orders that holds in 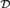 ${\cal D}$ also holds in
${\cal D}$ also holds in 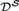 ${{\cal D}^{\cal S}}$, where
${{\cal D}^{\cal S}}$, where 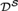 ${{\cal D}^{\cal S}}$ is the partial order of Turing degrees of elements of
${{\cal D}^{\cal S}}$ is the partial order of Turing degrees of elements of  ${\cal S}$.
${\cal S}$.
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2018


