Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-28T13:22:14.138Z Has data issue: false hasContentIssue false
  • English
  • Français

Wadge hierarchy and Veblen hierarchy Part I: Borel sets of finite rank

Published online by Cambridge University Press:  12 March 2014

J. Duparc
J. Duparc*
Affiliation:
Equipe de Logique Mathématique, CNRS URA 753, et Université Paris VII, U.F.R. de Mathématiques, 2 Place Jussieu. 75251 Paris Cedex 05, France, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

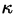We consider Borel sets of finite rank A ⊆ ∧ω where cardinality of Λ is less than some uncountable regular cardinal . We obtain a “normal form” of A, by finding a Borel set Ω, such that A and Ω continuously reduce to each other. In more technical terms: we define simple Borel operations which are homomorphic to ordinal sum, to multiplication by a countable ordinal, and to ordinal exponentiation of base 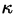, under the map which sends every Borel set A of finite rank to its Wadge degree.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 66 , Issue 1 , March 2001 , pp. 56 - 86
Copyright
Copyright © Association for Symbolic Logic 2001

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Kechris, A.S., Classical descriptive set theory, Graduate texts in mathematics, vol. 156, Springer Verlag, 1994.Google Scholar
[2]Louveau, A., Some results in the Wadge hierarchy of Borel sets, Cabal seminar 79-81, Lecture Notes in Mathematics, vol. 1019, Springer Verlag, 1983, pp. 28–55.CrossRefGoogle Scholar
[3]Louveau, A. and Raymond, J. St., The strength of Borel Wadge determinacy, Cabal seminar 81–85, Lecture Notes in Mathematics, vol. 1333, Springer Verlag, 1985, pp. 1–30.Google Scholar
[4]Louveau, A. and Raymond, J. St., Les propriétés de réduction et de norme pour les classes de Boréliens, Fundamenta Mathematical vol. 131 (1988), pp. 223–243.CrossRefGoogle Scholar
[5]Martin, D. A., Borel determinacy, Annals of Mathematics, vol. 102 (1975), pp. 363–371.CrossRefGoogle Scholar
[6]van Wesep, R., Wadge degrees and descriptive set theory, Cabal seminar 76–77, (Proceedings of the Caltech-UCLA logic seminar, 1976–77), Lecture Notes in Mathematics, vol. 689, Springer, Berlin, 1978, pp. 151–170.Google Scholar
[7]Veblen, O., Continuous increasing fonctions of finite and transfinite ordinals, Transactions of the American Mathematical Society, vol. 9 (1908), pp. 280–292.CrossRefGoogle Scholar
[8]Wadge, W. W., Degrees of complexity of subsets of the Baire space, Notices of the American Mathematical Society, (1972), pp. A–714.Google Scholar
[9]Wadge, W. W., Reducibility and determinateness on the Baire space., Ph.D. thesis, Berkeley, 1984.Google Scholar