Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-27T12:04:43.106Z Has data issue: false hasContentIssue false
  • English
  • Français

TOPOLOGICAL CELL DECOMPOSITION AND DIMENSION THEORY IN P-MINIMAL FIELDS

Published online by Cambridge University Press:  21 March 2017

PABLO CUBIDES KOVACSICS ,
LUCK DARNIÈRE  and
EVA LEENKNEGT
PABLO CUBIDES KOVACSICS
Affiliation:
LABORATOIRE NICOLAS ORESME UNIVERSITÉ DE CAEN CNRS U.M.R. 6139 F 14032 CAEN CEDEX, FRANCE E-mail: [email protected]
LUCK DARNIÈRE
Affiliation:
LAREMA, UNIVERSITÉ D’ANGERS 2 BD LAVOISIER, 49045 ANGERS CEDEX 01, FRANCE E-mail: [email protected]
EVA LEENKNEGT
Affiliation:
DEPARTMENT OF MATHEMATICS KULEUVEN CELESTIJNENLAAN 200B, 3001 HEVERLEE, BELGIUM E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

This paper addresses some questions about dimension theory for P-minimal structures. We show that, for any definable set A, the dimension of 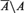$\bar A\backslash A$ is strictly smaller than the dimension of A itself, and that A has a decomposition into definable, pure-dimensional components. This is then used to show that the intersection of finitely many definable dense subsets of A is still dense in A. As an application, we obtain that any definable function 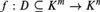$f:D \subseteq {K^m} \to {K^n}$ is continuous on a dense, relatively open subset of its domain D, thereby answering a question that was originally posed by Haskell and Macpherson.

In order to obtain these results, we show that P-minimal structures admit a type of cell decomposition, using a topological notion of cells inspired by real algebraic geometry.

Keywords

P-minimalityp-adically closed fieldsdimensioncell decomposition
Type
Articles
Information
The Journal of Symbolic Logic , Volume 82 , Issue 1 , March 2017 , pp. 347 - 358
Copyright
Copyright © The Association for Symbolic Logic 2017 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bochnak, J., Coste, M., and Roy, M.-F., Géométrie algébrique réelle, Ergebnisse der Mathematik und ihrer Grenzgebiete (3), vol. 12, Springer-Verlag, Berlin, 1987.Google Scholar
Cluckers, R., Analytic p-adic cell decomposition and integrals . Transactions of the American Mathematical Society, vol. 356 (2004), no. 4, pp. 1489–1499.Google Scholar
Cluckers, R. and Loeser, F., b-minimality . Journal of Mathematical Logic, vol. 7 (2007), no. 2, pp. 195–227.CrossRefGoogle Scholar
Cohen, P. J., Decision procedures for real and p-adic fields . Communications on Pure and Applied Mathematics, vol. 22 (1969), pp. 131–151.Google Scholar
Cubides Kovacsics, P. and Leenknegt, E., Integration and cell decomposition in P-minimal structures, this Journal, vol. 81 (2016), pp. 1124–1141.Google Scholar
Cubides Kovacsics, P. and Nguyen, K. H., A P-minimal field without definable skolem functions, this Journal, to appear, arXiv:1605.00945.Google Scholar
Darnière, L. and Halupczok, I., Cell decomposition and dimension theory in p-optimal fields, this Journal, vol. 82 (2017), no. 1, pp. 120–136.Google Scholar
Denef, J., The rationality of the Poincaré series associated to the p-adic points on a variety . Inventiones Mathematicae, vol. 77 (1984), no. 1, pp. 1–23.Google Scholar
Denef, J., p-adic semi-algebraic sets and cell decomposition . Journal für die Reine und Angewandte Mathematik, vol. 369 (1986), pp. 154–166.Google Scholar
van den Dries, L., Dimension of definable sets, algebraic boundedness and Henselian fields . Annals of Pure and Applied Logic, vol. 45 (1989), no. 2, pp. 189–209, Stability in model theory, II (Trento, 1987).Google Scholar
van den Dries, L., Tame Topology and O-minimal Structures, London Mathematical Society Lecture Note Series, vol. 248, Cambridge University Press, Cambridge, 1998.Google Scholar
Haskell, D. and Macpherson, D., A version of o-minimality for the p-adics, this Journal, vol. 62 (1997), no. 4, pp. 1075–1092.Google Scholar
Kuijpers, T. and Leenknegt, E., Differentiation in P-minimal structures and a p-adic local monotonicity theorem, this Journal, vol. 79 (2014), no. 4, pp. 1133–1147.Google Scholar
Mathews, L., Cell decomposition and dimension functions in first-order topological structures . Proceedings of the London Mathematical Society, Third Series, vol. 70 (1995), no. 1, pp. 1–32.Google Scholar
Mourgues, M-H., Cell decomposition for P-minimal fields . Mathematical Logic Quarterly, vol. 55 (2009), no. 5, pp. 487–492.Google Scholar
Pas, J., Cell decomposition and local zeta functions in a tower of unramified extensions of a p-adic field . Proceedings of the London Mathematical Society, Third Series, vol. 60 (1990), no. 1, pp. 37–67.Google Scholar