Article contents
A theorem on the isomorphism property
Published online by Cambridge University Press: 12 March 2014
Abstract
An 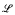 -structure is called internally presented in a nonstandard universe if its base set and interpretation of every symbol in
-structure is called internally presented in a nonstandard universe if its base set and interpretation of every symbol in 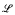 are internal. A nonstandard universe is said to satisfy the κ-isomorphism property if for any two internally presented
are internal. A nonstandard universe is said to satisfy the κ-isomorphism property if for any two internally presented 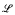 -structures
-structures 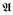 and
and 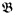 , where
, where 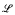 has less than κ many symbols,
has less than κ many symbols, 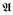 is elementarily equivalent to
is elementarily equivalent to 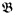 implies that
implies that 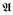 is isomorphic to
is isomorphic to 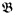 . In this paper we prove that the ℵ1-isomorphism property is equivalent to the ℵ0-isomorphism property plus ℵ1-saturation.
. In this paper we prove that the ℵ1-isomorphism property is equivalent to the ℵ0-isomorphism property plus ℵ1-saturation.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1992
References
REFERENCES
- 5
- Cited by


