SYMÉTRIES ET TRANSVEXIONS, PRINCIPALEMENT DANS LES GROUPES DE RANG DE MORLEY FINI SANS INVOLUTIONS

Résumé

L'analyse de la démonstration par contradiction de Frécon 2018 qui est faite dans Poizat 2018 met en évidence la structure symétrique des groupes de rang de Morley fini sans involutions; en effet, cette démonstration consiste en la construction d'un espace symétrique de dimension deux (“un plan”), puis à montrer que ce plan ne peut exister.

Aux sous-espaces symétriques définissables de ces groupes sont associées des symétries et des transvexions, qu'on entreprend d'étudier ici dans l'abstrait, sans référence à un groupe qui les enveloppe; cela nous mène à considérer des structures introduites axiomatiquement que nous appelons symétrons (plutôt qu'ensembles symétriques diadiques, comme les ont nommées Lawson & Lim 2004).

Le 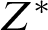$Z^*$ -Theorem de Glauberman permet d'élucider complètement la structure des symétrons finis: chacun est isomorphe à l'ensemble des symétries associées à un sous-espace symétrique d'un groupe fini sans involutions, qui est loin d'être uniquement déterminé: de fait, il existe des groupes finis non isomorphes qui ont les mêmes symétries, et aussi des symétrons finis qui ne sont pas isomorphes aux symétries d'un groupe,

La situation est plus incertaine dans le cas des symétrons de rang de Morley fini, ou même algébriques, qui sont l'objet d'étude principal de cet article. Mais bien qu'un symétron soit une structure nettement plus faible qu'un groupe, nous pouvons étendre aux symétrons des résultats bien connus à propos des groupes de rang de Morley fini: condition de chaîne, décomposition en composantes connexes, caractérisation des parties définissables génériques, génération elliptique, etc. Ces propriétés sont nouvelles même dans le cas des sous-espaces symétriques d’un groupe, et permettent de court-circuiter les calculs de Frécon dans la construction de son plan paradoxal.

En outre, sous l'hypothèse de la Conjecture d'Algébricité, nous généralisons le Théorème de Glauberman au contexte de rang de Morley fini.

Abstract

The role played by the symmetric structure of a group of finite Morley rank without involutions in the proof by contradiction of Frécon 2018 was put in evidence in Poizat 2018; indeed, this proof consists in the construction of a symmetric space of dimension two (“a plane”), and then in showing that such a plane cannot exist.

To a definable symmetric subset of such a group are associated symmetries and transvections, that we undertake here to study in the abstract, without mentioning a group envelopping them. This leads us to consider axiomatically defined structures that we call symmetrons (preferably to dyadic symmetric sets as was done in Lawson & Lim 2004).

Glauberman's 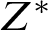$Z^*$ -Theorem allows to elucidate completely the structure of the finite symmetrons: each of them is isomorphic to the set of symmetries associated to a symmetric subspace of a finite group without involutions, which is far from being uniquely determined. In fact, there exist non-isomorphic finite groups which have the same symmetries, and also finite symmetrons which are not isomorphic to the symmetries of a group.

The situation is not so clear in the case of symmetrons of finite Morley rank, or even algebraic, which are the main objects of study of this paper. But in spite of the fact that a symmetron be a structure much weaker that a group, we can extend to symmetrons some well-known results concerning groups of finite Morley rank: chain condition, decomposition into connected components, characterisation of the generic definable subsets, elliptic generation, etc. These properties are new even in the case of a symmetric subspace of a group, and allow to bypass the computations made by Frécon during the construction of his paradoxical plane.

Moreover, assuming the Algebricity Conjecture, we generalize Glauberman's Theorem to the finite Morley rank context.