Published online by Cambridge University Press: 08 January 2021
For every countable wellordering 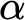 $\alpha $
greater than
$\alpha $
greater than 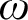 $\omega $
, it is shown that clopen determinacy for games of length
$\omega $
, it is shown that clopen determinacy for games of length  $\alpha $
with moves in
$\alpha $
with moves in 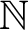 $\mathbb {N}$
is equivalent to determinacy for a class of shorter games, but with more complicated payoff. In particular, it is shown that clopen determinacy for games of length
$\mathbb {N}$
is equivalent to determinacy for a class of shorter games, but with more complicated payoff. In particular, it is shown that clopen determinacy for games of length  $\omega ^2$
is equivalent to
$\omega ^2$
is equivalent to 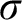 $\sigma $
-projective determinacy for games of length
$\sigma $
-projective determinacy for games of length 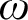 $\omega $
and that clopen determinacy for games of length
$\omega $
and that clopen determinacy for games of length 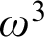 $\omega ^3$
is equivalent to determinacy for games of length
$\omega ^3$
is equivalent to determinacy for games of length 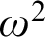 $\omega ^2$
in the smallest
$\omega ^2$
in the smallest 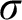 $\sigma $
-algebra on
$\sigma $
-algebra on 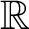 $\mathbb {R}$
containing all open sets and closed under the real game quantifier.
$\mathbb {R}$
containing all open sets and closed under the real game quantifier.