Article contents
Relative lawlessness in intuitionistic analysis
Published online by Cambridge University Press: 12 March 2014
Abstract
This paper introduces, as an alternative to the (absolutely) lawless sequences of Kreisel and Troelstra, a notion of choice sequence lawless with respect to a given class 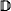 of lawlike sequences. For countable
of lawlike sequences. For countable 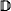 , the class of
, the class of 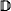 -lawless sequences is comeager in the sense of Baire. If a particular well-ordered class
-lawless sequences is comeager in the sense of Baire. If a particular well-ordered class  of sequences, generated by iterating definability over the continuum, is countable then the
of sequences, generated by iterating definability over the continuum, is countable then the  -lawless sequences satisfy the axiom of open data and the continuity principle for functions from lawless to lawlike sequences, but fail to satisfy Troelstra's extension principle. Classical reasoning is used.
-lawless sequences satisfy the axiom of open data and the continuity principle for functions from lawless to lawlike sequences, but fail to satisfy Troelstra's extension principle. Classical reasoning is used.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1987
Footnotes
I wish to thank Occidental College for granting me a sabbatical leave, and UCLA for allowing me to use its research facilities, in 1983-84 when most of this work was done. I also appreciate the referee's helpful suggestions on exposition.
References
REFERENCES
- 3
- Cited by


