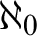Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Ben Yaacov, Itaï
2023.
Star sorts, Lelek fans, and the reconstruction of
non-ℵ0-categorical theories in continuous logic.
Model Theory,
Vol. 2,
Issue. 2,
p.
285.
Chen, Ruiyuan
2024.
Structural, point-free, non-Hausdorff topological realization of Borel groupoid actions.
Forum of Mathematics, Sigma,
Vol. 12,
Issue. ,