Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-27T05:17:32.523Z Has data issue: false hasContentIssue false
  • English
  • Français

Properties of ideals on the generalized Cantor spaces

Published online by Cambridge University Press:  12 March 2014

Jan Kraszewski
Jan Kraszewski*
Affiliation:
Institute of Mathematics, University of Wrocław, PL. Grunwaldzki 2/4. 50-156 Wrocław, Poland, E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We define a class of productiveσ-ideals of subsets of the Cantor space 2ω and observe that both σ-ideals of meagre sets and of null sets are in this class. From every productive σ-ideal 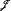we produce a σ-ideal 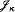of subsets of the generalized Cantor space 2κ. In particular, starting from meagre sets and null sets in 2ω we obtain meagre sets and null sets in 2ω, respectively. Then we investigate additivity, covering number, uniformity and cofinality of 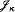. For example, we show that

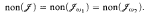
Our results generalizes those from [5].

Keywords

Cantor spaceσ-idealsnull setsmeagre setscardinal functions
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 66 , Issue 3 , September 2001 , pp. 1303 - 1320
Copyright
Copyright © Association for Symbolic Logic 2001

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Cichoń, J., handwritten notes, 1986.Google Scholar
[2]Cichoń, J., On two-cardinal properties of ideals, Transactions of the American Mathematical Society, vol. 314 (1989), pp. 693–708.CrossRefGoogle Scholar
[3]Cichoń, J. and Kraszewski, J., On some new ideals on the Cantor and Baire spaces, Proceedings of the American Mathematical Society, vol. 126 (1998), pp. 1549–1555.CrossRefGoogle Scholar
[4]Engelking, R., General topology, Państwowe Wydawnictwo Naukowe, Warsaw, 1985, 2nd edition.Google Scholar
[5]Fremlin, D. H., Measure algebras, Handbook of boolean algebras (Monk, J. D. and Bonnet, R., editors), North-Holland, Amsterdam, 1989, pp. 879–980.Google Scholar
[6]Fremlin, D. H., Real-valued measurable cardinals, Israel mathematical conference proceedings, vol. 6, 1993, pp. 151–304.Google Scholar
[7]Fuchino, S., Shelah, S., and Soukup, L., Sticks and clubs, Annals of Pure and Applied Logic, vol. 90 (1997), pp. 57–77.CrossRefGoogle Scholar
[8]Jech, T., Multiple forcing, Cambridge University Press, Cambridge, 1986.Google Scholar
[9]Jech, T. and Prikry, K., Ideals over uncountable sets: Application of almost disjoint functions and generic ultrapower, Memoirs of the American Mathematical Society, vol. 18 (1979).CrossRefGoogle Scholar
[10]Jech, T. and Prikry, K., Cofinality of the partial ordering offunctions from ω1 into co under eventual domination, Mathematical Proceedings of the Cambridge Philosophical Society, vol. 95 (1984), pp. 25–32.CrossRefGoogle Scholar
[11]Kamburelis, A. and Weglorz, B., Splittings, Archive for Mathematical Logic, vol. 35 (1996), pp. 263–277.CrossRefGoogle Scholar
[12]Kunen, K., Set theory, North-Holland, Amsterdam, 1980.Google Scholar
[13]Kunen, K., Random and Cohen reals, (Kunen, K. and Vaughan, J. A., editors), North-Holland, Amsterdam, 1984, pp. 889–911.Google Scholar
[14]Miller, A. W., Some properties of measure and category, Transactions of the American Mathematical Society, vol. 266 (1981), pp. 93–114.CrossRefGoogle Scholar
[15]Miller, A. W., The Baire category theorem and cardinals of countable cofinality, this Journal, vol. 47 (1982), pp. 275–288.Google Scholar
[16]Vaughan, J. E., Small uncountable cardinals and topology, Open problems in topology, North-Holland, 1990, pp. 195–218.Google Scholar