One-dimensional fibers of rigid subanalytic sets

Extract

Let K be an algebraically closed field of any characteristic, complete with respect to the non-trivial ultrametric absolute value ∣·∣: K → ℝ₊. By R denote the valuation ring of K, and by ℘ its maximal ideal. We work within the class of subanalytic sets defined in [5], but our results here also hold for the strongly subanalytic sets introduced in [11] as well as for those subanalytic sets considered in [6]. Let X ⊂ R¹ be subanalytic. In [8], we showed that there is a decomposition of X as a union of a finite number of special sets U ⊂ R¹ (see below). In this note, in Theorem 1.6, we obtain a version of this result which is uniform in parameters, thereby answering a question brought to our attention by Angus Macintyre. It follows immediately from Theorem 1.6 that the theory of K in the language 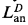(see [5] and [6]) is C-minimal in the sense of [3] and [9]. The analogous uniformity result in the p-adic case was recently proved in [12].

Definition 1.1. (i) A disc in R¹ is a set of one of the two following forms:

A special set in R¹ is a disc minus a finite union of discs.

(ii) R-domains u ⊂ R^m, and their associated rings of analytic functions, 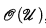, are defined inductively as follows. R^m is an R-domain and , the ring of strictly convergent power series in X₁,…, X_m over K. If u is an R-domain with associated ring 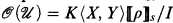, (where K 〈X, Y〉 〚ρ〛_S is a ring of separated power series, see [5, §2] and [1, §1]) and f, 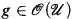have no common zero on u and ◸ ϵ {<, ≤}, then

is an R-domain and

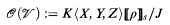
where J is the ideal generated by I and f − gZ (Z is a new variable) if ◸ is ≤, and

where J is the ideal generated by I and f − gτ (τ a new variable) if ◸ is <. (See [8, Definition 2.2].) R-domains generalize the rational domains of [2, §7.2.3]. It is true, but not easy to prove, that 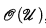only depends on u as a point set, and is independent of the particular representation of u.