Article contents
On the structure of semialgebraic sets over p-adic fields
Published online by Cambridge University Press: 12 March 2014
Extract
In his Singular points of complex hypersurfaces Milnor proves the following “curve selection lemma” [10, p. 25]:
Let V ⊂ R m be a real algebraic set, and let U ⊂ R m be an open set defined by finitely many polynomial inequalities:
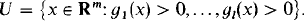
Lemma 3.1. If U ∩ V contains points arbitrarily close to the origin (that is if 0 ∈ Closure (U ∩ V)) then there exists a real analytic curve
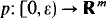
with p(0) = 0 and with p(t) ∈ U ∩ V for t > 0.
Of course, this result will also apply to semialgebraic sets (finite unions of sets U ∩ V), and by Tarski's theorem such sets are exactly the sets obtained from real varieties by means of the finite Boolean operations and the projection maps R
n+1 → R
n
. If, in this tiny extension of Milnor's result, we replace ‘R’ everywhere by ‘Q
p
’, we obtain a p-adic curve selection lemma, a version of which we will prove in this essay. Semialgebraic sets, in the p-adic context, may be defined just as they are over the reals: namely, as those sets obtained from p-adic varieties by means of the finite Boolean operations and the projection maps 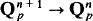 . Analytic maps are maps whose coordinate functions are given locally by convergent power series.
. Analytic maps are maps whose coordinate functions are given locally by convergent power series.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1988
References
REFERENCES
- 14
- Cited by


