ON 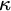$\kappa $-HOMOGENEOUS, BUT NOT 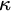$\kappa $-TRANSITIVE PERMUTATION GROUPS

Abstract

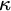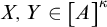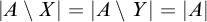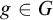A permutation group G on a set A is ${\kappa }$ -homogeneous iff for all $X,Y\in \bigl [ {A} \bigr ]^ {\kappa } $ with $|A\setminus X|=|A\setminus Y|=|A|$ there is a $g\in G$ with $g[X]=Y$ . 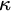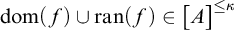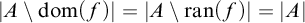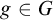G is ${\kappa }$ -transitive iff for any injective function f with $\operatorname {dom}(f)\cup \operatorname {ran}(f)\in \bigl [ {A} \bigr ]^ {\le {\kappa }} $ and $|A\setminus \operatorname {dom}(f)|=|A\setminus \operatorname {ran}(f)|=|A|$ there is a $g\in G$ with $f\subset g$ .

Giving a partial answer to a question of P. M. Neumann [6] we show that there is an 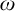${\omega }$ -homogeneous but not 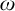${\omega }$ -transitive permutation group on a cardinal 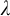${\lambda }$ provided

1. (i) 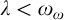${\lambda }<{\omega }_{\omega }$ , or

2. (ii) $2^{\omega }<{\lambda }$ , and ${\mu }^{\omega }={\mu }^+$ and 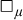$\Box _{\mu }$ hold for each ${\mu }\le {\lambda }$ with 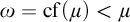${\omega }=\operatorname {cf}({\mu })<{{\mu }}$ , or

3. (iii) our model was obtained by adding 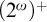$(2^{\omega })^+$ many Cohen generic reals to some ground model.

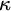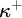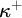For ${\kappa }>{\omega }$ we give a method to construct large ${\kappa }$ -homogeneous, but not ${\kappa }$ -transitive permutation groups. Using this method we show that there exist ${\kappa }^+$ -homogeneous, but not ${\kappa }^+$ -transitive permutation groups on 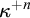${\kappa }^{+n}$ for each infinite cardinal 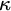${\kappa }$ and natural number $n\ge 1$ provided $V=L$ .