No CrossRef data available.
Article contents
ON CUTS IN ULTRAPRODUCTS OF LINEAR ORDERS II
Published online by Cambridge University Press: 01 May 2018
Abstract
We continue our study of the class  ${\cal C}\left( D \right)$, where D is a uniform ultrafilter on a cardinal κ and
${\cal C}\left( D \right)$, where D is a uniform ultrafilter on a cardinal κ and 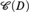 ${\cal C}\left( D \right)$ is the class of all pairs
${\cal C}\left( D \right)$ is the class of all pairs 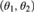 $\left( {{\theta _1},{\theta _2}} \right)$, where
$\left( {{\theta _1},{\theta _2}} \right)$, where 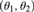 $\left( {{\theta _1},{\theta _2}} \right)$ is the cofinality of a cut in
$\left( {{\theta _1},{\theta _2}} \right)$ is the cofinality of a cut in  ${J^\kappa }/D$ and J is some
${J^\kappa }/D$ and J is some 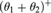 ${\left( {{\theta _1} + {\theta _2}} \right)^ + }$-saturated dense linear order. We give a combinatorial characterization of the class
${\left( {{\theta _1} + {\theta _2}} \right)^ + }$-saturated dense linear order. We give a combinatorial characterization of the class 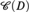 ${\cal C}\left( D \right)$. We also show that if
${\cal C}\left( D \right)$. We also show that if 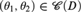 $\left( {{\theta _1},{\theta _2}} \right) \in {\cal C}\left( D \right)$ and D is
$\left( {{\theta _1},{\theta _2}} \right) \in {\cal C}\left( D \right)$ and D is 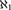 ${\aleph _1}$-complete or
${\aleph _1}$-complete or  ${\theta _1} + {\theta _2} > {2^\kappa }$, then
${\theta _1} + {\theta _2} > {2^\kappa }$, then 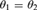 ${\theta _1} = {\theta _2}$.
${\theta _1} = {\theta _2}$.
- Type
- Articles
- Information
- Copyright
- Copyright © The Association for Symbolic Logic 2018


