No CrossRef data available.
Published online by Cambridge University Press: 12 August 2021
We make comments on some problems Erdős and Hajnal posed in their famous problem list. Let X be a graph on  $\omega _1$
with the property that every uncountable set A of vertices contains a finite set s such that each element of
$\omega _1$
with the property that every uncountable set A of vertices contains a finite set s such that each element of  $A-s$
is joined to one of the elements of s. Does then X contain an uncountable clique? (Problem 69) We prove that both the statement and its negation are consistent. Do there exist circuitfree graphs
$A-s$
is joined to one of the elements of s. Does then X contain an uncountable clique? (Problem 69) We prove that both the statement and its negation are consistent. Do there exist circuitfree graphs  $\{X_n:n<\omega \}$
on
$\{X_n:n<\omega \}$
on 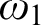 $\omega _1$
such that if
$\omega _1$
such that if 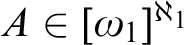 $A\in [\omega _1]^{\aleph _1}$
, then
$A\in [\omega _1]^{\aleph _1}$
, then 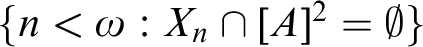 $\{n<\omega :X_n\cap [A]^2=\emptyset \}$
is finite? (Problem 61) We show that the answer is yes under CH, and no under Martin’s axiom. Does there exist
$\{n<\omega :X_n\cap [A]^2=\emptyset \}$
is finite? (Problem 61) We show that the answer is yes under CH, and no under Martin’s axiom. Does there exist 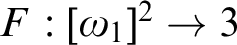 $F:[\omega _1]^2\to 3$
with all three colors appearing in every uncountable set, and with no triangle of three colors. (Problem 68) We give a different proof of Todorcevic’ theorem that the existence of a
$F:[\omega _1]^2\to 3$
with all three colors appearing in every uncountable set, and with no triangle of three colors. (Problem 68) We give a different proof of Todorcevic’ theorem that the existence of a  $\kappa $
-Suslin tree gives
$\kappa $
-Suslin tree gives  $F:[\kappa ]^2\to \kappa $
establishing
$F:[\kappa ]^2\to \kappa $
establishing 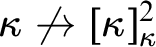 $\kappa \not \to [\kappa ]^2_{\kappa }$
with no three-colored triangles. This statement in turn implies the existence of a
$\kappa \not \to [\kappa ]^2_{\kappa }$
with no three-colored triangles. This statement in turn implies the existence of a  $\kappa $
-Aronszajn tree.
$\kappa $
-Aronszajn tree.