Article contents
A note on a proof of Hilbert's second ε-theorem
Published online by Cambridge University Press: 12 March 2014
Extract
The aim of this short note is to correct an error in the proof in [1], more precisely in Remark 1 on page 395, where it is stated that any improper word has rank ≥2. This claim is false; and, consequently, Remark 2, asserting that any 1,0-deduction does not contain improper Q-terms, also fails immediately. In fact, let us consider the word
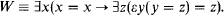
It is easily seen that the rank of W is 1, but W is obviously improper. We remark that it is possible, in the same way, to give examples of improper words of rank 1 containing (without subordination) ε-subterms of arbitrarily high rank. In the following section we sketch a corrected version of the proof in order to eliminate all improper ε-terms.
We refer to the notation and definitions of [1]. A more extended description of the theories involved can be found in [2].
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1987
References
REFERENCES
- 2
- Cited by


