MORE ON YET ANOTHER IDEAL VERSION OF THE BOUNDING NUMBER

Abstract

This is a continuation of the paper [J. Symb. Log. 87 (2022), 1065–1092]. For an ideal $\mathcal {I}$ on 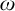$\omega $ we denote $\mathcal {D}_{\mathcal {I}}=\{f\in \omega ^{\omega }: f^{-1}[\{n\}]\in \mathcal {I} \text { for every } n\in \omega \}$ and write 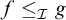$f\leq _{\mathcal {I}} g$ if 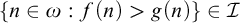$\{n\in \omega :f(n)>g(n)\}\in \mathcal {I}$, where 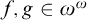$f,g\in \omega ^{\omega }$.

We study the cardinal numbers 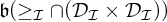$\mathfrak {b}(\geq _{\mathcal {I}}\cap (\mathcal {D}_{\mathcal {I}} \times \mathcal {D}_{\mathcal {I}}))$ describing the smallest sizes of subsets of 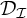$\mathcal {D}_{\mathcal {I}}$ that are unbounded from below with respect to 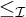$\leq _{\mathcal {I}}$.

In particular, we examine the relationships of 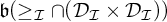$\mathfrak {b}(\geq _{\mathcal {I}}\cap (\mathcal {D}_{\mathcal {I}} \times \mathcal {D}_{\mathcal {I}}))$ with the dominating number 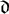$\mathfrak {d}$. We show that, consistently, 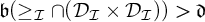$\mathfrak {b}(\geq _{\mathcal {I}}\cap (\mathcal {D}_{\mathcal {I}} \times \mathcal {D}_{\mathcal {I}}))>\mathfrak {d}$ for some ideal 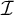$\mathcal {I}$, however 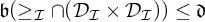$\mathfrak {b}(\geq _{\mathcal {I}}\cap (\mathcal {D}_{\mathcal {I}} \times \mathcal {D}_{\mathcal {I}}))\leq \mathfrak {d}$ for all analytic ideals $\mathcal {I}$. Moreover, we give example of a Borel ideal with 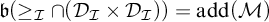$\mathfrak {b}(\geq _{\mathcal {I}}\cap (\mathcal {D}_{\mathcal {I}} \times \mathcal {D}_{\mathcal {I}}))=\operatorname {\mathrm {add}}(\mathcal {M})$.