Hostname: page-component-78c5997874-lj6df Total loading time: 0 Render date: 2024-11-19T20:50:22.241Z Has data issue: false hasContentIssue false
  • English
  • Français

MODAL OPERATORS ON RINGS OF CONTINUOUS FUNCTIONS

Part of: Maps and general types of spaces defined by maps Ordered structures General logic

Published online by Cambridge University Press:  08 October 2021

GURAM BEZHANISHVILI ,
LUCA CARAI  and
PATRICK J. MORANDI Open the ORCID record for PATRICK J. MORANDI [Opens in a new window]
GURAM BEZHANISHVILI
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES NEW MEXICO STATE UNIVERSITYLAS CRUCES, NM 88003, USAE-mail:[email protected]
LUCA CARAI*
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES NEW MEXICO STATE UNIVERSITYLAS CRUCES, NM 88003, USA DIPARTIMENTO DI MATEMATICA UNIVERSITÀ DEGLI STUDI DI SALERNO84084FISCIANO (SA), ITALY
PATRICK J. MORANDI
Affiliation:
DEPARTMENT OF MATHEMATICAL SCIENCES NEW MEXICO STATE UNIVERSITYLAS CRUCES, NM 88003, USAE-mail:[email protected]
*
E-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

It is a classic result in modal logic, often referred to as Jónsson-Tarski duality, that the category of modal algebras is dually equivalent to the category of descriptive frames. The latter are Kripke frames equipped with a Stone topology such that the binary relation is continuous. This duality generalizes the celebrated Stone duality for boolean algebras. Our goal is to generalize descriptive frames so that the topology is an arbitrary compact Hausdorff topology. For this, instead of working with the boolean algebra of clopen subsets of a Stone space, we work with the ring of continuous real-valued functions on a compact Hausdorff space. The main novelty is to define a modal operator on such a ring utilizing a continuous relation on a compact Hausdorff space.

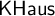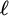Our starting point is the well-known Gelfand duality between the category ${\sf KHaus}$ of compact Hausdorff spaces and the category $\boldsymbol {\mathit {uba}\ell }$ of uniformly complete bounded archimedean $\ell $ -algebras. We endow a bounded archimedean $\ell $ -algebra with a modal operator, which results in the category $\boldsymbol {\mathit {mba}\ell }$ of modal bounded archimedean $\ell $ -algebras. Our main result establishes a dual adjunction between $\boldsymbol {\mathit {mba}\ell }$ and the category 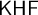${\sf KHF}$ of what we call compact Hausdorff frames; that is, Kripke frames equipped with a compact Hausdorff topology such that the binary relation is continuous. 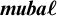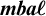This dual adjunction restricts to a dual equivalence between ${\sf KHF}$ and the reflective subcategory $\boldsymbol {\mathit {muba}\ell }$ of $\boldsymbol {\mathit {mba}\ell }$ consisting of uniformly complete objects of $\boldsymbol {\mathit {mba}\ell }$ . This generalizes both Gelfand duality and Jónsson-Tarski duality.

Keywords

Modal algebraKripke framereal-valued functionℓ-algebracompact Hausdorff spacecontinuous relation

MSC classification

Primary: 03B45: Modal logic (including the logic of norms)
Secondary: 54C30: Real-valued functions 06F25: Ordered rings, algebras, modules 06E25: Boolean algebras with additional operations (diagonalizable algebras, etc.) 06E15: Stone spaces (Boolean spaces) and related structures
Type
Article
Information
The Journal of Symbolic Logic , Volume 87 , Issue 4 , December 2022 , pp. 1322 - 1348
Check for updates
Copyright
© The Author(s), 2021. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Banaschewski, B. and Mulvey, C. J., Stone-Čech compactification of locales. I . Houston Journal of Mathematics , vol. 6 (1980), no. 3, pp. 301–312.Google Scholar
Bezhanishvili, G., Stone duality and Gleason covers through de Vries duality . Topology and its Applications , vol. 157 (2010), no. 6, pp. 1064–1080.CrossRefGoogle Scholar
Bezhanishvili, G., De Vries algebras and compact regular frames . Applied Categorical Structures , vol. 20 (2012), no. 6, pp. 569–582.CrossRefGoogle Scholar
Bezhanishvili, G., Bezhanishvili, N., and Harding, J., Modal compact Hausdorff spaces . Journal of Logic and Computation , vol. 25 (2015), no. 1, pp. 1–35.CrossRefGoogle Scholar
Bezhanishvili, G., Bezhanishvili, N., Santoli, T., and Venema, Y., A strict implication calculus for compact Hausdorff spaces . Annals of Pure and Applied Logic , vol. 170 (2019), no. 11, p. 29.CrossRefGoogle Scholar
Bezhanishvili, G., Morandi, P. J., and Olberding, B., Bounded Archimedean  $\ell$ -algebras and Gelfand-Neumark-Stone duality . Theory and Applications of Categories , vol. 28 (2013), pp. 435–475.Google Scholar
Bezhanishvili, G., Morandi, P. J., and Olberding, B., A functional approach to Dedekind completions and the representation of vector lattices and  $\ell$ -algebras by normal functions . Theory and Applications of Categories , vol. 31 (2016), pp. 1095–1133.Google Scholar
Birkhoff, G., Lattice Theory , third ed., American Mathematical Society Colloquium Publications, 25, American Mathematical Society, Providence, RI, 1979.Google Scholar
Blackburn, P., de Rijke, M., and Venema, Y., Modal logic , Cambridge Tracts in Theoretical Computer Science, 53, Cambridge University Press, Cambridge, 2001.CrossRefGoogle Scholar
Casari, E., Comparative logics and abelian $l$ -groups, Logic Colloquium ’88 (Padova, 1988) , Studies in Logic and the Foundation of Mathematics, 127, North-Holland, Amsterdam, 1989, pp. 161–190.Google Scholar
Chagrov, A. and Zakharyaschev, M., Modal logic , Oxford Logic Guides, vol. 35, The Clarendon Press, Oxford University Press, New York, 1997.Google Scholar
de Vries, H., Compact spaces and compactifications. An algebraic approach , Ph.D. thesis, University of Amsterdam, 1962.Google Scholar
Diaconescu, D., Metcalfe, G., and Schnüriger, L., A real-valued modal logic . Logical Methods in Computer Science , vol. 14 (2018), no. 1, p. 27.Google Scholar
Esakia, L. L., Topological Kripke models . Soviet Mathematics Doklady , vol. 15 (1974), pp. 147–151.Google Scholar
Furber, R., Mardare, R., and Mio, M., Probabilistic logics based on Riesz spaces. Logical Methods in Computer Science , vol. 16 (2020), no. 1, p. 45.Google Scholar
Gelfand, I. and Neumark, M., On the imbedding of normed rings into the ring of operators in Hilbert space . Recreational Mathematics [Matematicheskii Sbornik] N.S. , vol. 12 (1943), no. 54, pp. 197–213.Google Scholar
Gillman, L. and Jerison, M., Rings of Continuous Functions , The University Series in Higher Mathematics, D. Van Nostrand Co., Inc., Princeton, N.J.-Toronto-London-New York, 1960.CrossRefGoogle Scholar
Goldblatt, R. I., Metamathematics of modal logic . Reports on Mathematical Logic , vol. 6 (1976), pp. 41–77.Google Scholar
Halmos, P. R., Algebraic logic. I. Monadic Boolean algebras . Compositio Mathematica , vol. 12 (1956), pp. 217–249.Google Scholar
Henriksen, M. and Johnson, D. G., On the structure of a class of Archimedean lattice-ordered algebras . Fundamenta Mathematicae , vol. 50 (1961/1962), pp. 73–94.CrossRefGoogle Scholar
Isbell, J., Atomless parts of spaces . Mathematica Scandinavica , vol. 31 (1972), pp. 5–32.CrossRefGoogle Scholar
Johnson, D. G., A structure theory for a class of lattice-ordered rings . Acta Math. , vol. 104 (1960), pp. 163–215.CrossRefGoogle Scholar
Johnstone, P. T., Stone spaces , Cambridge Studies in Advanced Mathematics, 3, Cambridge University Press, Cambridge, 1982.Google Scholar
Jónsson, B. and Tarski, A., Boolean algebras with operators. I. American Journal of Mathematics , vol. 73 (1951), pp. 891–939.CrossRefGoogle Scholar
Kakutani, S., Weak topology, bicompact set and the principle of duality . Proceedings of the Imperial Academy of Tokyo , vol. 16 (1940), pp. 63–67.Google Scholar
Kakutani, S., Concrete representation of abstract  $(M)$ -spaces. (A characterization of the space of continuous functions) . Annals of Mathematics (2) , vol. 42 (1941), pp. 994–1024.CrossRefGoogle Scholar
Kracht, M., Tools and Techniques in Modal Logic , Studies in Logic and the Foundations of Mathematics, 142, North-Holland Publishing Co., Amsterdam, 1999.Google Scholar
Krein, M. and Krein, S., On an inner characteristic of the set of all continuous functions defined on a bicompact Hausdorff space . Comptes Rendus (Doklady) Academy of Sciences URSS (N.S.) , vol. 27 (1940), pp. 427–430.Google Scholar
Kripke, S. A., Semantical considerations on modal logic , Acta Philosophica Fennica , vol. 16 (1963), pp. 83–94.Google Scholar
Kupke, C., Kurz, A., and Venema, Y., Stone coalgebras . Theoretical Computer Science , vol. 327 (2004), nos. 1–2, pp. 109–134.Google Scholar
Luxemburg, W. A. J. and Zaanen, A. C., Riesz Spaces , vol. 1 , North-Holland Mathematical Library, North-Holland Publishing Co., Amsterdam, 1971.Google Scholar
McGovern, W. W., Neat rings . Journal of Pure and Applied Algebra , vol. 205 (2006), no. 2, pp. 243–265.CrossRefGoogle Scholar
Meyer, R. and Slaney, J., Abelian logic from A to Z , Paraconsistent logic: Essays on the inconsistent , Philosophia Verlag, Berlin, 1989, pp. 245–288.CrossRefGoogle Scholar
Michael, E., Topologies on spaces of subsets . Transactions of the American Mathematical Society , vol. 71 (1951), pp. 152–182.CrossRefGoogle Scholar
Sambin, G. and Vaccaro, V., Topology and duality in modal logic . Annals of Pure and Applied Logic , vol. 37 (1988), no. 3, pp. 249–296.CrossRefGoogle Scholar
Stone, M. H., A general theory of spectra. I . Proceedings of the National Academy of Sciences of the United States of America , vol. 26 (1940), pp. 280–283.CrossRefGoogle ScholarPubMed
Yosida, K., On vector lattice with a unit . Proceedings of the Imperial Academy of Tokyo , vol. 17 (1941), pp. 121–124.Google Scholar