Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-28T15:30:05.766Z Has data issue: false hasContentIssue false
  • English
  • Français

Interpolation and preservation for pebble logics

Published online by Cambridge University Press:  12 March 2014

Alexandru Baltag
Alexandru Baltag*
Affiliation:
CWI, P.O. Bax 94079, 1090 GB Amsterdam, The Netherlands Department of Mathematics, Indiana University, Bloomington, IN 47405, USA E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Extract

In Barwise and van Benthem [6], the authors give a general method for obtaining interpolation and preservation theorems for fragments of L∞ω, those for which there is a co-inductive pebble game Γ characterizing equivalence in the logic. The method is exemplified by an analysis of the following fragments: L∞ω itself, its existential fragment 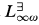, its positive fragment 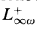, the k-variable fragment (and its existential and positive subfragments) and the modal fragment 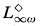(and its existential and positive subfragments).

While most of their method is general, there is one part (showing that Γ has the Scott property relative to the fragment) that required a case-by-case analysis. The purpose of our paper is to replace this case-by-case analysis by a general theorem, and to illustrate this method by obtaining their kinds of results for some additional fragments of L∞ω.

Our general problem can be stated in the following way: Given a “nice” fragment F of L∞ω (one satisfying some natural closure conditions), find a pebble game characterization Γ of “preservation of F-formulas” and prove that Γ has the Scott property with respect to F. Applying the Abstract Interpolation Theorem from [6], we can conclude that F has Γ-interpolation, and the corresponding preservation result. In this paper, we shall give an answer to this question. (Our answer is “sufficient” but we don't know if our conditions are necessary.)

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 64 , Issue 2 , June 1999 , pp. 846 - 858
Copyright
Copyright © Association for Symbolic Logic 1999

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1] Barwise, J., Back and forth through infinitary logic, Studies in model theory (Morley, M., editor), MAA Studies in Mathematics, 1973, pp. 5–35.Google Scholar
[2] Barwise, J., Admissible sets and structures, Springer-Verlag, 1975.CrossRefGoogle Scholar
[3] Barwise, J. and Feferman, S., Model-theoretic logics, Springer-Verlag, 1985.Google Scholar
[4] Barwise, J. and Moschovakis, Y., Global inductive definability, this Journal, vol. 43 (1978), pp. 521–534.Google Scholar
[5] Barwise, J. and Moss, L., Vicious Circles, CSLI Publications, Stanford, 1996.Google Scholar
[6] Barwise, J. and van Benthem, J., Interpolation preservation, and pebble games, this Journal, vol. 64 (1999), pp. 881–903 (this issue).Google Scholar
[7] Ebbinghaus, H-D and Flum, J., Finite model theory, Springer Verlag, 1995.Google Scholar
[8] Karp, C., Finite quantifier equivalence, The theory of models, N. Holland, 1965, pp. 407–412.Google Scholar
[9] Lopez-Escobar, E., An interpolation theorem for denumerably long sentences, Fundamenta Mathematicae, vol. LVII (1965), pp. 253–272.Google Scholar
[10] Malitz, J., Infinitary analogues of theorems from first-order model theory, this Journal, vol. 36 (1971), pp. 216–228.Google Scholar
[11] van Benthem, J., Exploring logical dynamics, Studies in Logic, Language and Information, CSLI Publications, Stanford.Google Scholar