Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-27T12:46:03.183Z Has data issue: false hasContentIssue false
  • English
  • Français

Infinite substructure lattices of models of Peano Arithmetic

Published online by Cambridge University Press:  12 March 2014

James H. Schmerl
James H. Schmerl*
Affiliation:
Department of Mathematics, University of Connecticut, Storrs, CT 06269-3009, USA. E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

Bounded lattices (that is lattices that are both lower bounded and upper bounded) form a large class of lattices that include all distributive lattices, many nondistributive finite lattices such as the pentagon lattice N5. and all lattices in any variety generated by a finite bounded lattice. Extending a theorem of Paris for distributive lattices, we prove that if L is an ℵ0-algebraic bounded lattice, then every countable nonstandard model of Peano Arithmetic has a cofinal elementary extension 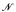such that the interstructure lattice Lt(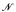/) is isomorphic to L.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 75 , Issue 4 , December 2010 , pp. 1366 - 1382
Copyright
Copyright © Association for Symbolic Logic 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Day, Alan, A simple solution to the word problem for lattices, Canadian Mathematical Bulletin, vol. 13 (1970), pp. 253–254.CrossRefGoogle Scholar
[2]Day, Alan, Characterizations of finite lattices that are bounded-homomorphic images of sublattices of free lattices, Canadian Journal of Mathematics, vol. 31 (1979), pp. 69–78.CrossRefGoogle Scholar
[3]Day, Alan, Nation, J.B., and Tschantz, Steve, Doubling convex sets in lattices and a generalized semidistributivity condition, Order, vol. 6 (1989), pp. 175–180.CrossRefGoogle Scholar
[4]Freese, Ralph, Ježek, Jaroslav, and Nation, J.B., Free lattices, Mathematical Surveys and Monographs, vol. 42, American Mathematical Society, Providence, RI, 1995.CrossRefGoogle Scholar
[5]Kossak, R. and Schmerl, J.H., The structure of models of Peano Arithmetic, Oxford Logic Guides, vol. 50, Oxford University Press, Oxford, 2006.CrossRefGoogle Scholar
[6]Mills, G., Substructure lattices of models of arithmetic, Annals of Mathematical Logic, vol. 16 (1979), pp. 145–180.CrossRefGoogle Scholar
[7]Nation, J.B., e-mail to the author, 06 8, 2009.Google Scholar
[8]Paris, J.B., On models of arithmetic, Conference in Mathematical Logic — London '70 (Bedford College, London, 1970), Lecture Notes in Math., vol. 255, Springer, Berlin, 1972, pp. 251–280.CrossRefGoogle Scholar
[9]Pudlák, P. and Tůma, J., Yeast graphs and fermentation of algebraic lattices, Lattice Theory (Proc. Colloq., Szeged, 1974), Colloq. Math. Soc. Janos Bolyai, vol. 14, North-Holland, Amsterdam, 1976, pp. 301–341.Google Scholar
[10]Pudlák, P., Every finite lattice can be embedded in afinite partition lattice, Algebra Universalis, vol. 10 (1980), pp. 74–95.CrossRefGoogle Scholar
[11]Rolf, H.L., The free lattice generated by a set of chains, Pacific Journal of Mathematics, vol. 8 (1958), pp. 585–595.CrossRefGoogle Scholar
[12]Schmerl, J.H., Finite substructure lattices of models of Peano arithmetic. Proceedings of the American Mathematical Society, vol. 117 (1993), pp. 833–838.CrossRefGoogle Scholar
[13]Semënova, M.V., On lattices that are embeddable into lattices of suborders (russian), Algebra i Logika, vol. 44 (2005), pp. 483–511, (English translation in: Algebra and Logic, vol. 44 (2005), pp. 270–285).Google Scholar
[14]Sivak, B., Representations of finite lattices by orders on finite sets, Mathematica Slovaca, vol. 28 (1978), pp. 203–215.Google Scholar
[15]Wilkie, A.J., On models of arithmetic having non-modular substructure lattices, Fundamenta Mathematicae, vol. 95 (1977), pp. 223–237.CrossRefGoogle Scholar