Hostname: page-component-cd9895bd7-mkpzs Total loading time: 0 Render date: 2024-12-28T10:39:02.206Z Has data issue: false hasContentIssue false
  • English
  • Français

IDENTITY CRISIS BETWEEN SUPERCOMPACTNESS AND VǑPENKA’S PRINCIPLE

Part of: Set theory

Published online by Cambridge University Press:  07 September 2020

YAIR HAYUT ,
MENACHEM MAGIDOR  and
ALEJANDRO POVEDA
YAIR HAYUT
Affiliation:
KURT GÖDEL RESEARCH CENTER INSTITUT FÜR MATHEMATIK UNIVERSITÄT WIEN, 1090 WIEN, AUSTRIAE-mail:[email protected]
MENACHEM MAGIDOR
Affiliation:
INSTITUTE OF MATHEMATICS THE HEBREW UNIVERSITY OF JERUSALEMJERUSALEM91904, ISRAELE-mail:[email protected]
ALEJANDRO POVEDA
Affiliation:
DEPARTAMENT DE MATEMÀTIQUES I INFORMÀTICA UNIVERSITAT DE BARCELONA, GRAN VIA DE LES CORST CATALANES 585, BARCELONA08007, CATALONIAE-mail:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

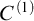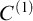In this paper we study the notion of $C^{(n)}$ -supercompactness introduced by Bagaria in [3] and prove the identity crises phenomenon for such class. Specifically, we show that consistently the least supercompact is strictly below the least $C^{(1)}$ -supercompact but also that the least supercompact is $C^{(1)}$ -supercompact (and even 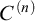$C^{(n)}$ -supercompact). Furthermore, we prove that under suitable hypothesis the ultimate identity crises is also possible. These results solve several questions posed by Bagaria and Tsaprounis.

Keywords

forcingPrikry-type forcinglarge cardinalsC(n)-cardinals

MSC classification

Secondary: 03E50: Continuum hypothesis and Martin's axiom 03E57: Generic absoluteness and forcing axioms
Type
Article
Information
The Journal of Symbolic Logic , Volume 87 , Issue 2 , June 2022 , pp. 626 - 648
Check for updates
Copyright
© The Author(s), 2020. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Apter, A. W., Diamond, square, and level by level equivalence . Archive for Mathematical Logic, vol. 44 (2005), no. 3, pp. 387–395.10.1007/s00153-004-0252-0CrossRefGoogle Scholar
Apter, A. W., The least strongly compact can be the least strong and indestructible . Annals of Pure and Applied Logic, vol. 144 (2006), no. 1, pp. 33–42.10.1016/j.apal.2006.05.002CrossRefGoogle Scholar
Bagaria, J., $C^{(n)}$ -cardinals. Archive for Mathematical Logic, vol. 51 (2012), no. 3, pp. 213–240.10.1007/s00153-011-0261-8CrossRefGoogle Scholar
Bagaria, J., Casacuberta, C., Mathias, A. R. D., and Rosickỳ, J., Definable orthogonality classes in accessible categories are small . Journal of the European Mathematical Society, vol. 17 (2015), no. 3, pp. 549–589.Google Scholar
Bagaria, J., Hamkins, J. D., Tsaprounis, K., and Usuba, T., Superstrong and other large cardinals are never laver indestructible . Archive for Mathematical Logic, vol. 55 (2016), no. 1-2, pp. 19–35.10.1007/s00153-015-0458-3CrossRefGoogle Scholar
Bagaria, J. and Magidor, M., Group radicals and strongly compact cardinals . Transactions of the American Mathematical Society, vol. 366 (2014), no. 4, pp. 1857–1877.CrossRefGoogle Scholar
Bagaria, J. and Magidor, M., On ${\omega}_1$ -strongly compact cardinals, this Journal, vol. 79 (2014), no. 1, pp. 266–278.Google Scholar
Bagaria, J. and Poveda, A., More on the preservation of large cardinals under class forcing. Preprint, 2018, arXiv:1810.09195.Google Scholar
Barbanel, J. B., Diprisco, C. A., and Tan, I. B., Many-times huge and superhuge cardinals , this Journal, vol. 49 (1984), no. 1, pp. 112–122.Google Scholar
Cummings, J., Iterated forcing and elementary embeddings , Handbook of Set Theory, Springer, New York, 2010, pp. 775–883.Google Scholar
Cummings, J., Foreman, M., and Magidor, M., Squares, scales and stationary reflection . Journal of Mathematical Logic, 1 (2001), no. 1, pp. 35–98.10.1142/S021906130100003XCrossRefGoogle Scholar
Gitik, M., Prikry-type forcings , Handbook of Set Theory, Springer, New York, 2010, pp. 1351–1447.CrossRefGoogle Scholar
Kunen, K., Set Theory an Introduction to Independence Proofs, Studies in Logic and the Foundations of Mathematics, vol. 102, Elsevier, Amsterdam, 2014.Google Scholar
Laver, R., Making the supercompactness of $\kappa$  indestructible under  $\kappa$ -directed closed forcing . Israel Journal of Mathematics, vol. 29 (1978), no. 4, pp. 385–388.10.1007/BF02761175CrossRefGoogle Scholar
Poveda, A., Contributions to the theory of Large Cardinals through the method of Forcing . Ph.D. dissertation, Universitat de Barcelona, 2020.Google Scholar
Solovay, R. M., Strongly Compact Cardinals and the GCH , 1974, pp. 365–372.10.1090/pspum/025/0379200CrossRefGoogle Scholar
Tsaprounis, K., Large cardinals and resurrection axioms. 2012.Google Scholar
Tsaprounis, K.. On extendible cardinals and the GCH. Archive for Mathematical Logic, vol. 52 (2013), no. 5-6, pp. 593–602.10.1007/s00153-013-0333-zCrossRefGoogle Scholar
Tsaprounis, K.. Elementary chains and ${C}^{(n)}$ -cardinals. Archive for Mathematical Logic, Vol. 53 (2014), no. 1-2, pp. 89–118.10.1007/s00153-013-0357-4CrossRefGoogle Scholar
Tsaprounis, K., On resurrection axioms, this Journal, vol. 80 (2015), no. 2, pp. 587–608.Google Scholar
Tsaprounis, K., On ${C}^{(n)}$ -extendible cardinals, this Journal, vol. 83 (2018), no. 3, pp. 1112–1131.Google Scholar