Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Schlicht, Philipp
2014.
Thin equivalence relations and inner models.
Annals of Pure and Applied Logic,
Vol. 165,
Issue. 10,
p.
1577.
Motto Ros, Luca
2017.
Can we classify complete metric spaces up to isometry?.
Bollettino dell'Unione Matematica Italiana,
Vol. 10,
Issue. 3,
p.
369.


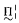 equivalence relation either Borel reduces
equivalence relation either Borel reduces  manner allows the assignment of bounded subsets of ω
manner allows the assignment of bounded subsets of ω