Hostname: page-component-669899f699-7tmb6 Total loading time: 0 Render date: 2025-04-25T06:19:54.565Z Has data issue: false hasContentIssue false
  • English
  • Français

GENERIC EXPANSIONS OF GEOMETRIC THEORIES

Part of: Model theory

Published online by Cambridge University Press:  18 April 2024

SOMAYE JALILI ,
MASSOUD POURMAHDIAN  and
NAZANIN ROSHANDEL TAVANA
SOMAYE JALILI
Affiliation:
DEPARTMENT OF MATHEMATICS AND COMPUTER SCIENCE AMIRKABIR UNIVERSITY OF TECHNOLOGY (TEHRAN POLYTECHNIC) HAFEZ AVENUE 15194, P.O. BOX 15875-4413, TEHRAN, IRAN E-mail: [email protected], [email protected]
MASSOUD POURMAHDIAN*
Affiliation:
DEPARTMENT OF MATHEMATICS AND COMPUTER SCIENCE AMIRKABIR UNIVERSITY OF TECHNOLOGY (TEHRAN POLYTECHNIC) HAFEZ AVENUE 15194, P.O. BOX 15875-4413, TEHRAN, IRAN and SCHOOL OF MATHEMATICS INSTITUTE FOR RESEARCH IN FUNDAMENTAL SCIENCES (IPM) P.O. BOX 19395-5746, TEHRAN, IRAN
NAZANIN ROSHANDEL TAVANA
Affiliation:
DEPARTMENT OF MATHEMATICS AND COMPUTER SCIENCE AMIRKABIR UNIVERSITY OF TECHNOLOGY (TEHRAN POLYTECHNIC) HAFEZ AVENUE 15194, P.O. BOX 15875-4413, TEHRAN, IRAN E-mail: [email protected], [email protected]
*
E-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

As a continuation of ideas initiated in [19], we study bi-colored (generic) expansions of geometric theories in the style of the Fraïssé–Hrushovski construction method. Here we examine that the properties 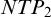$NTP_{2}$, strongness, 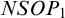$NSOP_{1}$, and simplicity can be transferred to the expansions. As a consequence, while the corresponding bi-colored expansion of a red non-principal ultraproduct of p-adic fields is $NTP_{2}$, the expansion of algebraically closed fields with generic automorphism is a simple theory. Furthermore, these theories are strong with $\operatorname {\mathrm {bdn}}(\text {"}x=x\text {"})=(\aleph _0)_{-}$.

Keywords

geometric theoriesFraïssé–Hrushovski constructionbi-colored expansionsrich structuresNTP2 theoriesNSOP1 theoriessimple theories

MSC classification

Primary: 03C45: Classification theory, stability and related concepts
Type
Article
Information
The Journal of Symbolic Logic , First View , pp. 1 - 22
Check for updates
Copyright
© The Author(s), 2024. Published by Cambridge University Press on behalf of The Association for Symbolic Logic

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Article purchase

Temporarily unavailable

References

Baldwin, J., Recursion theory and abstract dependence . Studies in Logic and the Foundations of Mathematics , vol. 109 (1982), pp. 67–76.CrossRefGoogle Scholar
Baldwin, J. and Holland, K., Constructing $\omega$ -stable structures: Rank 2 fields . Journal of Symbolic Logic , vol. 65 (2000), pp. 371–391.CrossRefGoogle Scholar
Baldwin, J. and Holland, K., Constructing $\omega$ -stable structures: Computing rank . Fundamenta Mathematicae , vol. 170 (2001), pp. 1–20.CrossRefGoogle Scholar
Baldwin, J. and Shi, N., Stable generic structures . Annals of Pure and Applied Logic , vol. 79 (1996), pp. 1–35.CrossRefGoogle Scholar
Baudisch, A., Hils, M., Pizarro, M., and Wagner, F., Die böse farbe . Journal of the Institute of Mathematics of Jussieu , vol. 8 (2009), pp. 415–443.CrossRefGoogle Scholar
Baudisch, A., Pizarro, M., and Ziegler, M., Red fields . Journal of Symbolic Logic , vol. 72 (2007), pp. 207–225.CrossRefGoogle Scholar
Ben-Yaacov, I., Pillay, A., and Vassiliev, E., Lovely pairs of models . Annals of Pure and Applied Logic , vol. 122 (2003), pp. 235–261.CrossRefGoogle Scholar
Ben-Yacov, I. and Chernikov, A., An independence theorem for $NT{P}_2$ theories . Israel Journal of Mathematics , vol. 79 (2014), pp. 135–153.Google Scholar
Berenstein, A., Dolich, A., and Onshuus, A., The independence property in generalized dense pairs of structures . Journal of Symbolic Logic , vol. 76 (2011), pp. 391–404.CrossRefGoogle Scholar
Berenstein, A. and Vassiliev, E., Fields with a dense-codense linearly independent multiplicative subgroup . Archive for Mathematical Logic , vol. 59 (2020), pp. 197–228.CrossRefGoogle Scholar
Chatzidakis, Z. and Pillay, A., Generic structures and simple theories . Annals of Pure and Applied Logic , vol. 95 (1998), pp. 71–92.CrossRefGoogle Scholar
Délbée, C., Generic expansions by a reduct . Journal of Mathematical Logic , vol. 21 (2019), p. 2150016.CrossRefGoogle Scholar
Hrushovski, E. and Pillay, A., Groups definable in local fields and pseudo-finite fields . Israel Journal of Mathematics , vol. 85 (1994), pp. 203–262.CrossRefGoogle Scholar
Jalili, S., Pourmahdian, M., and Khani, M., Bi-Colored expansions of geometric theories, submitted, 2022, arXiv:2204.09142.Google Scholar
Kaplan, I. and Ramsey, N., On Kim-independence . Journal of the European Mathematical Society , vol. 22 (2020), pp. 1423–1474.CrossRefGoogle Scholar
Kim, B. and Pillay, A., Simple theories . Annals of Pure and Applied Logic , vol. 88 (1997), pp. 149–164.CrossRefGoogle Scholar
Kruckman, A. and Ramsey, N., Generic expansion and Skolemization in $NSO{P}_1$ theories . Annals of Pure and Applied Logic , vol. 169 (2018), pp. 755–774.CrossRefGoogle Scholar
Macintyre, A., On definable subsets of p-adic fields . Journal of Symbolic Logic , vol. 41 (1976), pp. 605–610.CrossRefGoogle Scholar
Pas, J., Uniform p-adic cell decomposition and local zeta functions . Journal für die Reine und Angewandte Mathematik , vol. 399 (1989), pp. 137–172.Google Scholar
Poizat, B., Paires de structures stables , Journal of Symbolic Logic , vol. 48 (1983), pp. 234–249.CrossRefGoogle Scholar
Poizat, B., Le carré de l’égalité . Journal of Symbolic Logic , vol. 64 (1999), pp. 1338–1355.Google Scholar
Poizat, B., L’égalité au cube . Journal of Symbolic Logic , vol. 66 (2001), pp. 1647–1676.CrossRefGoogle Scholar
Pourmahdian, M., Simple generic structure . Annals of Pure and Applied Logic , vol. 121 (2003), pp. 227–260.CrossRefGoogle Scholar
Tent, K. and Ziegler, M., A Course in Model Theory , Cambridge University Press, Cambridge, 2012.CrossRefGoogle Scholar