No CrossRef data available.
Article contents
A formal proof of Gödel's theorem
Published online by Cambridge University Press: 12 March 2014
Extract
I. The present paper contains a formal proof of the following theorem of the elementary system ⊨ [ 1 0 ] c:
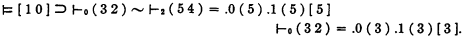
This theorem means that if there were a theorem of ⊢0 ( 3 2 ) 0 stating that there is no contradiction in ⊢2 ( 5 4 ) 2, we would have a contradiction in ⊢2 ( 5 4 ) 2. (This is in accordance with the second theorem of Gödel.)
Our proof is based on the calculus of the system ⊨ [ 1 0 ] c and of the metasystem ⊢0 ( 3 2 ) 0.
Note that we could take
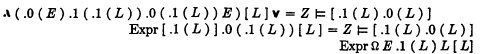
instead of Ax E .1 ( L ) (.1 ( L ) .0 ( L ) L) Z. We would then have to do with the simple systems and metasystems of Hetper, which do not contain propositional variables or the logico-semantical axiom. Our method applies equally to the systems and metasystems of New Foundation and to the simple systems and metasystems of Hetper.
Our proof can be considerably simplified by using a recent result of Hetper concerning ancestral functions. Hetper introduces the following abbreviations:

If A ( Z1c), B ( x1cy1cZ1c) are propositions, the expression 
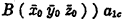 will be called an ancestral function (we prove without difficulty that this expression is a proposition). The expressions
will be called an ancestral function (we prove without difficulty that this expression is a proposition). The expressions 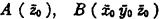 will be called respectively the principal term and the term of derivation of this function.
will be called respectively the principal term and the term of derivation of this function.
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1939
References
1 Cf. Chwistek, L. and Hetper, W., New foundation of formal metamathetmatics, this Journal, vol. 3 (1938), p. 36Google Scholar.
2 Loc. cit., p. 19.
3 Cf. W. Hetper, Simple systems, metasystems, and generalized metasystems (to appear).
4 Cf. Hetper, W., Relacje ancestralne w systemie semantyki, Archiwum Towarzystwa Naukowego we Lwowie, vol. 9, no. 6Google Scholar.
5 Cf. New foundation, p. 35.
6 Cf. New foundation, A11 and p. 35.


