Article contents
Equivalence between semantics for intuitionism. I
Published online by Cambridge University Press: 12 March 2014
Extract
It is probably because intuitionism is founded on the concept of (abstract) proof that it has been possible to develop various kinds of models. The following is but a partial list: Gabbay [5], Beth [2], Kripke [8], Kleene [7], Läuchli [9], McKinsey and Tarski [10], Rasiowa and Sikorski [14], Scott [15], de Swart [16], and Veldman [17].
The original purpose for having the models appears to have been for obtaining independence or consistency results for certain formalizations of intuitionism [see Beth [2], Prawitz [13]]; of course, if the models could be also justified as being plausible interpretations of intuitionistic thinking, so much the better. In fact, having some kind of plausible interpretation makes it much easier to work with the models. Occasionally the models were used to suggest possible extensions of the formal systems; for example, the Kripke models with constant domains have motivated interest in the formal logic CD which extends the Intuitionistic Predicate Calculus (IPC) by having the axiom schema
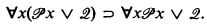
- Type
- Research Article
- Information
- Copyright
- Copyright © Association for Symbolic Logic 1981
References
REFERENCES
- 3
- Cited by


