Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-30T21:18:21.258Z Has data issue: false hasContentIssue false
  • English
  • Français

Dimension theory and homogeneity for elementary extensions of a model

Published online by Cambridge University Press:  12 March 2014

Anand Pillay
Anand Pillay*
Affiliation:
Université Paris VII, Paris, France
Get access Rights & Permissions [Opens in a new window]

Extract

We take a fixed countable model M0, and we look at the structure of and number of its countable elementary extensions (up to isomorphism over M0). Assuming that S(M0) is countable, we prove that if N is a weakly minimal extension of 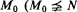, and if 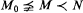then there is an elementary embedding of N into M over M0), then N is homogeneous over M0. Moreover the condition that ∣S(M0)∣ = ℵ0 cannot be removed. Under the hypothesis that M0 contains no infinite set of tuples ordered by a formula, we prove that M0 has infinitely many countable elementary extensions up to isomorphism over M0. A preliminary result is that all types over M0 are definable, and moreover is definable over M0 if and only if 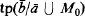is definable over M0 (forking symmetry). We also introduce a notion of relative homogeneity, and show that a large class of elementary extensions of M0 are relatively homogeneous over M0 (under the assumptions that M0 has no order and S(M0) is countable).

I will now discuss the background to and motivation behind the results in this paper, and also the place of this paper relative to other conjectures and investigations. To simplify notation let T denote the complete diagram of M0. First, our result that if M0 has no order then T has infinitely many countable models is related to the following conjecture: any theory with a finite number (more than one) of countable models is unstable.

Type
Research Article
Information
The Journal of Symbolic Logic , Volume 47 , Issue 1 , March 1982 , pp. 147 - 160
Copyright
Copyright © Association for Symbolic Logic 1982

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Lascar, D. and Poizat, B., An introduction to forking, this Journal, vol. 44 (1979), pp. 330–350.Google Scholar
[2]Poizat, B., Deviation des types, Doctoral Thesis, Université Paris VI, 1977.Google Scholar
[3]Pillay, A., Number of countable models, this Journal, vol. 43 (1978), pp. 492–496.Google Scholar
[4]Shelah, S., Classification theory and the number of non-isomorphic models, North-Holland, Amsterdam, 1978.Google Scholar
[5]Vaught, R., Denumerable models of complete theories, Infinitistic methods, Pergamon Press, New York, 1961.Google Scholar
[6]Lascar, D., Ordre de Rudin Keisler et poids dans les théories stables (to appear).Google Scholar