Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Ash, Chris
Knight, Julia
Manasse, Mark
and
Slaman, Theodore
1989.
Generic copies of countable structures.
Annals of Pure and Applied Logic,
Vol. 42,
Issue. 3,
p.
195.
Ash, C. J.
Jockusch, C. G.
and
Knight, J. F.
1990.
Jumps of orderings.
Transactions of the American Mathematical Society,
Vol. 319,
Issue. 2,
p.
573.
Harizanov, Valentina S.
1991.
Uncountable degree spectra.
Annals of Pure and Applied Logic,
Vol. 54,
Issue. 3,
p.
255.
Jockusch, Carl G.
and
Soare, Robert I.
1991.
Degrees of orderings not isomorphic to recursive linear orderings.
Annals of Pure and Applied Logic,
Vol. 52,
Issue. 1-2,
p.
39.
Downey, Rodney
and
Knight, Julia F.
1992.
Orderings with 𝛼th jump degree 0^{(𝛼)}.
Proceedings of the American Mathematical Society,
Vol. 114,
Issue. 2,
p.
545.
Remmel, J. B.
and
Crossley, J. N.
1993.
Logical Methods.
p.
1.
Downey, Rod
and
Jockusch, Carl G.
1994.
Every low Boolean algebra is isomorphic to a recursive one.
Proceedings of the American Mathematical Society,
Vol. 122,
Issue. 3,
p.
871.
Khoussainov, Bakhadyr
and
Shore, Richard A.
1998.
Computable isomorphisms, degree spectra of relations, and Scott families.
Annals of Pure and Applied Logic,
Vol. 93,
Issue. 1-3,
p.
153.
Wehner, Stephan
1998.
Enumerations, countable structures and Turing degrees.
Proceedings of the American Mathematical Society,
Vol. 126,
Issue. 7,
p.
2131.
Kalantari, I.
1998.
Handbook of Recursive Mathematics - Volume 1: Recursive Model Theory.
Vol. 138,
Issue. ,
p.
515.
Knight, J.F.
1998.
Handbook of Recursive Mathematics - Volume 1: Recursive Model Theory.
Vol. 138,
Issue. ,
p.
289.
Downey, R.G.
1998.
Handbook of Recursive Mathematics - Volume 2: Recursive Algebra, Analysis and Combinatorics.
Vol. 139,
Issue. ,
p.
823.
Harizanov, Valentina S.
1998.
Handbook of Recursive Mathematics - Volume 1: Recursive Model Theory.
Vol. 138,
Issue. ,
p.
3.
2000.
Computable Structures and the Hyperarithmetical Hierarchy.
Vol. 144,
Issue. ,
p.
323.
Harizanov, Valentina S.
2002.
Computability-Theoretic Complexity of Countable Structures.
Bulletin of Symbolic Logic,
Vol. 8,
Issue. 4,
p.
457.
Hirschfeldt, Denis R.
Khoussainov, Bakhadyr
Shore, Richard A.
and
Slinko, Arkadii M.
2002.
Degree spectra and computable dimensions in algebraic structures.
Annals of Pure and Applied Logic,
Vol. 115,
Issue. 1-3,
p.
71.
Soskova, Alexandra A.
2005.
New Computational Paradigms.
Vol. 3526,
Issue. ,
p.
451.
Stukachev, Alexey
2006.
Theory and Applications of Models of Computation.
Vol. 3959,
Issue. ,
p.
772.
Soskova, Alexandra A.
2006.
Logical Approaches to Computational Barriers.
Vol. 3988,
Issue. ,
p.
546.
Baleva, V.
2006.
The jump operation for structure degrees.
Archive for Mathematical Logic,
Vol. 45,
Issue. 3,
p.
249.
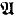 is a structure, then D(
is a structure, then D(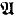 ), the open diagram of
), the open diagram of 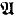 , can be thought of as a subset of ω, and it makes sense to talk about the Turing degree deg(D(
, can be thought of as a subset of ω, and it makes sense to talk about the Turing degree deg(D(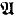 )). This depends on the presentation as well as the isomorphism type of
)). This depends on the presentation as well as the isomorphism type of 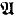 .
.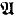 = (ω, <). For any B ⊆ ω, it is possible to code B in a copy of
= (ω, <). For any B ⊆ ω, it is possible to code B in a copy of 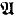 as follows: Let π be the permutation of ω such that for each n ∈ ω, π leaves 2n and 2n + 1 fixed if n ∈ B and switches 2n with 2n + 1 if n ∉ B. Let
as follows: Let π be the permutation of ω such that for each n ∈ ω, π leaves 2n and 2n + 1 fixed if n ∈ B and switches 2n with 2n + 1 if n ∉ B. Let 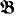 be the copy of
be the copy of 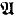 such that
such that 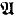 ≃π
≃π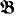 . Then n ∈ B iff the sentence 2n < 2n + 1 is in D(
. Then n ∈ B iff the sentence 2n < 2n + 1 is in D(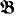 ). In §4, this idea will be used to show that for any structure
). In §4, this idea will be used to show that for any structure 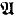 that is not completely trivial, {deg(D(
that is not completely trivial, {deg(D(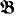 )):
)): 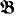 ≃
≃ 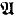 } is closed upwards.
} is closed upwards.

