Hostname: page-component-cd9895bd7-lnqnp Total loading time: 0 Render date: 2024-12-17T18:17:04.425Z Has data issue: false hasContentIssue false
  • English
  • Français

COUNTABLY PERFECTLY MEAGER SETS

Part of: Set theory Spaces with richer structures

Published online by Cambridge University Press:  07 June 2021

ROMAN POL  and
PIOTR ZAKRZEWSKI
ROMAN POL
Affiliation:
INSTITUTE OF MATHEMATICS UNIVERSITY OF WARSAW, UL. BANACHA 2 02-097 WARSAW, POLANDE-mail:[email protected]:[email protected]
PIOTR ZAKRZEWSKI
Affiliation:
INSTITUTE OF MATHEMATICS UNIVERSITY OF WARSAW, UL. BANACHA 2 02-097 WARSAW, POLANDE-mail:[email protected]:[email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We study a strengthening of the notion of a perfectly meager set. We say that a subset A of a perfect Polish space X is countably perfectly meager in X, if for every sequence of perfect subsets 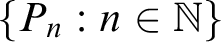$\{P_n: n \in \mathbb N\}$ of X, there exists an 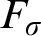$F_\sigma $ -set F in X such that 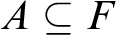$A \subseteq F$ and $F\cap P_n$ is meager in $P_n$ for each n. We give various characterizations and examples of countably perfectly meager sets. We prove that not every universally meager set is countably perfectly meager correcting an earlier result of Bartoszyński.

Keywords

perfectly meager setuniversally meager setperfectly meager set in the transitive senseλ′-setσ-ideal

MSC classification

Primary: 03E20: Other classical set theory (including functions, relations, and set algebra)
Secondary: 03E15: Descriptive set theory 54E52: Baire category, Baire spaces
Type
Article
Information
The Journal of Symbolic Logic , Volume 86 , Issue 3 , September 2021 , pp. 1214 - 1227
Check for updates
Copyright
© Association for Symbolic Logic 2021

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

Bartoszyński, T., On perfectly meager sets . Proceedings of the American Mathematical Society , vol. 130 (2002), no. 4, pp. 1189–1195.CrossRefGoogle Scholar
Bartoszyński, T., Remarks on small sets of reals . Proceedings of the American Mathematical Society , vol. 131 (2003), no. 2, pp. 625–630.CrossRefGoogle Scholar
Bartoszyński, T. and Shelah, S., Continuous images of sets of reals . Topology and its Applications , vol. 116 (2001), pp. 243–253.CrossRefGoogle Scholar
Bennett, H. R., Hosobuchi, M., and Lutzer, D. J., A note on perfect generalized ordered spaces . The Rocky Mountain Journal of Mathematics , vol. 10 (1999), no. 3, pp. 1195–1207.Google Scholar
Engelking, R., General Topology , Sigma Series in Pure Mathematics, vol. 6, Heldermann, Berlin, 1989.Google Scholar
Fremlin, D. H. and Miller, A. W., On some properties of Hurewicz, Menger and Rothberger . Fundamenta Mathematicae , vol. 129 (1988), pp. 17–33.Google Scholar
Galvin, F. and Miller, A. W., γ-sets and other singular sets of real numbers . Topology and its Applications , vol. 17 (1984), pp. 145–155.CrossRefGoogle Scholar
Grzegorek, E., Solution to a problem of Banach on σ-fields without continuous measures . Bulletin of the Polish Academy of Sciences , vol. 28 (1980), pp. 7–10.Google Scholar
Grzegorek, E., Always of the first category sets . Rendiconti del Circolo Matematico di Palermo, Serie II , Supplemento No. 6 (1984), pp. 139–147.Google Scholar
Grzegorek, E., Always of the first category sets. II . Rendiconti del Circolo Matematico di Palermo, Serie II , vol. 10 (1985), pp. 43–48.Google Scholar
Just, W., Miller, A. W., Scheepers, M., and Szeptycki, P. J., The combinatorics of open covers (II) . Topology and its Applications , vol. 73 (1996), pp. 241–266.CrossRefGoogle Scholar
Kechris, A. S., Classical Descriptive Set Theory , Graduate Texts in Mathematics, vol. 156, Springer, New York, 1995.CrossRefGoogle Scholar
Kechris, A. S. and Solecki, S., Approximation of analytic by Borel sets and definable countable chain conditions . Israel Journal of Mathematics , vol. 89 (1995), pp. 343–356.CrossRefGoogle Scholar
Miller, A. W., Special subsets of the real line , Handbook of Set Theoretic Topology (Kunen, K. and Vaughan, J., editors), North-Holland, Amsterdam, 1984, pp. 201–233.CrossRefGoogle Scholar
Nowik, A., Scheepers, M., and Weiss, T., The algebraic sum of sets of real numbers with strong measure zero sets. this Journal, vol. 63 (1998), no. 1, pp. 301–324.Google Scholar
Nowik, A. and Weiss, T., Not every Q-set is perfectly meager in the transitive sense . Proceedings of the American Mathematical Society , vol. 128 (2000), no. 10, pp. 3017–3024.CrossRefGoogle Scholar
Nowik, A. and Weiss, T., On the Ramseyan properties of some special subsets of 2ω and their algebraic sums, this Journal, vol. 67 (2002), no. 2, pp. 547–556.Google Scholar
Pawlikowski, J., Every Sierpiński set is strongly meager . Archive for Mathematical Logic , vol. 35 (1996), pp. 281–285.CrossRefGoogle Scholar
RecŁaw, I., Products of perfectly meagre sets . Proceedings of the American Mathematical Society , vol. 112 (1991), no. 4, pp. 1029–1031.CrossRefGoogle Scholar
RecŁaw, I., Some additive properties of special subsets of reals . Colloquium Mathematicum , vol. 62 (1991), no. 2, pp. 221–226.CrossRefGoogle Scholar
Sierpiński, W., Sur la non-invariance topologique de la propriéte λ’ . Fundamenta Mathematicae , vol. 33 (1945), pp. 264–268.CrossRefGoogle Scholar
Tsaban, B., Menger’s and Hurewicz’s problems: Solutions from “The Book” and refinements . Contemporary Mathematics , vol. 533 (2011), pp. 211–226.CrossRefGoogle Scholar
Weiss, T., On perfectly meager sets in the transitive sense . Proceedings of the American Mathematical Society , vol. 130 (2001), no. 2, pp. 591–594.CrossRefGoogle Scholar
Zakrzewski, P., Universally meager sets . Proceedings of the American Mathematical Society , vol. 129 (2001), no. 6, pp. 1793–1798.CrossRefGoogle Scholar
Zakrzewski, P., Universally meager sets, II . Topology and its Applications , vol. 155 (2008), pp. 1445–1449.CrossRefGoogle Scholar
Zindulka, O., Meager-additive sets in topological groups, preprint, 2018, https://arxiv.org/abs/1806.06674v1.CrossRefGoogle Scholar