No CrossRef data available.
Article contents
COUNTABLE LENGTH EVERYWHERE CLUB UNIFORMIZATION
Part of:
Set theory
Published online by Cambridge University Press: 21 November 2022
Abstract
Assume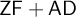 
$\mathsf {ZF} + \mathsf {AD}$ and all sets of reals are Suslin. Let 
$\Gamma $ be a pointclass closed under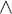 
$\wedge $, 
$\vee $,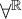 
$\forall ^{\mathbb {R}}$, continuous substitution, and has the scale property. Let 
$\kappa = \delta (\Gamma )$ be the supremum of the length of prewellorderings on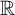 
$\mathbb {R}$ which belong to 
$\Delta = \Gamma \cap \check \Gamma $. Let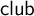 
$\mathsf {club}$ denote the collection of club subsets of 
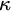$\kappa $. Then the countable length everywhere club uniformization holds for 
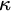$\kappa $: For every relation 
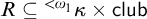$R \subseteq {}^{<{\omega _1}}\kappa \times \mathsf {club}$ with the property that for all 
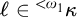$\ell \in {}^{<{\omega _1}}\kappa $ and clubs 
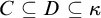$C \subseteq D \subseteq \kappa $, 
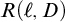$R(\ell ,D)$ implies 
$R(\ell ,C)$, there is a uniformization function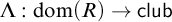 
$\Lambda : \mathrm {dom}(R) \rightarrow \mathsf {club}$ with the property that for all 
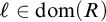$\ell \in \mathrm {dom}(R)$, 
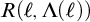$R(\ell ,\Lambda (\ell ))$. In particular, under these assumptions, for all 
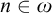$n \in \omega $, 
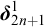$\boldsymbol {\delta }^1_{2n + 1}$ satisfies the countable length everywhere club uniformization.
MSC classification
- Type
- Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of The Association for Symbolic Logic
References
Chan, W., An introduction to combinatorics of determinacy
, Trends in Set Theory (S. Coskey and G. Sargsyan, editors), Contemporary Mathematics, vol. 752, American Mathematical Society, Providence, 2020, pp. 21–75.Google Scholar
Chan, W., Definable combinatorics of strong partition cardinals, in preparation.Google Scholar
Chan, W. and Jackson, S., Definable combinatorics at the first uncountable cardinal
. Transactions of the American Mathematical Society, vol. 374 (2021), no. 3, pp. 2035–2056.Google Scholar
Fuchs, G., A characterization of generalized Příkrý sequences
. Archive for Mathematical Logic, vol. 44 (2005), no. 8, pp. 935–971.Google Scholar
Jackson, S., Structural consequences of AD
, Handbook of Set Theory (M. Foreman and A. Kanamori, editors), Springer, Dordrecht, 2010, pp. 1753–1876.Google Scholar
Kechris, A. S., “AD + UNIFORMIZATION” is equivalent to “Half AD
ℝ”
, Cabal Seminar 81–85 (A. S. Kechris, D. A. Martin, and J. R. Steel, editors), Lecture Notes in Mathematics, vol. 1333, Springer, Berlin, 1988, pp. 98–102.Google Scholar
Kechris, A. S. and Moschovakis, Y. N., Notes on the theory of scales
, Cabal Seminar 76–77 (Proceedings, Caltech-UCLA Logic Seminar 1977–79) (A. S. Kechris and Y. N. Moschovakis, editors), Lecture Notes in Mathematics, vol. 689, Springer, Berlin–New York, 1978, pp. 1–53.Google Scholar
Kechris, A. S. and Woodin, W. H., Generic codes for uncountable ordinals, partition properties, and elementary embeddings
, Games, Scales, and Suslin Cardinals. The Cabal Seminar, Vol. I (A. S. Kechris, B. Löwe, and J. R. Steel, editors), Lecture Notes in Logic, 31, Association for Symbolic Logic, Chicago, 2008, pp. 379–397.Google Scholar
Kechris, A. S., Kleinberg, E. M., Moschovakis, Y. N., and Hugh Woodin, W., The axiom of determinacy, strong partition properties and nonsingular measures
, Cabal Seminar 77–79 (Proceedings, Caltech-UCLA Logic Seminar 1977–79) (A. S. Kechris, D. A. Martin, and Y. N. Moschovakis, editors), Lecture Notes in Mathematics, vol. 839, Springer, Berlin–New York, 1981, pp. 75–99.Google Scholar
Moschovakis, Y. N., Descriptive Set Theory, second ed., Mathematical Surveys and Monographs, vol. 155, American Mathematical Society, Providence, 2009.Google Scholar