Hostname: page-component-78c5997874-8bhkd Total loading time: 0 Render date: 2024-11-15T19:20:14.586Z Has data issue: false hasContentIssue false
  • English
  • Français

The consistency strength of choiceless failures of SCH

Published online by Cambridge University Press:  12 March 2014

Arthur W. Apter  and
Peter Koepke
Arthur W. Apter
Affiliation:
Department of Mathematics, Baruch College of Cuny, New York, New York 10010, USA The Cuny Graduate Center, Mathematics 365 Fifth Avenue, New York, New York 10016, USA. E-mail: [email protected], URL: http://faculty.baruch.cuny.edu/apter
Peter Koepke
Affiliation:
Mathematisches Institut, Rheinische Friedrich-Wilhelms-Universität, Endenicher Allee 60, D-53115 Bonn, Germany. E-mail: [email protected], URL: http://www.math.uni-bonn.de/people/koepke
Get access Rights & Permissions [Opens in a new window]

Abstract

We determine exact consistency strengths for various failures of the Singular Cardinals Hypothesis (SCH) in the setting of the Zermelo-Fraenkel axiom system ZF without the Axiom of Choice (AC). By the new notion of parallel Prikry forcing that we introduce, we obtain surjective failures of SCH using only one measurable cardinal, including a surjective failure of Shelah's pcf theorem about the size of the power set of ℵω. Using symmetric collapses to ℵω, 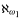, or 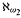, we show that injective failures at ℵω, 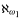, or 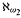can have relatively mild consistency strengths in terms of Mitchell orders of measurable cardinals. Injective failures of both the aforementioned theorem of Shelah and Silver's theorem that GCH cannot first fail at a singular strong limit cardinal of uncountable cofinality are also obtained. Lower bounds are shown by core model techniques and methods due to Gitik and Mitchell.

Keywords

Singular Cardinals Hypothesis (SCH)core modelparallel Prikry forcingsymmetric inner model
Type
Research Article
Information
The Journal of Symbolic Logic , Volume 75 , Issue 3 , September 2010 , pp. 1066 - 1080
Copyright
Copyright © Association for Symbolic Logic 2010

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

[1]Apter, A. and Koepke, P., The consistency strength ℵω and being Rowbottom cardinals without the Axiom of Choice, Archive for Mathematical Logic, vol. 45 (2006), pp. 721–737.CrossRefGoogle Scholar
[2]Devlin, K. and Jensen, R. B., Marginalia to a theorem of Silver, ISILC Logic Conference (Proceedings of the International Summer Institute and Logic Colloquium, Kiel, 1974), Lecture Notes in Mathematics 499, Springer-Verlag, Berlin and New York, 1975, pp. 115–142.Google Scholar
[3]Dodd, A. J., The core model, London Mathematical Society Lecture Note Series #61, Cambridge University Press, Cambridge, 1982.CrossRefGoogle Scholar
[4]Dodd, A. J. and Jensen, R. B., The core model, Annals of Mathematical Logic, vol. 20 (1981), pp. 43–75.CrossRefGoogle Scholar
[5]Easton, W., Powers of regular cardinals, Annals of Mathematical Logic, vol. 1 (1970), pp. 139–178.CrossRefGoogle Scholar
[6]Gitik, M., Dropping cofinalities and gaps, preprint.Google Scholar
[7]Gitik, M., The negation of the Singular Cardinal Hypothesis from ο(κ) = κ++, Annals of Pure and Applied Logic, vol. 43 (1989), pp. 209–234.CrossRefGoogle Scholar
[8]Gitik, M., The strength of the failure of the Singular Cardinal Hypothesis, Annals of Pure and Applied Logic, vol. 51 (1991), pp. 215–240.CrossRefGoogle Scholar
[9]Gitik, M., Blowing up power of a singular cardinal-wider gaps, Annals of Pure and Applied Logic, vol. 116 (2002), pp. 1–38.CrossRefGoogle Scholar
[10]Gitik, M., The power set function, Proceedings of the International Congress of Mathematicians (Beijing 2002), vol. 1, Higher Education Press, Beijing, 2002, pp. 507–513.Google Scholar
[11]Gitik, M., Prikry-type forcings, Handbook of set theory, forthcoming.Google Scholar
[12]Gitik, M. and Mitchell, W., Indiscernible sequences for extenders, and the Singular Cardinal Hypothesis, Annals of Pure and Applied Logic, vol. 82 (1996), pp. 273–316.CrossRefGoogle Scholar
[13]Gitik, M., Schindler, R., and Shelah, S., Pcf theory and Woodin cardinals, Logic Colloquium '02, Lecture Notes in Logic 27, Association for Symbolic Logic, La Jolla, California, 2006, pp. 172–205.Google Scholar
[14]Jech, T., Set theory, Springer-Verlag, Berlin and New York, 2003, The Third Millennium Edition, Revised and Expanded.Google Scholar
[15]Magidor, M., Changing cofinality of cardinals, Fundamenta Mathematicae, vol. 99(1978), pp. 61–71.CrossRefGoogle Scholar
[16]Mcdermott, M., Doctoral Dissertation, Oxford University.Google Scholar
[17]Shelah, S., Cardinal arithmetic, Oxford Logic Guides, vol. 29, Oxford University Press, New York, 1994.CrossRefGoogle Scholar
[18]Silver, J., On the singular cardinals problem, Proceedings of the International Congress of Mathematicians (Vancouver, British Columbia 1974), vol. 1, Canadian Mathematical Congress, Montreal, Quebec, 1975, pp. 265–268.Google Scholar