Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Beyersdorff, Olaf
and
Müller, Sebastian
2008.
Computer Science Logic.
Vol. 5213,
Issue. ,
p.
199.
Beyersdorff, Olaf
and
Sadowski, Zenon
2009.
Computer Science - Theory and Applications.
Vol. 5675,
Issue. ,
p.
47.
Beyersdorff, Olaf
Köbler, Johannes
and
Müller, Sebastian
2009.
Language and Automata Theory and Applications.
Vol. 5457,
Issue. ,
p.
164.
Beyersdorff, Olaf
2009.
On the correspondence between arithmetic theories and propositional proof systems – a survey.
Mathematical Logic Quarterly,
Vol. 55,
Issue. 2,
p.
116.
Beyersdorff, Olaf
2009.
On the Existence of Complete Disjoint NP-Pairs.
p.
282.
Beyersdorff, Olaf
and
Müller, Sebastian
2009.
Theory and Applications of Satisfiability Testing - SAT 2009.
Vol. 5584,
Issue. ,
p.
65.
Hirsch, Edward A.
2010.
Theory and Applications of Models of Computation.
Vol. 6108,
Issue. ,
p.
28.
Beyersdorff, Olaf
and
Müller, Sebastian
2010.
A tight Karp-Lipton collapse result in bounded arithmetic.
ACM Transactions on Computational Logic,
Vol. 11,
Issue. 4,
p.
1.
Beyersdorff, Olaf
and
Müller, Sebastian
2010.
Theory and Applications of Models of Computation.
Vol. 6108,
Issue. ,
p.
50.
Beyersdorff, Olaf
Datta, Samir
Mahajan, Meena
Scharfenberger-Fabian, Gido
Sreenivasaiah, Karteek
Thomas, Michael
and
Vollmer, Heribert
2011.
Mathematical Foundations of Computer Science 2011.
Vol. 6907,
Issue. ,
p.
84.
Beyersdorff, Olaf
and
Sadowski, Zenon
2011.
Do there exist complete sets for promise classes?.
Mathematical Logic Quarterly,
Vol. 57,
Issue. 6,
p.
535.
Beyersdorff, Olaf
Köbler, Johannes
and
Müller, Sebastian
2011.
Proof systems that take advice.
Information and Computation,
Vol. 209,
Issue. 3,
p.
320.
Jain, Sanjay
Stephan, Frank
and
Zeugmann, Thomas
2012.
Theory and Applications of Models of Computation.
Vol. 7287,
Issue. ,
p.
423.
Hirsch, Edward A.
and
Itsykson, Dmitry
2012.
On an optimal randomized acceptor for graph nonisomorphism.
Information Processing Letters,
Vol. 112,
Issue. 5,
p.
166.
Ben Daniel, Sebastian
2012.
Computing and Combinatorics.
Vol. 7434,
Issue. ,
p.
470.
Hirsch, Edward A.
Itsykson, Dmitry
Monakhov, Ivan
and
Smal, Alexander
2012.
On Optimal Heuristic Randomized Semidecision Procedures, with Applications to Proof Complexity and Cryptography.
Theory of Computing Systems,
Vol. 51,
Issue. 2,
p.
179.
Kolokolova, A.
2012.
Expressing versus Proving: Relating Forms of Complexity in Logic.
Journal of Logic and Computation,
Vol. 22,
Issue. 2,
p.
267.
Beyersdorff, Olaf
Datta, Samir
Krebs, Andreas
Mahajan, Meena
Scharfenberger-Fabian, Gido
Sreenivasaiah, Karteek
Thomas, Michael
and
Vollmer, Heribert
2013.
Verifying proofs in constant depth.
ACM Transactions on Computation Theory,
Vol. 5,
Issue. 1,
p.
1.
Krebs, Andreas
Limaye, Nutan
Mahajan, Meena
and
Sreenivasaiah, Karteek
2013.
Mathematical Foundations of Computer Science 2013.
Vol. 8087,
Issue. ,
p.
583.
Pich, Ján
2015.
Circuit lower bounds in bounded arithmetics.
Annals of Pure and Applied Logic,
Vol. 166,
Issue. 1,
p.
29.
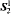 proves NP ⊆ P/poly iff
proves NP ⊆ P/poly iff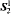 proves coNP ⊆ NP/O(log n). (iv) If
proves coNP ⊆ NP/O(log n). (iv) If 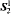 proves NP ⊆ P/poly then
proves NP ⊆ P/poly then 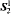 proves that the Polynomial Hierarchy collapses to PNP[log n]. (v) If
proves that the Polynomial Hierarchy collapses to PNP[log n]. (v) If 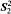 proves NP ⊆ P/poly then
proves NP ⊆ P/poly then 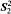 proves that the Polynomial Hierarchy collapses to PNP.
proves that the Polynomial Hierarchy collapses to PNP.

